Two RC coupled amplifiers are connected to form a 2-stage amplier. If the lower and upper cutoff frequencies of each individual amplifier respectively are 100 HZ and 20 kHZ, What these frequencies are for the 2-stage amplifier?a)8.56 kHzb)12.8 kHzc)13.56 kHzd)22.56 kHzCorrect answer is option 'B'. Can you explain this answer?

Question

Answer

Explanation:

To find the cutoff frequencies of the 2-stage amplifier, we need to consider the individual cutoff frequencies of each amplifier and analyze their combined effect.

Individual Amplifier Cutoff Frequencies:

The lower cutoff frequency, fL, of an RC coupled amplifier is given by the formula:
fL = 1 / (2πRC)

Given:
fL1 = 100 Hz
fL2 = 20 kHz

Combined Effect:

When two amplifiers are connected in series, the overall gain and bandwidth are determined by the product of the individual gains and bandwidths.

The overall gain, A, is given by:
A = A1 * A2

The overall bandwidth, B, is given by:
B = B1 * B2

The gain and bandwidth of each amplifier are related to their cutoff frequencies as follows:

A1 = 1 + (f / fL1)
A2 = 1 + (f / fL2)

B1 = fL1 / (A1 - 1)
B2 = fL2 / (A2 - 1)

Calculations:

To find the overall cutoff frequencies, we need to determine the frequencies at which the overall gain and bandwidth decrease by 3 dB (half power).

For the overall gain:
A = 1 + (f / fL1) * 1 + (f / fL2)
0.5A = 1 + (f / fL1) * 1 + (f / fL2)

Solving the above equation, we get:
f = (fL1 * fL2) / (sqrt(A) * (fL2 + fL1))

For the overall bandwidth:
B = fL1 / (A1 - 1) * fL2 / (A2 - 1)
0.5B = fL1 / (A1 - 1) * fL2 / (A2 - 1)

Solving the above equation, we get:
f = (fL1 * fL2) / (sqrt(B) * (fL2 + fL1))

Calculating the Cutoff Frequencies:

Substituting the given values:
fL1 = 100 Hz
fL2 = 20 kHz

For A = 0.5:
f = (100 * 20000) / (sqrt(0.5) * (20000 + 100))
f ≈ 12800 Hz

For B = 0.5:
f = (100 * 20000) / (sqrt(0.5) * (20000 + 100))
f ≈ 12800 Hz

Therefore, the cutoff frequencies of the 2-stage amplifier are approximately 12.8 kHz.

The correct answer is option 'B'.

Answer

For multi-stage amplifier consisting of n identical amplifying stages, the lower cut off f_1(multi)is expressed as,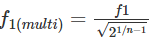

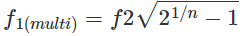
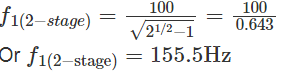
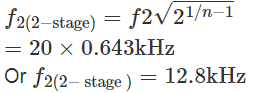
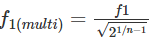
Where, f1 is lower cut-off of individual stages And the upper cutoff

f_{2( multi )} is expressed as,

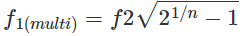
Where f2 is upper cut-off of individual stages Therefore, the lower cut-off of 2-stages amplifier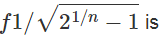

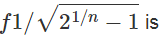
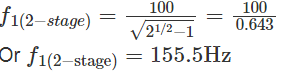
Similarly, the upper cutoff of the 2 -stage amplifier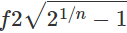

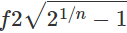is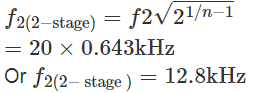

thus the band width gets reduced in a multi-stage amplifier.

Similar Electronics and Communication Engineering (ECE) Doubts

Two RC coupled amplifiers are connected to form a 2-stage amplifier. If the lower and upper cut off frequencies of each individual amplifiers is 100 Hz and 20 kHz. Then the 3-dB bandwidth of the two-state amplifier is ____ Hz. Correct answer is between '12700,12720'. Can you explain this answer?

An amplifier has an open loop gain of 100, and its lower and upper cut- off frequency are 100 Hz and 100 KHz respectively. A feed back network with a feed back factor of 0.99 is connected to the amplifier. The new lower and upper cut off frequencies are at

Common Data Questi on s 29 to 30Q.Two amplifiers A and B are to be connectd in cascade. Find out the order in which the amplifiers must be connected to minimise the noise. Amplifiers A has noise factor of 1.5, gain of 10 dB and amplifier B has noise figure 2 dB, gain of 15 dB.

Which of these is not true for a class B amplifier?

Top Courses for Electronics and Communication Engineering (ECE)View all

GATE ECE (Electronics) Mock Test Series 2025

Network Theory (Electric Circuits)

General Aptitude for GATE

Crash Course: Electronic Communication Engineering (ECE)

Signals and Systems

Top Courses for Electronics and Communication Engineering (ECE)

Top Courses for Electronics and Communication Engineering (ECE)

Suggested Free Tests

Related Content

Mock Test Series

Subject-wise Courses

Subject-wise Courses

Other Engg Branches

Company