CAT Exam > CAT Questions > A can complete a piece of work in 4 days; B t...
Start Learning for Free
A can complete a piece of work in 4 days; B takes double the time taken by A; C takes double the time taken by B; and D takes double the time taken by C. They are paired in groups of two each. The first pair takes two-thirds of the time taken by the second pair to complete the work. Who make up the first pair?
- a)A and B
- b)A and C
- c)B and C
- d)A and D
Correct answer is option 'D'. Can you explain this answer?
Most Upvoted Answer
A can complete a piece of work in 4 days; B takes double the time take...
Amounts of work done in one day by A, B, C and D are 1/4, 1/8, 1/16, and 1/32, respectively.
Using options, we note that the pair of B and C does 3/16 of work in one day.
The pair of A and D does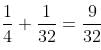 of the work in one day.
of the work in one day.
Hence, A and D take 32/9 days.
Using options, we note that the pair of B and C does 3/16 of work in one day.
The pair of A and D does
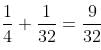 of the work in one day.
of the work in one day.Hence, A and D take 32/9 days.
Free Test
FREE
| Start Free Test |
Community Answer
A can complete a piece of work in 4 days; B takes double the time take...
Given:
A can complete the work in 4 days.
B takes double the time taken by A.
C takes double the time taken by B.
D takes double the time taken by C.
Let's find the time taken by each person to complete the work:
A takes 4 days.
B takes double the time taken by A, so B takes 2 * 4 = 8 days.
C takes double the time taken by B, so C takes 2 * 8 = 16 days.
D takes double the time taken by C, so D takes 2 * 16 = 32 days.
Now, let's consider the pairs of workers:
The first pair takes two-thirds of the time taken by the second pair to complete the work.
Let's assume the first pair completes the work in X days.
So, the second pair will take (2/3)X days to complete the work.
Now, let's calculate the time taken by the first pair:
A takes 4 days, and B takes 8 days. So, together, A and B can complete the work in 4 * 8 / (4 + 8) = 32 / 12 = 8/3 days.
Similarly, let's calculate the time taken by the second pair:
C takes 16 days, and D takes 32 days. So, together, C and D can complete the work in 16 * 32 / (16 + 32) = 512 / 48 = 32/3 days.
According to the given condition, the first pair takes two-thirds of the time taken by the second pair to complete the work. So, X = (2/3) * (32/3) = 64/9 days.
Therefore, the first pair consists of A and D, as they are the only pair that can complete the work in 64/9 days.
Hence, the correct answer is option D) A and D.
A can complete the work in 4 days.
B takes double the time taken by A.
C takes double the time taken by B.
D takes double the time taken by C.
Let's find the time taken by each person to complete the work:
A takes 4 days.
B takes double the time taken by A, so B takes 2 * 4 = 8 days.
C takes double the time taken by B, so C takes 2 * 8 = 16 days.
D takes double the time taken by C, so D takes 2 * 16 = 32 days.
Now, let's consider the pairs of workers:
The first pair takes two-thirds of the time taken by the second pair to complete the work.
Let's assume the first pair completes the work in X days.
So, the second pair will take (2/3)X days to complete the work.
Now, let's calculate the time taken by the first pair:
A takes 4 days, and B takes 8 days. So, together, A and B can complete the work in 4 * 8 / (4 + 8) = 32 / 12 = 8/3 days.
Similarly, let's calculate the time taken by the second pair:
C takes 16 days, and D takes 32 days. So, together, C and D can complete the work in 16 * 32 / (16 + 32) = 512 / 48 = 32/3 days.
According to the given condition, the first pair takes two-thirds of the time taken by the second pair to complete the work. So, X = (2/3) * (32/3) = 64/9 days.
Therefore, the first pair consists of A and D, as they are the only pair that can complete the work in 64/9 days.
Hence, the correct answer is option D) A and D.

|
Explore Courses for CAT exam
|

|
Similar CAT Doubts
A can complete a piece of work in 4 days; B takes double the time taken by A; C takes double the time taken by B; and D takes double the time taken by C. They are paired in groups of two each. The first pair takes two-thirds of the time taken by the second pair to complete the work. Who make up the first pair?a)A and Bb)A and Cc)B and Cd)A and DCorrect answer is option 'D'. Can you explain this answer?
Question Description
A can complete a piece of work in 4 days; B takes double the time taken by A; C takes double the time taken by B; and D takes double the time taken by C. They are paired in groups of two each. The first pair takes two-thirds of the time taken by the second pair to complete the work. Who make up the first pair?a)A and Bb)A and Cc)B and Cd)A and DCorrect answer is option 'D'. Can you explain this answer? for CAT 2025 is part of CAT preparation. The Question and answers have been prepared according to the CAT exam syllabus. Information about A can complete a piece of work in 4 days; B takes double the time taken by A; C takes double the time taken by B; and D takes double the time taken by C. They are paired in groups of two each. The first pair takes two-thirds of the time taken by the second pair to complete the work. Who make up the first pair?a)A and Bb)A and Cc)B and Cd)A and DCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for CAT 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A can complete a piece of work in 4 days; B takes double the time taken by A; C takes double the time taken by B; and D takes double the time taken by C. They are paired in groups of two each. The first pair takes two-thirds of the time taken by the second pair to complete the work. Who make up the first pair?a)A and Bb)A and Cc)B and Cd)A and DCorrect answer is option 'D'. Can you explain this answer?.
A can complete a piece of work in 4 days; B takes double the time taken by A; C takes double the time taken by B; and D takes double the time taken by C. They are paired in groups of two each. The first pair takes two-thirds of the time taken by the second pair to complete the work. Who make up the first pair?a)A and Bb)A and Cc)B and Cd)A and DCorrect answer is option 'D'. Can you explain this answer? for CAT 2025 is part of CAT preparation. The Question and answers have been prepared according to the CAT exam syllabus. Information about A can complete a piece of work in 4 days; B takes double the time taken by A; C takes double the time taken by B; and D takes double the time taken by C. They are paired in groups of two each. The first pair takes two-thirds of the time taken by the second pair to complete the work. Who make up the first pair?a)A and Bb)A and Cc)B and Cd)A and DCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for CAT 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A can complete a piece of work in 4 days; B takes double the time taken by A; C takes double the time taken by B; and D takes double the time taken by C. They are paired in groups of two each. The first pair takes two-thirds of the time taken by the second pair to complete the work. Who make up the first pair?a)A and Bb)A and Cc)B and Cd)A and DCorrect answer is option 'D'. Can you explain this answer?.
Solutions for A can complete a piece of work in 4 days; B takes double the time taken by A; C takes double the time taken by B; and D takes double the time taken by C. They are paired in groups of two each. The first pair takes two-thirds of the time taken by the second pair to complete the work. Who make up the first pair?a)A and Bb)A and Cc)B and Cd)A and DCorrect answer is option 'D'. Can you explain this answer? in English & in Hindi are available as part of our courses for CAT.
Download more important topics, notes, lectures and mock test series for CAT Exam by signing up for free.
Here you can find the meaning of A can complete a piece of work in 4 days; B takes double the time taken by A; C takes double the time taken by B; and D takes double the time taken by C. They are paired in groups of two each. The first pair takes two-thirds of the time taken by the second pair to complete the work. Who make up the first pair?a)A and Bb)A and Cc)B and Cd)A and DCorrect answer is option 'D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A can complete a piece of work in 4 days; B takes double the time taken by A; C takes double the time taken by B; and D takes double the time taken by C. They are paired in groups of two each. The first pair takes two-thirds of the time taken by the second pair to complete the work. Who make up the first pair?a)A and Bb)A and Cc)B and Cd)A and DCorrect answer is option 'D'. Can you explain this answer?, a detailed solution for A can complete a piece of work in 4 days; B takes double the time taken by A; C takes double the time taken by B; and D takes double the time taken by C. They are paired in groups of two each. The first pair takes two-thirds of the time taken by the second pair to complete the work. Who make up the first pair?a)A and Bb)A and Cc)B and Cd)A and DCorrect answer is option 'D'. Can you explain this answer? has been provided alongside types of A can complete a piece of work in 4 days; B takes double the time taken by A; C takes double the time taken by B; and D takes double the time taken by C. They are paired in groups of two each. The first pair takes two-thirds of the time taken by the second pair to complete the work. Who make up the first pair?a)A and Bb)A and Cc)B and Cd)A and DCorrect answer is option 'D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A can complete a piece of work in 4 days; B takes double the time taken by A; C takes double the time taken by B; and D takes double the time taken by C. They are paired in groups of two each. The first pair takes two-thirds of the time taken by the second pair to complete the work. Who make up the first pair?a)A and Bb)A and Cc)B and Cd)A and DCorrect answer is option 'D'. Can you explain this answer? tests, examples and also practice CAT tests.

|
Explore Courses for CAT exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.

























