Electrical Engineering (EE) Exam > Electrical Engineering (EE) Questions > A synchronous generator is connected to an in...
Start Learning for Free
A synchronous generator is connected to an infinite bus with excitation voltage Ef = 1.3 pu. The generator has a synchronous reactance of 1.1 pu and is delivering real power (P) of 0.6 pu to the bus. Assume the infinite bus voltage to be 1.0 pu. Neglect stator resistance. The reactive power (Q) in pu supplied by the generator to the bus under this condition is___________.
Correct answer is '0.109'. Can you explain this answer?
Verified Answer
A synchronous generator is connected to an infinite bus with excitatio...
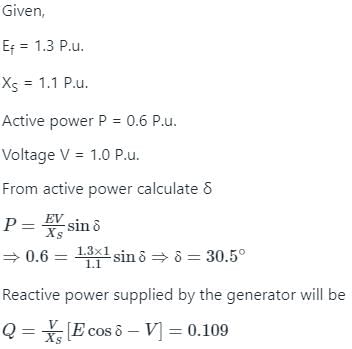
Most Upvoted Answer
A synchronous generator is connected to an infinite bus with excitatio...
Understanding the System
The synchronous generator is connected to an infinite bus, which means it can deliver power without affecting the bus voltage. Here, we are given:
- Excitation voltage (Ef) = 1.3 pu
- Synchronous reactance (Xs) = 1.1 pu
- Real power (P) = 0.6 pu
- Infinite bus voltage (V) = 1.0 pu
Calculating the Internal Voltage (E)
To find the reactive power (Q), we first need to determine the internal voltage E of the generator.
- The relationship between E, V, and the power angle (δ) can be expressed as:
E = V + jQ / (Xs)
In this case, we need to calculate Q before we can find δ.
Using Power Equations
The real power P is given by:
P = V * I * cos(δ)
Where I is the current flowing into the bus.
Next, the equation for reactive power Q is:
Q = V * I * sin(δ)
Combining these equations, we can express Q in terms of known values.
Current Calculation
Using the formula for P:
- I = P / V = 0.6 pu / 1.0 pu = 0.6 pu
Now, we can find the power factor angle (δ) using the relationship:
P = |E| * |V| / |Xs| * sin(δ)
From the equivalent circuit, we can calculate δ and subsequently Q.
Final Calculation of Q
Using the derived equations, we can compute Q:
- Q = (V^2 / Xs) - (P * tan(δ))
Substituting the values leads to:
- Q = 1.0^2 / 1.1 - 0.6 * tan(δ)
Solving this gives:
- Q ≈ 0.109 pu
Conclusion
The reactive power supplied by the generator to the bus under the given conditions is approximately 0.109 pu. This indicates that the generator is supplying a small amount of reactive power to maintain voltage levels at the bus.
The synchronous generator is connected to an infinite bus, which means it can deliver power without affecting the bus voltage. Here, we are given:
- Excitation voltage (Ef) = 1.3 pu
- Synchronous reactance (Xs) = 1.1 pu
- Real power (P) = 0.6 pu
- Infinite bus voltage (V) = 1.0 pu
Calculating the Internal Voltage (E)
To find the reactive power (Q), we first need to determine the internal voltage E of the generator.
- The relationship between E, V, and the power angle (δ) can be expressed as:
E = V + jQ / (Xs)
In this case, we need to calculate Q before we can find δ.
Using Power Equations
The real power P is given by:
P = V * I * cos(δ)
Where I is the current flowing into the bus.
Next, the equation for reactive power Q is:
Q = V * I * sin(δ)
Combining these equations, we can express Q in terms of known values.
Current Calculation
Using the formula for P:
- I = P / V = 0.6 pu / 1.0 pu = 0.6 pu
Now, we can find the power factor angle (δ) using the relationship:
P = |E| * |V| / |Xs| * sin(δ)
From the equivalent circuit, we can calculate δ and subsequently Q.
Final Calculation of Q
Using the derived equations, we can compute Q:
- Q = (V^2 / Xs) - (P * tan(δ))
Substituting the values leads to:
- Q = 1.0^2 / 1.1 - 0.6 * tan(δ)
Solving this gives:
- Q ≈ 0.109 pu
Conclusion
The reactive power supplied by the generator to the bus under the given conditions is approximately 0.109 pu. This indicates that the generator is supplying a small amount of reactive power to maintain voltage levels at the bus.

|
Explore Courses for Electrical Engineering (EE) exam
|

|
Similar Electrical Engineering (EE) Doubts
Question Description
A synchronous generator is connected to an infinite bus with excitation voltage Ef= 1.3 pu. The generator has a synchronous reactance of 1.1 pu and is delivering real power (P) of 0.6 pu to the bus. Assume the infinite bus voltage to be 1.0 pu. Neglect stator resistance. The reactive power (Q) in pu supplied by the generator to the bus under this condition is___________.Correct answer is '0.109'. Can you explain this answer? for Electrical Engineering (EE) 2025 is part of Electrical Engineering (EE) preparation. The Question and answers have been prepared according to the Electrical Engineering (EE) exam syllabus. Information about A synchronous generator is connected to an infinite bus with excitation voltage Ef= 1.3 pu. The generator has a synchronous reactance of 1.1 pu and is delivering real power (P) of 0.6 pu to the bus. Assume the infinite bus voltage to be 1.0 pu. Neglect stator resistance. The reactive power (Q) in pu supplied by the generator to the bus under this condition is___________.Correct answer is '0.109'. Can you explain this answer? covers all topics & solutions for Electrical Engineering (EE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A synchronous generator is connected to an infinite bus with excitation voltage Ef= 1.3 pu. The generator has a synchronous reactance of 1.1 pu and is delivering real power (P) of 0.6 pu to the bus. Assume the infinite bus voltage to be 1.0 pu. Neglect stator resistance. The reactive power (Q) in pu supplied by the generator to the bus under this condition is___________.Correct answer is '0.109'. Can you explain this answer?.
A synchronous generator is connected to an infinite bus with excitation voltage Ef= 1.3 pu. The generator has a synchronous reactance of 1.1 pu and is delivering real power (P) of 0.6 pu to the bus. Assume the infinite bus voltage to be 1.0 pu. Neglect stator resistance. The reactive power (Q) in pu supplied by the generator to the bus under this condition is___________.Correct answer is '0.109'. Can you explain this answer? for Electrical Engineering (EE) 2025 is part of Electrical Engineering (EE) preparation. The Question and answers have been prepared according to the Electrical Engineering (EE) exam syllabus. Information about A synchronous generator is connected to an infinite bus with excitation voltage Ef= 1.3 pu. The generator has a synchronous reactance of 1.1 pu and is delivering real power (P) of 0.6 pu to the bus. Assume the infinite bus voltage to be 1.0 pu. Neglect stator resistance. The reactive power (Q) in pu supplied by the generator to the bus under this condition is___________.Correct answer is '0.109'. Can you explain this answer? covers all topics & solutions for Electrical Engineering (EE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A synchronous generator is connected to an infinite bus with excitation voltage Ef= 1.3 pu. The generator has a synchronous reactance of 1.1 pu and is delivering real power (P) of 0.6 pu to the bus. Assume the infinite bus voltage to be 1.0 pu. Neglect stator resistance. The reactive power (Q) in pu supplied by the generator to the bus under this condition is___________.Correct answer is '0.109'. Can you explain this answer?.
Solutions for A synchronous generator is connected to an infinite bus with excitation voltage Ef= 1.3 pu. The generator has a synchronous reactance of 1.1 pu and is delivering real power (P) of 0.6 pu to the bus. Assume the infinite bus voltage to be 1.0 pu. Neglect stator resistance. The reactive power (Q) in pu supplied by the generator to the bus under this condition is___________.Correct answer is '0.109'. Can you explain this answer? in English & in Hindi are available as part of our courses for Electrical Engineering (EE).
Download more important topics, notes, lectures and mock test series for Electrical Engineering (EE) Exam by signing up for free.
Here you can find the meaning of A synchronous generator is connected to an infinite bus with excitation voltage Ef= 1.3 pu. The generator has a synchronous reactance of 1.1 pu and is delivering real power (P) of 0.6 pu to the bus. Assume the infinite bus voltage to be 1.0 pu. Neglect stator resistance. The reactive power (Q) in pu supplied by the generator to the bus under this condition is___________.Correct answer is '0.109'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A synchronous generator is connected to an infinite bus with excitation voltage Ef= 1.3 pu. The generator has a synchronous reactance of 1.1 pu and is delivering real power (P) of 0.6 pu to the bus. Assume the infinite bus voltage to be 1.0 pu. Neglect stator resistance. The reactive power (Q) in pu supplied by the generator to the bus under this condition is___________.Correct answer is '0.109'. Can you explain this answer?, a detailed solution for A synchronous generator is connected to an infinite bus with excitation voltage Ef= 1.3 pu. The generator has a synchronous reactance of 1.1 pu and is delivering real power (P) of 0.6 pu to the bus. Assume the infinite bus voltage to be 1.0 pu. Neglect stator resistance. The reactive power (Q) in pu supplied by the generator to the bus under this condition is___________.Correct answer is '0.109'. Can you explain this answer? has been provided alongside types of A synchronous generator is connected to an infinite bus with excitation voltage Ef= 1.3 pu. The generator has a synchronous reactance of 1.1 pu and is delivering real power (P) of 0.6 pu to the bus. Assume the infinite bus voltage to be 1.0 pu. Neglect stator resistance. The reactive power (Q) in pu supplied by the generator to the bus under this condition is___________.Correct answer is '0.109'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A synchronous generator is connected to an infinite bus with excitation voltage Ef= 1.3 pu. The generator has a synchronous reactance of 1.1 pu and is delivering real power (P) of 0.6 pu to the bus. Assume the infinite bus voltage to be 1.0 pu. Neglect stator resistance. The reactive power (Q) in pu supplied by the generator to the bus under this condition is___________.Correct answer is '0.109'. Can you explain this answer? tests, examples and also practice Electrical Engineering (EE) tests.

|
Explore Courses for Electrical Engineering (EE) exam
|

|
Signup to solve all Doubts
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.





















