Class 12 Exam > Class 12 Questions > Derive an expression for cyclotron frequency?
Start Learning for Free
Derive an expression for cyclotron frequency?
Most Upvoted Answer
Derive an expression for cyclotron frequency?
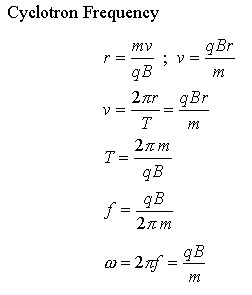
Community Answer
Derive an expression for cyclotron frequency?
Introduction:
The cyclotron frequency is a fundamental concept in physics that describes the rotation of charged particles in a magnetic field. It is an essential parameter in the study of particle accelerators and magnetic resonance imaging (MRI) technology. This frequency can be derived by considering the motion of a charged particle in a uniform magnetic field.
Motion of a Charged Particle:
When a charged particle with charge q and mass m is placed in a uniform magnetic field B, it experiences a magnetic force given by the equation F = qvB, where v is the velocity of the particle. This force acts as a centripetal force that keeps the particle moving in a circular path.
Centripetal Force:
The centripetal force required to keep the particle in a circular path can be given by the equation F = (mv^2)/r, where r is the radius of the circular path. Equating these two forces, we have qvB = (mv^2)/r.
Deriving the Cyclotron Frequency:
By rearranging the equation, we get qB = (mv)/r. Here, we can express the velocity v in terms of the radius r and the angular velocity ω.
Angular Velocity:
The angular velocity ω is defined as the rate at which the particle rotates in radians per unit time. It can be calculated using the equation ω = v/r.
Substituting for v:
Substituting the value of v in terms of ω and r in the previous equation, we get qB = mωr.
Cyclotron Frequency:
The cyclotron frequency, denoted by ωc, is defined as the angular frequency of the circular motion of the charged particle. It is given by the equation ωc = qB/m.
Conclusion:
The cyclotron frequency is derived by considering the motion of a charged particle in a uniform magnetic field. It represents the angular frequency of the circular motion and is given by the equation ωc = qB/m. Understanding the cyclotron frequency is crucial in various fields of physics, such as particle accelerators and MRI technology.
The cyclotron frequency is a fundamental concept in physics that describes the rotation of charged particles in a magnetic field. It is an essential parameter in the study of particle accelerators and magnetic resonance imaging (MRI) technology. This frequency can be derived by considering the motion of a charged particle in a uniform magnetic field.
Motion of a Charged Particle:
When a charged particle with charge q and mass m is placed in a uniform magnetic field B, it experiences a magnetic force given by the equation F = qvB, where v is the velocity of the particle. This force acts as a centripetal force that keeps the particle moving in a circular path.
Centripetal Force:
The centripetal force required to keep the particle in a circular path can be given by the equation F = (mv^2)/r, where r is the radius of the circular path. Equating these two forces, we have qvB = (mv^2)/r.
Deriving the Cyclotron Frequency:
By rearranging the equation, we get qB = (mv)/r. Here, we can express the velocity v in terms of the radius r and the angular velocity ω.
Angular Velocity:
The angular velocity ω is defined as the rate at which the particle rotates in radians per unit time. It can be calculated using the equation ω = v/r.
Substituting for v:
Substituting the value of v in terms of ω and r in the previous equation, we get qB = mωr.
Cyclotron Frequency:
The cyclotron frequency, denoted by ωc, is defined as the angular frequency of the circular motion of the charged particle. It is given by the equation ωc = qB/m.
Conclusion:
The cyclotron frequency is derived by considering the motion of a charged particle in a uniform magnetic field. It represents the angular frequency of the circular motion and is given by the equation ωc = qB/m. Understanding the cyclotron frequency is crucial in various fields of physics, such as particle accelerators and MRI technology.

|
Explore Courses for Class 12 exam
|

|
Similar Class 12 Doubts
Derive an expression for cyclotron frequency?
Question Description
Derive an expression for cyclotron frequency? for Class 12 2025 is part of Class 12 preparation. The Question and answers have been prepared according to the Class 12 exam syllabus. Information about Derive an expression for cyclotron frequency? covers all topics & solutions for Class 12 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Derive an expression for cyclotron frequency?.
Derive an expression for cyclotron frequency? for Class 12 2025 is part of Class 12 preparation. The Question and answers have been prepared according to the Class 12 exam syllabus. Information about Derive an expression for cyclotron frequency? covers all topics & solutions for Class 12 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Derive an expression for cyclotron frequency?.
Solutions for Derive an expression for cyclotron frequency? in English & in Hindi are available as part of our courses for Class 12.
Download more important topics, notes, lectures and mock test series for Class 12 Exam by signing up for free.
Here you can find the meaning of Derive an expression for cyclotron frequency? defined & explained in the simplest way possible. Besides giving the explanation of
Derive an expression for cyclotron frequency?, a detailed solution for Derive an expression for cyclotron frequency? has been provided alongside types of Derive an expression for cyclotron frequency? theory, EduRev gives you an
ample number of questions to practice Derive an expression for cyclotron frequency? tests, examples and also practice Class 12 tests.

|
Explore Courses for Class 12 exam
|

|
Signup to solve all Doubts
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.





















