All Exams >
UPSC >
CSAT Preparation >
All Questions
All questions of Calendars for UPSC CSE Exam
Find the leap year?
- a)700
- b)2000
- c)900
- d)1000
Correct answer is option 'B'. Can you explain this answer?
a)
700
b)
2000
c)
900
d)
1000
|
|
Arun Sharma answered |
Remember the leap year rule:
- Every year divisible by 4 is a leap year, if it is not a century.
- Every 4th century is a leap year, but no other century is a leap year.
- 800,1200 and 2000 comes in the category of 4th century (such as 400,800,1200,1600,2000 etc).
Hence, 800,1200 and 2000 are leap years.
The century can end with
- a)Sunday
- b)Saturday
- c)Thursday
- d)Tuesday
Correct answer is option 'A'. Can you explain this answer?
a)
Sunday
b)
Saturday
c)
Thursday
d)
Tuesday

|
Dhruv Mehra answered |
100 years contain 5 odd days.
Last day of 1st century is Friday.
200 years contain (5 x 2) 3 odd days.
Last day of 2nd century is Wednesday.
300 years contain (5 x 3) = 15 1 odd day.
Last day of 3rd century is Monday.
400 years contain 0 odd day.
Last day of 4th century is Sunday.
This cycle is repeated.
Last day of a century cannot be Tuesday or Thursday or Saturday.
hence,sunday is left only
hence,sunday is left only
what was the day of Aug15 1955?
- a)Sunday
- b)Monday
- c)Tuesday
- d)Friday
Correct answer is option 'B'. Can you explain this answer?
a)
Sunday
b)
Monday
c)
Tuesday
d)
Friday

|
Dhruv Mehra answered |
Date Facts:
August 15, 1955 was a Monday
Zodiac Sign for this date is: Leo
This date was 22,955 days ago
August 15th 2018 is on a Wednesday
Someone born on this date is 62 years old
.
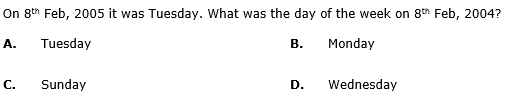
- a)A
- b)B
- c)C
- d)D
Correct answer is option 'C'. Can you explain this answer?
a)
A
b)
B
c)
C
d)
D
|
|
Rohit Jain answered |
The year 2004 is a leap year. It has 2 odd days.
∴ The day on 8th Feb, 2004 is 2 days before the day on 8th Feb, 2005.
Hence, this day is Sunday.
∴ The day on 8th Feb, 2004 is 2 days before the day on 8th Feb, 2005.
Hence, this day is Sunday.
What was the day of the Week on 17th June 1998?
- a)Monday
- b)Tuesday
- c)Wednesday
- d)Thursday
Correct answer is option 'C'. Can you explain this answer?
a)
Monday
b)
Tuesday
c)
Wednesday
d)
Thursday
|
|
Arun Sharma answered |
17th June, 1998 = (1997 years + Period from 1.1.1998 to 17.6.1998)
Odd days in 1600 years = 0
Odd days in 300 years = (5 x 3) ≡ 1
97 years has 24 leap years + 73 ordinary years.
Number of odd days in 97 years ( 24 x 2 + 73) = 121 = 2 odd days.
Jan. Feb. March April May June
(31 + 28 + 31 + 30 + 31 + 17) = 168 days
Therefore 168 days = 24 weeks = 0 odd day.
Total number of odd days = (0 + 1 + 2 + 0) = 3.
Given day is Wednesday.
Odd days in 1600 years = 0
Odd days in 300 years = (5 x 3) ≡ 1
97 years has 24 leap years + 73 ordinary years.
Number of odd days in 97 years ( 24 x 2 + 73) = 121 = 2 odd days.
Jan. Feb. March April May June
(31 + 28 + 31 + 30 + 31 + 17) = 168 days
Therefore 168 days = 24 weeks = 0 odd day.
Total number of odd days = (0 + 1 + 2 + 0) = 3.
Given day is Wednesday.
.
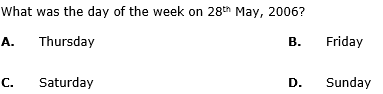
- a)A
- b)B
- c)C
- d)D
Correct answer is option 'D'. Can you explain this answer?
a)
A
b)
B
c)
C
d)
D

|
Aadhar Academy answered |
28 May, 2006 = (2005 years + Period from 1.1.2006 to 28.5.2006)
Odd days in 1600 years = 0
Odd days in 400 years = 0
5 years = (4 ordinary years + 1 leap year) = (4 x 1 + 1 x 2) ≡ 6 odd days
Jan. Feb. March April May
(31 + 28 + 31 + 30 + 28 ) = 148 days
∴ 148 days = (21 weeks + 1 day) ≡ 1 odd day.
Total number of odd days = (0 + 0 + 6 + 1) = 7 ≡ 0 odd day.
Given day is Sunday.
Odd days in 1600 years = 0
Odd days in 400 years = 0
5 years = (4 ordinary years + 1 leap year) = (4 x 1 + 1 x 2) ≡ 6 odd days
Jan. Feb. March April May
(31 + 28 + 31 + 30 + 28 ) = 148 days
∴ 148 days = (21 weeks + 1 day) ≡ 1 odd day.
Total number of odd days = (0 + 0 + 6 + 1) = 7 ≡ 0 odd day.
Given day is Sunday.
.
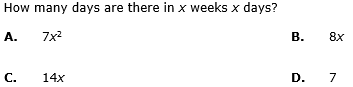
- a)A
- b)B
- c)C
- d)D
Correct answer is option 'B'. Can you explain this answer?
a)
A
b)
B
c)
C
d)
D
|
|
Amit Sharma answered |
x weeks x days = (7x + x) days
= 8x days.
= 8x days.
which calendar year will be same as the year 2008?- a)2018
- b)2020
- c)1980
- d)1960
Correct answer is option 'C'. Can you explain this answer?
which calendar year will be same as the year 2008?
a)
2018
b)
2020
c)
1980
d)
1960
|
|
Kiran Reddy answered |
For every 28 years, the calendars will same,
so the years 2008,2036 have the same calendar as 1980.
so the years 2008,2036 have the same calendar as 1980.
What will be the day of the week 15th August 2010?- a)Sunday
- b)Monday
- c)Tuesday
- d)Friday
Correct answer is option 'A'. Can you explain this answer?
What will be the day of the week 15th August 2010?
a)
Sunday
b)
Monday
c)
Tuesday
d)
Friday

|
Priyanka Menon answered |
**Explanation:**
To determine the day of the week for a given date, we can use the concept of the Gregorian calendar and some basic calculations.
**Step 1: Determining the Reference Day**
- To find the day of the week for a specific date, we need to determine a reference day. In this case, we can choose a known day and its corresponding date that falls within the same year as the given date. Let's choose the reference day as January 1, 2010, which was a Friday.
**Step 2: Counting the Number of Days**
- The next step is to count the number of days between the reference day and the given date. For this, we need to consider both the number of days within the same year and the number of days in the intervening years.
- From January 1, 2010, to August 15, 2010, there are 226 days.
- Additionally, we need to consider the number of days in the intervening years (2011-2019) between the reference year and the given year. There are 9 years, and each year has 365 days, so the total number of intervening days is 9 * 365 = 3285.
- Therefore, the total number of days between the reference day and August 15, 2010, is 226 + 3285 = 3511.
**Step 3: Determining the Day of the Week**
- Now, we need to find the remainder when the total number of days is divided by 7. This remainder will give us the day of the week.
- 3511 divided by 7 equals 501 remainder 4.
- Since the reference day was Friday (which corresponds to 0), we can count 4 days forward to determine the day of the week for August 15, 2010.
- Friday (0) -> Saturday (1) -> Sunday (2) -> Monday (3) -> **Tuesday (4)**.
Therefore, the day of the week for August 15, 2010, was **Tuesday**.
To determine the day of the week for a given date, we can use the concept of the Gregorian calendar and some basic calculations.
**Step 1: Determining the Reference Day**
- To find the day of the week for a specific date, we need to determine a reference day. In this case, we can choose a known day and its corresponding date that falls within the same year as the given date. Let's choose the reference day as January 1, 2010, which was a Friday.
**Step 2: Counting the Number of Days**
- The next step is to count the number of days between the reference day and the given date. For this, we need to consider both the number of days within the same year and the number of days in the intervening years.
- From January 1, 2010, to August 15, 2010, there are 226 days.
- Additionally, we need to consider the number of days in the intervening years (2011-2019) between the reference year and the given year. There are 9 years, and each year has 365 days, so the total number of intervening days is 9 * 365 = 3285.
- Therefore, the total number of days between the reference day and August 15, 2010, is 226 + 3285 = 3511.
**Step 3: Determining the Day of the Week**
- Now, we need to find the remainder when the total number of days is divided by 7. This remainder will give us the day of the week.
- 3511 divided by 7 equals 501 remainder 4.
- Since the reference day was Friday (which corresponds to 0), we can count 4 days forward to determine the day of the week for August 15, 2010.
- Friday (0) -> Saturday (1) -> Sunday (2) -> Monday (3) -> **Tuesday (4)**.
Therefore, the day of the week for August 15, 2010, was **Tuesday**.
16th July 1776,the day of the week was?- a)Wednesday
- b)Tuesday
- c)Saturday
- d)Friday
Correct answer is option 'B'. Can you explain this answer?
16th July 1776,the day of the week was?
a)
Wednesday
b)
Tuesday
c)
Saturday
d)
Friday
|
|
Kiran Reddy answered |
16th July, 1776 = (1775 years + Period from 1st Jan, 1776 to 16th July, 1776)
Counting of odd days :
1600 years have 0 odd day
100 years have 5 odd days
75 years = (18 leap years + 57 ordinary years)
= [(18 x 2) + (57 x 1)]
= 93 (13 weeks + 2 days)
= 2 odd days
1775 years have (0 + 5 + 2) odd days = 7 odd days = 0 odd day
Jan Feb Mar Apr May Jun Jul
31 + 29 + 31 + 30 + 31 + 30 + 16
= 198 days
= (28 weeks + 2 days)
Total number of odd days = (0 + 2) = 2
Required day was 'Tuesday'.
Counting of odd days :
1600 years have 0 odd day
100 years have 5 odd days
75 years = (18 leap years + 57 ordinary years)
= [(18 x 2) + (57 x 1)]
= 93 (13 weeks + 2 days)
= 2 odd days
1775 years have (0 + 5 + 2) odd days = 7 odd days = 0 odd day
Jan Feb Mar Apr May Jun Jul
31 + 29 + 31 + 30 + 31 + 30 + 16
= 198 days
= (28 weeks + 2 days)
Total number of odd days = (0 + 2) = 2
Required day was 'Tuesday'.
On what dates of April 2001 did Wednesday fall?
- a)1st, 8th, 15th, 22nd, 29th
- b)2nd,9th, 16th, 23nd, 30th
- c)3rd, 10th, 17th, 24nd
- d)4st,11th, 18th, 25nd
Correct answer is option 'D'. Can you explain this answer?
a)
1st, 8th, 15th, 22nd, 29th
b)
2nd,9th, 16th, 23nd, 30th
c)
3rd, 10th, 17th, 24nd
d)
4st,11th, 18th, 25nd
|
|
Vikram Kapoor answered |
We shall find the day on 1st April, 2001.
1st April, 2001 = (2000 years + Period from 1.1.2001 to 1.4.2001)
Odd days in 1600 years = 0
Odd days in 400 years = 0
Jan. Feb. March April
(31 + 28 + 31 + 1) = 91 days ≡ 0 odd days.
Total number of odd days = (0 + 0 + 0) = 0
On 1st April, 2001 it was Sunday.
In April, 2001 Wednesday falls on 4th, 11th, 18th and 25th
1st April, 2001 = (2000 years + Period from 1.1.2001 to 1.4.2001)
Odd days in 1600 years = 0
Odd days in 400 years = 0
Jan. Feb. March April
(31 + 28 + 31 + 1) = 91 days ≡ 0 odd days.
Total number of odd days = (0 + 0 + 0) = 0
On 1st April, 2001 it was Sunday.
In April, 2001 Wednesday falls on 4th, 11th, 18th and 25th
On what dates of jan 1st 1992 did friday fall?
- a)1st,8th,15th,22nd,29th
- b)2nd,9th,15th,23nd,30th
- c)3rd,10th,16th,22nd,29th
- d)none of the above
Correct answer is option 'A'. Can you explain this answer?
a)
1st,8th,15th,22nd,29th
b)
2nd,9th,15th,23nd,30th
c)
3rd,10th,16th,22nd,29th
d)
none of the above
|
|
Kajal Sengupta answered |
Each day of the week is repeated after 7 days.
So, after 63 days, it will be Monday.
 After 61 days, it will be Saturday.
After 61 days, it will be Saturday.If 15 March 1816 was Friday, what day of the week would 15th April 1916 be?- a)Monday
- b)Saturday
- c)Thursday
- d)Wednesday
Correct answer is option 'B'. Can you explain this answer?
If 15 March 1816 was Friday, what day of the week would 15th April 1916 be?
a)
Monday
b)
Saturday
c)
Thursday
d)
Wednesday

|
Riverdale Learning Institute answered |
We are given that 15th March 1816 was a Friday.
Now we know that 100 years have 5 odd days. So till 15th March 1916, we will be having 5 odd days.
So if we move from 15th March 1816 to 15th March 1916, we will encounter 5 odd days.
Now from 15th March 1916 to 15th April 1916 there would be 3 odd days.
So total number of odd days = 5 + 3 = 8
8 mod 7 = 1
So 15th April 1916 would be Friday + 1 = Saturday
If 09/12/2001(DD/MM/YYYY) happens to be Sunday, then 09/12/1971 would have been a- a)Wednesday
- b)Thursday
- c)Saturday
- d)Tuesday
Correct answer is option 'B'. Can you explain this answer?
If 09/12/2001(DD/MM/YYYY) happens to be Sunday, then 09/12/1971 would have been a
a)
Wednesday
b)
Thursday
c)
Saturday
d)
Tuesday

|
Upsc Rank Holders answered |
30 years. The number of leap years is 8 (1972,1976,1980,1984,1988,1992,1996,2000).
So, the total number of days = 22*365 + 8*366 = 10958
10958 mod 7 = 3
Since 9/12/2001 is a Sunday, 9/12/1971 should be a Thursday.
If 28th August 1946 was a Wednesday, what day of the week was 31 August 1961?- a)Tuesday
- b)Monday
- c)Thursday
- d)Wednesday
Correct answer is option 'C'. Can you explain this answer?
If 28th August 1946 was a Wednesday, what day of the week was 31 August 1961?
a)
Tuesday
b)
Monday
c)
Thursday
d)
Wednesday

|
S.S Career Academy answered |
It is given that 28th August 1946 was Wednesday.
From 28th August 1946 to 28th August 1961, we have 4 leap years and 11 normal years.
So the number of odd days would be 11*1 + 4*2 = 19
Now the date which is asked is 31 Aug 1961. So if we move from 28th August to 31st August, we will have 3 more odd days.
So total number of odd days = 5 + 3 = 8
Now 8 mod 7 = 1 .
So 31st August 1961 would be Wednesday + 1 = Thursday.
It was Sunday on Jan 1, 2006. What was the day of the Week Jan 1, 2010- a)Sunday
- b)Saturday
- c)Friday
- d)Wednesday
Correct answer is option 'C'. Can you explain this answer?
It was Sunday on Jan 1, 2006. What was the day of the Week Jan 1, 2010
a)
Sunday
b)
Saturday
c)
Friday
d)
Wednesday

|
Rithika Chavan answered |
On 31st December, 2005 it was Saturday.
Number of odd days from the year 2006 to the year 2009 = (1 + 1 + 2 + 1) = 5 days.
∴ On 31st December 2009, it was Thursday.
Thus, on 1st Jan, 2010 it is Friday.
Number of odd days from the year 2006 to the year 2009 = (1 + 1 + 2 + 1) = 5 days.
∴ On 31st December 2009, it was Thursday.
Thus, on 1st Jan, 2010 it is Friday.
A year 1991 is having a same calendar as that of the year X. Which of the following is a possible valueof X.
- a)2002
- b)2000
- c)1902
- d)1903
Correct answer is option 'A'. Can you explain this answer?
A year 1991 is having a same calendar as that of the year X. Which of the following is a possible valueof X.
a)
2002
b)
2000
c)
1902
d)
1903
|
|
Sameer Iyer answered |
Explanation:
A Leap Year in 1895:
- A leap year is a year that is evenly divisible by 4, except for years that are evenly divisible by 100 but not by 400.
- In 1895, the calendar followed the standard leap year rules, so it had 366 days.
Calendar Matching with Year X:
- To find a possible value of X that has the same calendar as 1895, we need to look for a year that is also a leap year.
- The leap years are generally every 4 years, so we need to find a year that is 4 years away from 1895.
Possible Value of X:
- The year that is 4 years after 1895 is 1899 (1895 + 4 = 1899).
- Since 1899 is a leap year (divisible by 4), it will have the same calendar as 1895.
- Therefore, a possible value of X is 1899.
Conclusion:
- Option 'a) 1901' is incorrect because it is not a leap year.
- Option 'b) 1900' is incorrect because it is not 4 years after 1895.
- Option 'c) 1902' is incorrect because it is not a leap year.
- Option 'd) 1903' is incorrect because it is not 4 years after 1895.
- The correct answer is option 'a) 1901' as it is 4 years after 1895 and a leap year.
A Leap Year in 1895:
- A leap year is a year that is evenly divisible by 4, except for years that are evenly divisible by 100 but not by 400.
- In 1895, the calendar followed the standard leap year rules, so it had 366 days.
Calendar Matching with Year X:
- To find a possible value of X that has the same calendar as 1895, we need to look for a year that is also a leap year.
- The leap years are generally every 4 years, so we need to find a year that is 4 years away from 1895.
Possible Value of X:
- The year that is 4 years after 1895 is 1899 (1895 + 4 = 1899).
- Since 1899 is a leap year (divisible by 4), it will have the same calendar as 1895.
- Therefore, a possible value of X is 1899.
Conclusion:
- Option 'a) 1901' is incorrect because it is not a leap year.
- Option 'b) 1900' is incorrect because it is not 4 years after 1895.
- Option 'c) 1902' is incorrect because it is not a leap year.
- Option 'd) 1903' is incorrect because it is not 4 years after 1895.
- The correct answer is option 'a) 1901' as it is 4 years after 1895 and a leap year.
The last day of a Century cannot be- a)Monday
- b)Wednesday
- c)Tuesday
- d)Friday
Correct answer is option 'C'. Can you explain this answer?
The last day of a Century cannot be
a)
Monday
b)
Wednesday
c)
Tuesday
d)
Friday

|
Malavika Rane answered |
100 years contain 5 odd days.
∴ Last day of 1st century is Friday.
200 years contain (5 x 2) ≡ 3 odd days.
∴ Last day of 2nd century is Wednesday.
300 years contain (5 x 3) = 15 ≡ 1 odd day.
∴ Last day of 3rd century is Monday.
400 years contain 0 odd day.
∴ Last day of 4th century is Sunday.
This cycle is repeated.
∴ Last day of a century cannot be Tuesday or Thursday or Saturday.
∴ Last day of 1st century is Friday.
200 years contain (5 x 2) ≡ 3 odd days.
∴ Last day of 2nd century is Wednesday.
300 years contain (5 x 3) = 15 ≡ 1 odd day.
∴ Last day of 3rd century is Monday.
400 years contain 0 odd day.
∴ Last day of 4th century is Sunday.
This cycle is repeated.
∴ Last day of a century cannot be Tuesday or Thursday or Saturday.
In 2016, Mohan celebrated his birthday on Friday. Which will be the first year after 2016 when Mohan will celebrate his birthday on a Wednesday? (He was not born in January or February)- a)2020
- b)2023
- c)2021
- d)2025
Correct answer is option 'A'. Can you explain this answer?
In 2016, Mohan celebrated his birthday on Friday. Which will be the first year after 2016 when Mohan will celebrate his birthday on a Wednesday? (He was not born in January or February)
a)
2020
b)
2023
c)
2021
d)
2025

|
Innovative Classes answered |
Since it has been mentioned that Mohan was not born in February, so he can’t be born on 29th Feb.
Hence He will celebrate his next birthday on a Wednesday in the year for which the sum of the odd days becomes 5 or a multiple of 5.
By his birthday in 2017, there will be 1 odd day.
By his birthday in 2018, there will be 2 odd days.
By his birthday in 2019, there will be 3 odd days.
By his birthday in 2020, there will be 5 odd days, as 2020 is a leap year.
So in 2020 He will celebrate his birthday on Wednesday.
Chapter doubts & questions for Calendars - CSAT Preparation 2025 is part of UPSC CSE exam preparation. The chapters have been prepared according to the UPSC CSE exam syllabus. The Chapter doubts & questions, notes, tests & MCQs are made for UPSC CSE 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests here.
Chapter doubts & questions of Calendars - CSAT Preparation in English & Hindi are available as part of UPSC CSE exam.
Download more important topics, notes, lectures and mock test series for UPSC CSE Exam by signing up for free.

Contact Support
Our team is online on weekdays between 10 AM - 7 PM
Typical reply within 3 hours
|
Free Exam Preparation
at your Fingertips!
Access Free Study Material - Test Series, Structured Courses, Free Videos & Study Notes and Prepare for Your Exam With Ease

 Join the 10M+ students on EduRev
Join the 10M+ students on EduRev
|

|
Create your account for free
OR
Forgot Password
OR
Signup on EduRev and stay on top of your study goals
10M+ students crushing their study goals daily









