All Courses
Get the App Signup / Login
Sign in Open App
All Exams  >   Mechanical Engineering  >   General Aptitude for GATE  >   All Questions

All questions of Series for Mechanical Engineering Exam

Clear all your doubts with EduRev

After striking the floor, a rubber ball rebounds to 4/5th of the height from which it has fallen. Find the total distance that it travels before coming to rest if it has been gently dropped from a height of 120 metres.
  • a)
    540 m
  • b)
    960 m
  • c)
    1080 m
  • d)
    1020 m
  • e)
    1120 m
Correct answer is option 'C'. Can you explain this answer?

Vikas Choudhury answered
The first drop is 120 metres. After this the ball will rise by 96 metres and fall by 96 metres. This process will continue in the form of infinite GP with common ratio 0.8 and first term 96. The required answer is given by the formula:
a/(1-r) 
Now,
[{120/(1/5)}+{96/(1/5)}] 
= 1080 m.

Find the 15th term of the sequence 20, 15, 10....
  • a)
    -45
  • b)
    -55
  • c)
    -50
  • d)
    0
Correct answer is option 'C'. Can you explain this answer?

Santosh Jaiswal answered
Since above sequence is in A.P with common difference of -5 and first term 20.
Then applying formula of AP we get 15 term as
20 + (n-1) d.
15 term is 20 + (15-1) -5 i.e. -50

Four  angles  of  a  quadrilateral  are  in  G.P.  Whose  common  ratio  is  an  intiger.  Two  of  the  angles  are  acute  while  the  other  two  are  obtuse.   The  measure  of  the  smallest   angle  of  the  quadrilateral  is
  • a)
    12
  • b)
    24
  • c)
    36
  • d)
    48
Correct answer is option 'B'. Can you explain this answer?

Kavya Saxena answered
Let   the  angles  be  a, ar, ar 2, ar 3.
Sum  of  the angles = a ( r 4- 1 ) /r -1 = a ( r 2 + 1 ) ( r + 1 ) = 360
a< 90 , and  ar< 90,  Therefore,  a ( 1 + r ) <  180,  or   ( r 2 + 1 ) > 2
Therefore, r  is  not  equal  to  1.  Trying  for  r  =  2  we  get  a  = 24  Therefore, The  angles  are  24, 48, 96  and  192.

Find  the  15th  term  of  an  arithmetic  progression  whose  first  term  is  2  and  the  common  difference  is 3
  • a)
    45
  • b)
    38
  • c)
    44
  • d)
    40
Correct answer is option 'C'. Can you explain this answer?

Aisha Gupta answered
Method to Solve :

A ( first term ) :- 2

d ( common difference ) :- 3

n = 15

To find nth term we have formula as

an = a + ( n - 1 )d

a15 = 2 + 14 � 3

a15 = 2 + 42

a15 = 44

How many terms are there in 20, 25, 30......... 140
  • a)
    22
  • b)
    25
  • c)
    23
  • d)
    24
Correct answer is option 'B'. Can you explain this answer?

Anaya Patel answered
Number of terms = { (1st term - last term) / common difference} + 1
= {(140-20) / 5} + 1
⇒ (120/5) + 1
⇒ 24 + 1 = 25.

Find the first term of an AP whose 8th and 12th terms are respectively 39 and 59.
  • a)
    5
  • b)
    6
  • c)
    4
  • d)
    3
  • e)
    7
Correct answer is 'C'. Can you explain this answer?

Disha Dasgupta answered
Solution: 1st Method:
8th term = a+7d = 39 ........... (i)
12th term = a+11d = 59 ........... (ii)
(i)-(ii);

Or, a+7d-a-11d = 39-59; Or, 4d = 20;
Or, d = 5;
Hence, a+7*5 = 39;
Thus, a = 39-35 = 4.
2nd Method (Thought Process): 
8th term = 39;
And, 12th term = 59;
Here, we see that 20 is added to 8th term 39 to get 12th term 59 i.e. 4 times the common difference is added to 39.
So, CD = 20/4 = 5.
Hence, 7 times CD is added to 1st term to get 39. That means 4 is the 1st term of the AP.

How many terms are there in the AP 20, 25, 30,… 130.
  • a)
    22
  • b)
    23
  • c)
    21
  • d)
    24
Correct answer is option 'B'. Can you explain this answer?

Arnav Rane answered
In order to count the number of terms in the AP, use the short cut:
[(last term – first term)/ common difference] + 1. In this case it would become:
[(130 – 20)/5] +1 = 23. Option (b) is correct.

The sum of the first four terms of an AP is 28 and sum of the first eight terms of the same AP is 88.Find the sum of the first 16 terms of the AP?
  • a)
    346
  • b)
    340
  • c)
    304
  • d)
    268
Correct answer is option 'C'. Can you explain this answer?

Abhay Shah answered
Think like this:
The average of the first 4 terms is 7, while the average of the first 8 terms must be 11.
Now visualize this :
Hence, d = 4/2 = 2 [Note: understand this as a property of an A.P.]
Hence, the average of the 6th and 7th terms = 15 and the average of the 8th and 9th term = 19
But this (19) also represents the average of the 16 term A.P.
Hence, required answer = 16 × 19 = 304.

The sum of 5 numbers in AP is 30 and the sum of their squares is 220. Which of the following is the third term?
  • a)
    5
  • b)
    6
  • c)
    8
  • d)
    9
Correct answer is option 'B'. Can you explain this answer?

Isha Mukherjee answered
Since the sum of 5 numbers in AP is 30, their average would be 6. The average of 5 terms in AP is
also equal to the value of the 3rd term (logic of the middle term of an AP). Hence, the third term’s
value would be 6. Option (b) is correct.

How many terms are there in the GP 5, 20, 80, 320........... 20480?
  • a)
    5
  • b)
    6
  • c)
    8
  • d)
    9
  • e)
    7
Correct answer is option 'E'. Can you explain this answer?

Sameer Rane answered
Common ratio, r = 20/5 = 4;
Last term or nth term of GP = ar^[n-1].
20480 = 5*[4^(n-1)];
Or, 4^(n-1) = 20480/5 = 4^8;
So, comparing the power, 
Thus, n-1 = 8;
Or, n = 7;
Number of terms = 7.

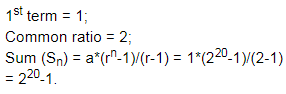
The sum of the first 100 numbers, 1 to 100 is divisible by
  • a)
    1,2,4,8
  • b)
    2 and 4
  • c)
    2
  • d)
    none
Correct answer is option 'C'. Can you explain this answer?

Sagar Sharma answered
The sum of the first 100 numbers, 1 to 100, can be calculated using the formula for the sum of an arithmetic series. The formula is given by:

S = (n/2)(a + l)

where S is the sum of the series, n is the number of terms, a is the first term, and l is the last term.

In this case, we have n = 100, a = 1, and l = 100. Plugging these values into the formula, we get:

S = (100/2)(1 + 100)
S = 50(101)
S = 5050

Therefore, the sum of the first 100 numbers is 5050.

To determine which options this sum is divisible by, we can perform the division:

5050 ÷ 1 = 5050
5050 ÷ 2 = 2525
5050 ÷ 4 = 1262.5
5050 ÷ 8 = 631.25

Therefore, the sum of the first 100 numbers is divisible by 1, 2, and 4.

Now, let's analyze the given options:

a) 1, 2, 4, 8: This option is incorrect because the sum of the first 100 numbers is not divisible by 8.

b) 2 and 4: This option is incorrect because the sum of the first 100 numbers is divisible by 1 and 4, but not just by 2.

c) 2: This option is correct because the sum of the first 100 numbers is divisible by 2.

d) None: This option is incorrect because the sum of the first 100 numbers is divisible by 2.

Therefore, the correct answer is option 'C', which states that the sum of the first 100 numbers is divisible by 2.

 If the roots of x3 - 12x2 + 39x - 28 = 0 are in an AP then their common difference is
  • a)
    ± 1
  • b)
    ± 2
  • c)
    ± 3
  • d)
    ± 4
Correct answer is option 'C'. Can you explain this answer?

Gowri Chakraborty answered
Factorize the equation and we get ( x - 1 ) ( x - 4 ) ( x - 7 )
Explanation:

Given Equation: x³ - 12x² + 39x - 28 = 0

Roots in an AP:
Let the roots of the equation be a - d, a, a + d, where d is the common difference.

Sum of roots: = -b/a
Sum of roots = a - d + a + a + d = 3a

From the equation, we know that sum of roots = -(-12) = 12

Therefore, 3a = 12
a = 4

Product of roots: = -d/a
Product of roots = (a - d)(a)(a + d) = 4(a2 - d2) = 28

Substitute a = 4 in the equation:
42 - d² = 7
16 - d² = 7
d² = 9
d = ±3


Therefore, the correct answer is C: ±3.

If a man saves ` 4 more each year than he did the year before and if he saves ` 20 in the first year,after how many years will his savings be more than ` 1000 altogether?
  • a)
    19 years
  • b)
    20 years
  • c)
    21years
  • d)
    18 years
Correct answer is option 'A'. Can you explain this answer?

Aarav Sharma answered
Solution:

To solve this problem, we can use the formula for the sum of an arithmetic progression:

Sn = n/2[2a + (n-1)d]

where Sn is the sum of the first n terms, a is the first term, d is the common difference, and n is the number of terms.

We know that the man saves 4 more each year than he did the year before, so the common difference is d = 4.

We also know that he saves `20 in the first year, so the first term is a = 20.

We want to find out after how many years his savings will be more than `1000 altogether, so we want to find the value of n that satisfies the inequality Sn > 1000.

Substituting the values that we know into the formula, we get:

Sn = n/2[2a + (n-1)d]
Sn = n/2[2(20) + (n-1)(4)]
Sn = n/2[40 + 4n - 4]
Sn = n/2[36 + 4n]
Sn = 18n + 2n^2

Now we can set up the inequality:

Sn > 1000
18n + 2n^2 > 1000
2n^2 + 18n - 1000 > 0

We can solve this quadratic inequality by factoring or using the quadratic formula, but we can also estimate the value of n by looking at the options given.

Option A is 19 years, which means that the man's savings would be:

S19 = 19/2[2(20) + (19-1)(4)]
S19 = 19/2[40 + 72]
S19 = 19/2[112]
S19 = 1064

This is more than `1000, so option A is the correct answer.

We can also check the other options:

Option B: S20 = 20/2[2(20) + (20-1)(4)] = 1120
Option C: S21 = 21/2[2(20) + (21-1)(4)] = 1204
Option D: S18 = 18/2[2(20) + (18-1)(4)] = 988

Therefore, the correct answer is option A, 19 years.

A sum of money kept in a bank amounts to ` 1240 in 4 years and ` 1600 in 10 years at simpleInterest. Find the sum.
  • a)
    ` 800
  • b)
    ` 900
  • c)
    ` 1150
  • d)
    ` 1000
Correct answer is option 'D'. Can you explain this answer?

Debanshi Sarkar answered
The difference between the amounts at the end of 4 years and 10 years will be the simple interest
on the initial capital for 6 years.
Hence, 360/6 = 60 =(simple interest.)
Also, the Simple Interest for 4 years when added to the sum gives 1240 as the amount.
Hence, the original sum must be 1000.

The sum of the three numbers in A.P is 21 and the product of their extremes is 45. Find the numbers.
  • a)
    9, 7 and 5
  • b)
    5, 7 and 9
  • c)
    Both (1) and (2)
  • d)
    None of these
Correct answer is option 'B'. Can you explain this answer?

Arya Roy answered
The correct answer is c.
Let the numbers are be a - d, a, a + d 
Then a-d+a+a+d= 21 
3a = 21 
a = 7 
and (a - d) (a + d) = 45 
a^2 - d^2 = 45 
d^2=4 
d =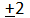 
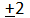
 Hence, the numbers are 5, 7 and 9 when d = 2 and 9, 7 and 5 when d = -2. In both the cases numbers are the same. 

How many terms are there in the GP 5, 20, 80, 320,… 20480?
  • a)
    6
  • b)
    5
  • c)
    7
  • d)
    8
Correct answer is option 'C'. Can you explain this answer?

Saanvi Sarkar answered
The series would be 5, 20, 80, 320, 1280, 5120, 20480. Thus, there are a total of 7 terms in the
series. Option (c) is correct.

Find the lowest number in an AP such that the sum of all the terms is 105 and greatest term is 6 times the least.
  • a)
    5
  • b)
    10
  • c)
    15
  • d)
    (a), (b) & (c)
Correct answer is option 'D'. Can you explain this answer?

Akash Sengupta answered
Trying Option (a),
We get least term 5 and largest term 30 (since the largest term is 6 times the least term).
The average of the A.P becomes (5 + 30)/2 = 17.5
Thus, 17.5 × n = 105 gives us:
to get a total of 105 we need n = 6 i.e. 6 terms in this A.P. That means the A.P. should look like:
5, _ , _ , _, _, 30.
It can be easily seen that the common difference should be 5. The A.P, 5, 10, 15, 20, 25, 30 fits the
situation.
The same process used for option (b) gives us the A.P. 10, 35, 60. (10 + 35 + 60 = 105) and in the
third option 15, 90 (15 + 90 = 105).
Hence, all the three options are correct.

A group of friends have some money which was in an increasing GP. The total money with the first and the last friend was Rs 66 and the product of the money that the second friend had and that the last but one friend had was Rs 128. If the total money with all of them together was Rs 126, then how many friends were there?
  • a)
    6
  • b)
    5
  • c)
    3
  • d)
    Cannot be determined
Correct answer is option 'A'. Can you explain this answer?

Prasenjit Basu answered
The sum of money with the first and the last friend = 66. This can be used as a hint. Let us assume the first friend was having Rs 2 and the last friend was having Rs 64. So, the money can be in the sequence 2, 4, 8, 16, 32, 64. It satisfies the given conditions. Alternatively, this can be done by using the formula for tn of GP also.

The least value of n for which the sum of the series 5 + 8 + 11… n terms is not less than 670 is
  • a)
    20
  • b)
    19
  • c)
    22
  • d)
    21
Correct answer is option 'A'. Can you explain this answer?

Isha Mukherjee answered
Solve this question through trial and error by using values of n from the options:
For 19 terms, the series would be 5 + 8 + 11 + …. + 59 which would give us a sum for the series
as 19 × 32 = 608. The next term (20th term of the series) would be 62. Thus, 608 + 62 = 670
would be the sum to 20 terms. It can thus be concluded that for 20 terms the value of the sum of the
series is not less than 670. Option (a) is correct.

Find the 1st term of an AP whose 8th and 12th terms are respectively 39 and 59.
  • a)
    5
  • b)
    6
  • c)
    4
  • d)
    3
Correct answer is option 'C'. Can you explain this answer?

Isha Mukherjee answered
Since the 8th and the 12th terms of the AP are given as 39 and 59 respectively, the difference
between the two terms would equal 4 times the common difference. Thus we get 4d = 59 – 39 =
20. This gives us d = 5. Also, the 8th term in the AP is represented by a + 7d, we get:
a + 7d = 39 Æ a + 7 × 5 = 39 Æ a = 4. Option (c) is correct.

The sum of three numbers in a GP is 14 and the sum of their squares is 84. Find the largestnumber.
  • a)
    8
  • b)
    6
  • c)
    4
  • d)
    12
Correct answer is option 'A'. Can you explain this answer?

Krithika Sengupta answered
Visualising the squares below 84, we can see that the only way to get the sum of 3 squares as 84
is: 22 + 42 + 82 = 4 + 16 + 64. The largest number is 8. Option (a) is correct.

The sum of the first 16 terms of an AP whose first term and third term are 5 and 15 respectively is
  • a)
    600
  • b)
    765
  • c)
    640
  • d)
    680
  • e)
    690
Correct answer is option 'D'. Can you explain this answer?

Arya Roy answered
1st Method:
1st term = 5;
3rd term = 15;
Then, d = 5;
16th term = a+15d = 5+15*5 = 80;
Sum = {n*(a+L)/2} = {No. of terms*(first term + last term)/2}.
Thus, sum = {16*(5+80)/2} = 680.
2nd Method (Thought Process): 
Sum = Number of terms * Average of that AP.
Sum = 16* {(5+80)/2} = 16*45 = 680.

What  is  the  sum  of  the  first  15  terms  of  an  A.P  whose  11 th  and   7 th  terms  are  5.25  and  3.25  respectively
  • a)
    56.25  
  • b)
    60
  • c)
    52.5
  • d)
    none of these
Correct answer is option 'A'. Can you explain this answer?

Ishani Rane answered
a +10d  = 5.25, a+6d  = 3.25,  4d  =  2,  d  =  1/4
a +5  =  5.25, a  = 0.25  = 1/4,   s 15 =  15/2 ( 2 * 1/4 +  14 * 1/4 )
=  15/2 (1/2 +14/2 )     =  15/2 *15/2  =225/ 4   =   56.25

The 4th and 10th term of an GP are 1/3 and 243 respectively. Find the 2nd term.
  • a)
    3
  • b)
    1
  • c)
    1/27
  • d)
    1/9
Correct answer is option 'C'. Can you explain this answer?

Aarav Sharma answered
Given, 4th term = 1/3 and 10th term = 243.

Let the first term be 'a' and the common ratio be 'r'.

We know that the nth term of a GP is given by ar^(n-1).

So, we can write:

ar^3 = 1/3 ...(1)

ar^9 = 243 ...(2)

Dividing equation (2) by equation (1), we get:

r^6 = 729

r = 3

Substituting this value of r in equation (1), we get:

a(3^3) = 1/3

a = 1/27

Therefore, the first term of the GP is 1/27 and the second term can be found using the formula:

ar = (1/27)*3 = 1/9

Hence, the correct answer is option (c) 1/27.

The sum of the first 16 terms of an AP whose first term and third term are 5 and 15 respectively is
  • a)
    600
  • b)
    765
  • c)
    640
  • d)
    680
Correct answer is option 'D'. Can you explain this answer?

Akash Sengupta answered
a = 5, a + 2d = 15 means d = 5. The 16th term would be a + 15d = 5 + 75 = 80. The sum of the
series would be given by:
[16/2] × [5 + 80] = 16 × 42.5 = 680. Option (d) is correct.

Chapter doubts & questions for Series - General Aptitude for GATE 2025 is part of Mechanical Engineering exam preparation. The chapters have been prepared according to the Mechanical Engineering exam syllabus. The Chapter doubts & questions, notes, tests & MCQs are made for Mechanical Engineering 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests here.

Chapter doubts & questions of Series - General Aptitude for GATE in English & Hindi are available as part of Mechanical Engineering exam. Download more important topics, notes, lectures and mock test series for Mechanical Engineering Exam by signing up for free.

General Aptitude for GATE

194 videos|167 docs|152 tests

Top Courses Mechanical Engineering

Signup to see your scores go up within 7 days!

Study with 1000+ FREE Docs, Videos & Tests
10M+ students study on EduRev
© EduRev
Education Revolution
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup