GMAT Exam > GMAT Questions > Sequence S is defined as Sn = X + (1/X), wher...
Start Learning for Free
Sequence S is defined as Sn = X + (1/X), where X = Sn – 1 + 1, for all n > 1.
If S1= 201, then which of the following must be true of Q, the sum of the first 50 terms of S?
If S1= 201, then which of the following must be true of Q, the sum of the first 50 terms of S?
- a)13,000 < Q < 14,000
- b)12,000 < Q < 13,000
- c)11,000 < Q < 12,000
- d)10,000 < Q < 11,000
- e)9,000 < Q < 10,000
Correct answer is option 'C'. Can you explain this answer?
Verified Answer
Sequence S is defined as Sn = X + (1/X), where X = Sn – 1 + 1, f...
To find each successive term in S, we add 1 to the previous term and add this to the reciprocal of the previous term plus 1.
S1= 201
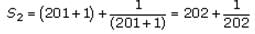
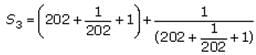
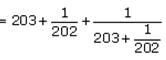
The question asks to estimate (Q), the sum of the first 50 terms of S. If we look at the endpoints of the intervals in the answer choices, we see have quite a bit of leeway as far as our estimation is concerned. In fact, we can simply ignore the fractional portion of each term. Let’s use S2 ≈ 202, S3 ≈ 203. In this way, the sum of the first 50 terms of S will be approximately equal to the sum of the fifty consecutive integers 201, 202 … 250.
To find the sum of the 50 consecutive integers, we can multiply the mean of the integers by the number of integers since average = sum / (number of terms).
The mean of these 50 integers = (201 + 250) / 2 = 225.5
Therefore, the sum of these 50 integers = 50 x 225.5 = 11,275, which falls between 11,000 and 12,000. The correct answer is C.
S1= 201
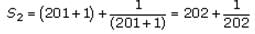
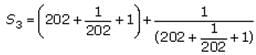
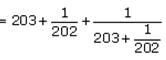
The question asks to estimate (Q), the sum of the first 50 terms of S. If we look at the endpoints of the intervals in the answer choices, we see have quite a bit of leeway as far as our estimation is concerned. In fact, we can simply ignore the fractional portion of each term. Let’s use S2 ≈ 202, S3 ≈ 203. In this way, the sum of the first 50 terms of S will be approximately equal to the sum of the fifty consecutive integers 201, 202 … 250.
To find the sum of the 50 consecutive integers, we can multiply the mean of the integers by the number of integers since average = sum / (number of terms).
The mean of these 50 integers = (201 + 250) / 2 = 225.5
Therefore, the sum of these 50 integers = 50 x 225.5 = 11,275, which falls between 11,000 and 12,000. The correct answer is C.
Most Upvoted Answer
Sequence S is defined as Sn = X + (1/X), where X = Sn – 1 + 1, f...
In this sequence, each term Sn is defined as the reciprocal of the previous term X. The value of X is equal to the previous term Sn.
Let's start with the first term, S1. Since there is no previous term, we can assign any value to it. Let's say S1 = 1.
Now, we can calculate the value of S2 using the formula Sn = X (1/X), where X = Sn.
S2 = X (1/X)
S2 = S1 (1/S1)
S2 = 1 (1/1)
S2 = 1
Similarly, we can calculate the value of S3 using the same formula, but with X = S2.
S3 = X (1/X)
S3 = S2 (1/S2)
S3 = 1 (1/1)
S3 = 1
Continuing this pattern, we can see that all the terms in the sequence will be equal to 1. So, the sequence S is a constant sequence with all terms equal to 1.
Let's start with the first term, S1. Since there is no previous term, we can assign any value to it. Let's say S1 = 1.
Now, we can calculate the value of S2 using the formula Sn = X (1/X), where X = Sn.
S2 = X (1/X)
S2 = S1 (1/S1)
S2 = 1 (1/1)
S2 = 1
Similarly, we can calculate the value of S3 using the same formula, but with X = S2.
S3 = X (1/X)
S3 = S2 (1/S2)
S3 = 1 (1/1)
S3 = 1
Continuing this pattern, we can see that all the terms in the sequence will be equal to 1. So, the sequence S is a constant sequence with all terms equal to 1.

|
Explore Courses for GMAT exam
|

|
Similar GMAT Doubts
Sequence S is defined as Sn = X + (1/X), where X = Sn – 1 + 1, for all n > 1.If S1= 201, then which of the following must be true of Q, the sum of the first 50 terms of S?a)13,000 < Q < 14,000b)12,000 < Q < 13,000c)11,000 < Q < 12,000d)10,000 < Q < 11,000e)9,000 < Q < 10,000Correct answer is option 'C'. Can you explain this answer?
Question Description
Sequence S is defined as Sn = X + (1/X), where X = Sn – 1 + 1, for all n > 1.If S1= 201, then which of the following must be true of Q, the sum of the first 50 terms of S?a)13,000 < Q < 14,000b)12,000 < Q < 13,000c)11,000 < Q < 12,000d)10,000 < Q < 11,000e)9,000 < Q < 10,000Correct answer is option 'C'. Can you explain this answer? for GMAT 2025 is part of GMAT preparation. The Question and answers have been prepared according to the GMAT exam syllabus. Information about Sequence S is defined as Sn = X + (1/X), where X = Sn – 1 + 1, for all n > 1.If S1= 201, then which of the following must be true of Q, the sum of the first 50 terms of S?a)13,000 < Q < 14,000b)12,000 < Q < 13,000c)11,000 < Q < 12,000d)10,000 < Q < 11,000e)9,000 < Q < 10,000Correct answer is option 'C'. Can you explain this answer? covers all topics & solutions for GMAT 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Sequence S is defined as Sn = X + (1/X), where X = Sn – 1 + 1, for all n > 1.If S1= 201, then which of the following must be true of Q, the sum of the first 50 terms of S?a)13,000 < Q < 14,000b)12,000 < Q < 13,000c)11,000 < Q < 12,000d)10,000 < Q < 11,000e)9,000 < Q < 10,000Correct answer is option 'C'. Can you explain this answer?.
Sequence S is defined as Sn = X + (1/X), where X = Sn – 1 + 1, for all n > 1.If S1= 201, then which of the following must be true of Q, the sum of the first 50 terms of S?a)13,000 < Q < 14,000b)12,000 < Q < 13,000c)11,000 < Q < 12,000d)10,000 < Q < 11,000e)9,000 < Q < 10,000Correct answer is option 'C'. Can you explain this answer? for GMAT 2025 is part of GMAT preparation. The Question and answers have been prepared according to the GMAT exam syllabus. Information about Sequence S is defined as Sn = X + (1/X), where X = Sn – 1 + 1, for all n > 1.If S1= 201, then which of the following must be true of Q, the sum of the first 50 terms of S?a)13,000 < Q < 14,000b)12,000 < Q < 13,000c)11,000 < Q < 12,000d)10,000 < Q < 11,000e)9,000 < Q < 10,000Correct answer is option 'C'. Can you explain this answer? covers all topics & solutions for GMAT 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Sequence S is defined as Sn = X + (1/X), where X = Sn – 1 + 1, for all n > 1.If S1= 201, then which of the following must be true of Q, the sum of the first 50 terms of S?a)13,000 < Q < 14,000b)12,000 < Q < 13,000c)11,000 < Q < 12,000d)10,000 < Q < 11,000e)9,000 < Q < 10,000Correct answer is option 'C'. Can you explain this answer?.
Solutions for Sequence S is defined as Sn = X + (1/X), where X = Sn – 1 + 1, for all n > 1.If S1= 201, then which of the following must be true of Q, the sum of the first 50 terms of S?a)13,000 < Q < 14,000b)12,000 < Q < 13,000c)11,000 < Q < 12,000d)10,000 < Q < 11,000e)9,000 < Q < 10,000Correct answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for GMAT.
Download more important topics, notes, lectures and mock test series for GMAT Exam by signing up for free.
Here you can find the meaning of Sequence S is defined as Sn = X + (1/X), where X = Sn – 1 + 1, for all n > 1.If S1= 201, then which of the following must be true of Q, the sum of the first 50 terms of S?a)13,000 < Q < 14,000b)12,000 < Q < 13,000c)11,000 < Q < 12,000d)10,000 < Q < 11,000e)9,000 < Q < 10,000Correct answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Sequence S is defined as Sn = X + (1/X), where X = Sn – 1 + 1, for all n > 1.If S1= 201, then which of the following must be true of Q, the sum of the first 50 terms of S?a)13,000 < Q < 14,000b)12,000 < Q < 13,000c)11,000 < Q < 12,000d)10,000 < Q < 11,000e)9,000 < Q < 10,000Correct answer is option 'C'. Can you explain this answer?, a detailed solution for Sequence S is defined as Sn = X + (1/X), where X = Sn – 1 + 1, for all n > 1.If S1= 201, then which of the following must be true of Q, the sum of the first 50 terms of S?a)13,000 < Q < 14,000b)12,000 < Q < 13,000c)11,000 < Q < 12,000d)10,000 < Q < 11,000e)9,000 < Q < 10,000Correct answer is option 'C'. Can you explain this answer? has been provided alongside types of Sequence S is defined as Sn = X + (1/X), where X = Sn – 1 + 1, for all n > 1.If S1= 201, then which of the following must be true of Q, the sum of the first 50 terms of S?a)13,000 < Q < 14,000b)12,000 < Q < 13,000c)11,000 < Q < 12,000d)10,000 < Q < 11,000e)9,000 < Q < 10,000Correct answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Sequence S is defined as Sn = X + (1/X), where X = Sn – 1 + 1, for all n > 1.If S1= 201, then which of the following must be true of Q, the sum of the first 50 terms of S?a)13,000 < Q < 14,000b)12,000 < Q < 13,000c)11,000 < Q < 12,000d)10,000 < Q < 11,000e)9,000 < Q < 10,000Correct answer is option 'C'. Can you explain this answer? tests, examples and also practice GMAT tests.

|
Explore Courses for GMAT exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


























