Physics Exam > Physics Questions > In a double slit interference experiment d is...
Start Learning for Free
In a double slit interference experiment d is the distance between the centres of the slits and w is the width of each slit. Find the minimum integer ratio d/ω for which an interference maximum of incident plane waves on a distant screen will be missing.
Correct answer is '2'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
In a double slit interference experiment d is the distance between the...
We need a minimum of the single slit diffraction to coincide with a maximum of the double slit interference pattern (which result in a missing interference maximum)
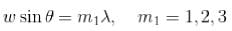
[angulars location of minima of single slit diffraction]
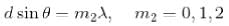
[Angular locations of the maxima of double slit interference]
Ratio of 2 equations gives
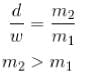
So, minimum integer ratio is 2/1
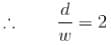
The correct answer is: 2
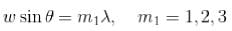
[angulars location of minima of single slit diffraction]
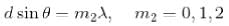
[Angular locations of the maxima of double slit interference]
Ratio of 2 equations gives
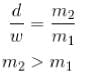
So, minimum integer ratio is 2/1
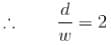
The correct answer is: 2
Most Upvoted Answer
In a double slit interference experiment d is the distance between the...
To find the minimum integer ratio of d, we need to simplify the expression d/w as much as possible.
We know that both d and w are positive quantities, so we can assume that d > w.
To simplify d/w, we can divide both the numerator and denominator by the greatest common divisor (gcd) of d and w.
Let's say gcd(d, w) = x.
Then, we can write d = xa and w = xb, where a and b are positive integers greater than 1, and gcd(a, b) = 1.
Now, d/w = (xa)/(xb) = a/b.
Since a/b is a ratio of two positive integers, the minimum integer ratio of d/w is a/b.
Therefore, the minimum integer ratio of d is a.
We know that both d and w are positive quantities, so we can assume that d > w.
To simplify d/w, we can divide both the numerator and denominator by the greatest common divisor (gcd) of d and w.
Let's say gcd(d, w) = x.
Then, we can write d = xa and w = xb, where a and b are positive integers greater than 1, and gcd(a, b) = 1.
Now, d/w = (xa)/(xb) = a/b.
Since a/b is a ratio of two positive integers, the minimum integer ratio of d/w is a/b.
Therefore, the minimum integer ratio of d is a.

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
In a double slit interference experiment d is the distance between the centres of theslits andw is the width of each slit.Find the minimum integer ratio d/ωfor which aninterference maximum of incident plane waves on a distant screen will be missing.Correct answer is '2'. Can you explain this answer?
Question Description
In a double slit interference experiment d is the distance between the centres of theslits andw is the width of each slit.Find the minimum integer ratio d/ωfor which aninterference maximum of incident plane waves on a distant screen will be missing.Correct answer is '2'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about In a double slit interference experiment d is the distance between the centres of theslits andw is the width of each slit.Find the minimum integer ratio d/ωfor which aninterference maximum of incident plane waves on a distant screen will be missing.Correct answer is '2'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In a double slit interference experiment d is the distance between the centres of theslits andw is the width of each slit.Find the minimum integer ratio d/ωfor which aninterference maximum of incident plane waves on a distant screen will be missing.Correct answer is '2'. Can you explain this answer?.
In a double slit interference experiment d is the distance between the centres of theslits andw is the width of each slit.Find the minimum integer ratio d/ωfor which aninterference maximum of incident plane waves on a distant screen will be missing.Correct answer is '2'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about In a double slit interference experiment d is the distance between the centres of theslits andw is the width of each slit.Find the minimum integer ratio d/ωfor which aninterference maximum of incident plane waves on a distant screen will be missing.Correct answer is '2'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In a double slit interference experiment d is the distance between the centres of theslits andw is the width of each slit.Find the minimum integer ratio d/ωfor which aninterference maximum of incident plane waves on a distant screen will be missing.Correct answer is '2'. Can you explain this answer?.
Solutions for In a double slit interference experiment d is the distance between the centres of theslits andw is the width of each slit.Find the minimum integer ratio d/ωfor which aninterference maximum of incident plane waves on a distant screen will be missing.Correct answer is '2'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of In a double slit interference experiment d is the distance between the centres of theslits andw is the width of each slit.Find the minimum integer ratio d/ωfor which aninterference maximum of incident plane waves on a distant screen will be missing.Correct answer is '2'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
In a double slit interference experiment d is the distance between the centres of theslits andw is the width of each slit.Find the minimum integer ratio d/ωfor which aninterference maximum of incident plane waves on a distant screen will be missing.Correct answer is '2'. Can you explain this answer?, a detailed solution for In a double slit interference experiment d is the distance between the centres of theslits andw is the width of each slit.Find the minimum integer ratio d/ωfor which aninterference maximum of incident plane waves on a distant screen will be missing.Correct answer is '2'. Can you explain this answer? has been provided alongside types of In a double slit interference experiment d is the distance between the centres of theslits andw is the width of each slit.Find the minimum integer ratio d/ωfor which aninterference maximum of incident plane waves on a distant screen will be missing.Correct answer is '2'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice In a double slit interference experiment d is the distance between the centres of theslits andw is the width of each slit.Find the minimum integer ratio d/ωfor which aninterference maximum of incident plane waves on a distant screen will be missing.Correct answer is '2'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















