Physics Exam > Physics Questions > A particle is executing SHM with an amplitude...
Start Learning for Free
A particle is executing SHM with an amplitude 4 cm. The displacement (in cm) at which its energy it half kinetic and half potential is :
Correct answer is '2.828'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A particle is executing SHM with an amplitude 4 cm. The displacement (...
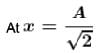
Potential energy = Kinetic energy
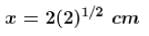
The correct answer is: 2.828
Most Upvoted Answer
A particle is executing SHM with an amplitude 4 cm. The displacement (...
Given:
- Amplitude of SHM = 4 cm
To find:
- Displacement at which the energy is half kinetic and half potential
Explanation:
1. Understanding Simple Harmonic Motion (SHM):
- Simple Harmonic Motion (SHM) is a type of periodic motion in which the restoring force is directly proportional to the displacement of the particle from its equilibrium position.
- The displacement of the particle from its equilibrium position is given by the equation:
x = A*cos(ωt + φ)
where x is the displacement, A is the amplitude, ω is the angular frequency, t is the time, and φ is the phase constant.
2. Energy in SHM:
- The total mechanical energy of a particle executing SHM is the sum of its kinetic energy and potential energy.
- The kinetic energy (KE) of the particle is given by the equation:
KE = 1/2 * m * v^2
where m is the mass of the particle and v is its velocity.
- The potential energy (PE) of the particle is given by the equation:
PE = 1/2 * k * x^2
where k is the spring constant and x is the displacement of the particle.
3. Finding the Displacement:
- The total mechanical energy (E) of the particle is constant throughout the motion and is given by:
E = KE + PE
- Since the energy is half kinetic and half potential, we can write:
KE = 1/2 * E
PE = 1/2 * E
- Substituting the equations for KE and PE, we get:
1/2 * m * v^2 = 1/2 * E
1/2 * k * x^2 = 1/2 * E
- Rearranging the equations, we find:
v^2 = E/m
x^2 = E/k
- Since E is constant, we can write:
v^2 = x^2 * k/m
- Using the equation for velocity in SHM, we have:
v = -A * ω * sin(ωt + φ)
- Substituting this equation into the previous equation, we get:
(-A * ω * sin(ωt + φ))^2 = x^2 * k/m
- Simplifying the equation, we find:
A^2 * ω^2 * sin^2(ωt + φ) = x^2 * k/m
- Since the amplitude is given, we can write:
4^2 * ω^2 * sin^2(ωt + φ) = x^2 * k/m
16 * ω^2 * sin^2(ωt + φ) = x^2 * k/m
- The maximum value of sin^2(ωt + φ) is 1, so we can write:
16 * ω^2 = x^2 * k/m
- Rearranging the equation, we find:
x^2 = 16 * ω^2 * m/k
- Substituting the value of ω^2 (ω^2 = k/m),
- Amplitude of SHM = 4 cm
To find:
- Displacement at which the energy is half kinetic and half potential
Explanation:
1. Understanding Simple Harmonic Motion (SHM):
- Simple Harmonic Motion (SHM) is a type of periodic motion in which the restoring force is directly proportional to the displacement of the particle from its equilibrium position.
- The displacement of the particle from its equilibrium position is given by the equation:
x = A*cos(ωt + φ)
where x is the displacement, A is the amplitude, ω is the angular frequency, t is the time, and φ is the phase constant.
2. Energy in SHM:
- The total mechanical energy of a particle executing SHM is the sum of its kinetic energy and potential energy.
- The kinetic energy (KE) of the particle is given by the equation:
KE = 1/2 * m * v^2
where m is the mass of the particle and v is its velocity.
- The potential energy (PE) of the particle is given by the equation:
PE = 1/2 * k * x^2
where k is the spring constant and x is the displacement of the particle.
3. Finding the Displacement:
- The total mechanical energy (E) of the particle is constant throughout the motion and is given by:
E = KE + PE
- Since the energy is half kinetic and half potential, we can write:
KE = 1/2 * E
PE = 1/2 * E
- Substituting the equations for KE and PE, we get:
1/2 * m * v^2 = 1/2 * E
1/2 * k * x^2 = 1/2 * E
- Rearranging the equations, we find:
v^2 = E/m
x^2 = E/k
- Since E is constant, we can write:
v^2 = x^2 * k/m
- Using the equation for velocity in SHM, we have:
v = -A * ω * sin(ωt + φ)
- Substituting this equation into the previous equation, we get:
(-A * ω * sin(ωt + φ))^2 = x^2 * k/m
- Simplifying the equation, we find:
A^2 * ω^2 * sin^2(ωt + φ) = x^2 * k/m
- Since the amplitude is given, we can write:
4^2 * ω^2 * sin^2(ωt + φ) = x^2 * k/m
16 * ω^2 * sin^2(ωt + φ) = x^2 * k/m
- The maximum value of sin^2(ωt + φ) is 1, so we can write:
16 * ω^2 = x^2 * k/m
- Rearranging the equation, we find:
x^2 = 16 * ω^2 * m/k
- Substituting the value of ω^2 (ω^2 = k/m),
Free Test
FREE
| Start Free Test |
Community Answer
A particle is executing SHM with an amplitude 4 cm. The displacement (...
2.82842

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
A particle is executing SHM with an amplitude 4 cm. The displacement (in cm) at which its energy it half kinetic and half potential is :Correct answer is '2.828'. Can you explain this answer?
Question Description
A particle is executing SHM with an amplitude 4 cm. The displacement (in cm) at which its energy it half kinetic and half potential is :Correct answer is '2.828'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about A particle is executing SHM with an amplitude 4 cm. The displacement (in cm) at which its energy it half kinetic and half potential is :Correct answer is '2.828'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A particle is executing SHM with an amplitude 4 cm. The displacement (in cm) at which its energy it half kinetic and half potential is :Correct answer is '2.828'. Can you explain this answer?.
A particle is executing SHM with an amplitude 4 cm. The displacement (in cm) at which its energy it half kinetic and half potential is :Correct answer is '2.828'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about A particle is executing SHM with an amplitude 4 cm. The displacement (in cm) at which its energy it half kinetic and half potential is :Correct answer is '2.828'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A particle is executing SHM with an amplitude 4 cm. The displacement (in cm) at which its energy it half kinetic and half potential is :Correct answer is '2.828'. Can you explain this answer?.
Solutions for A particle is executing SHM with an amplitude 4 cm. The displacement (in cm) at which its energy it half kinetic and half potential is :Correct answer is '2.828'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of A particle is executing SHM with an amplitude 4 cm. The displacement (in cm) at which its energy it half kinetic and half potential is :Correct answer is '2.828'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A particle is executing SHM with an amplitude 4 cm. The displacement (in cm) at which its energy it half kinetic and half potential is :Correct answer is '2.828'. Can you explain this answer?, a detailed solution for A particle is executing SHM with an amplitude 4 cm. The displacement (in cm) at which its energy it half kinetic and half potential is :Correct answer is '2.828'. Can you explain this answer? has been provided alongside types of A particle is executing SHM with an amplitude 4 cm. The displacement (in cm) at which its energy it half kinetic and half potential is :Correct answer is '2.828'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A particle is executing SHM with an amplitude 4 cm. The displacement (in cm) at which its energy it half kinetic and half potential is :Correct answer is '2.828'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.













