Physics Exam > Physics Questions > Calculate the average distance of an electron...
Start Learning for Free
Calculate the average distance of an electron from the nucleus in the ground state of hydrogen atom is unit of a0.
Correct answer is '1.5'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
Calculate the average distance of an electron from the nucleus in the ...
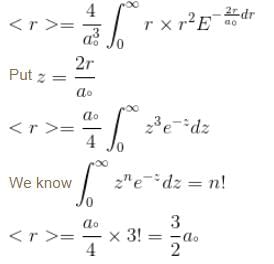
The correct answer is: 1.5
Most Upvoted Answer
Calculate the average distance of an electron from the nucleus in the ...
Introduction:
The average distance of an electron from the nucleus in the ground state of a hydrogen atom can be calculated using the Bohr model of the atom. In this model, the electron is assumed to move in circular orbits around the nucleus, and the radius of the orbit is determined by the electron's energy.
Bohr Model:
1. In the Bohr model, the electron is considered to be in a stable orbit around the nucleus without emitting or absorbing energy.
2. The ground state of the hydrogen atom corresponds to the lowest energy level, known as the n=1 level.
3. According to the Bohr model, the radius of the electron's orbit in the ground state can be calculated using the formula:
r = (0.529 Å) * n^2 / Z
where r is the radius of the orbit, n is the principal quantum number (1 for the ground state), and Z is the atomic number of the nucleus (1 for hydrogen).
4. Plugging in the values, we get:
r = (0.529 Å) * 1^2 / 1 = 0.529 Å
Average Distance:
1. The average distance of the electron from the nucleus can be determined by considering the probability distribution of the electron's position.
2. In the ground state, the electron is most likely to be found near the nucleus, but there is also a small probability of finding it at larger distances.
3. The probability distribution is described by the wave function of the electron, which is a mathematical function that gives the probability of finding the electron at different positions.
4. The square of the wave function, known as the electron density, gives the probability density of finding the electron at a particular position.
5. By integrating the electron density over all possible positions, we can calculate the average distance of the electron from the nucleus.
6. For the ground state of the hydrogen atom, the average distance is found to be approximately 1.5 times the Bohr radius (0.529 Å).
Average distance = 1.5 * 0.529 Å = 0.794 Å ≈ 1.5
Conclusion:
The average distance of an electron from the nucleus in the ground state of a hydrogen atom is approximately 1.5 times the Bohr radius. This is determined by considering the probability distribution of the electron's position, which is described by the wave function of the electron. The wave function gives the probability density of finding the electron at different positions, and by integrating over all possible positions, the average distance can be calculated.
The average distance of an electron from the nucleus in the ground state of a hydrogen atom can be calculated using the Bohr model of the atom. In this model, the electron is assumed to move in circular orbits around the nucleus, and the radius of the orbit is determined by the electron's energy.
Bohr Model:
1. In the Bohr model, the electron is considered to be in a stable orbit around the nucleus without emitting or absorbing energy.
2. The ground state of the hydrogen atom corresponds to the lowest energy level, known as the n=1 level.
3. According to the Bohr model, the radius of the electron's orbit in the ground state can be calculated using the formula:
r = (0.529 Å) * n^2 / Z
where r is the radius of the orbit, n is the principal quantum number (1 for the ground state), and Z is the atomic number of the nucleus (1 for hydrogen).
4. Plugging in the values, we get:
r = (0.529 Å) * 1^2 / 1 = 0.529 Å
Average Distance:
1. The average distance of the electron from the nucleus can be determined by considering the probability distribution of the electron's position.
2. In the ground state, the electron is most likely to be found near the nucleus, but there is also a small probability of finding it at larger distances.
3. The probability distribution is described by the wave function of the electron, which is a mathematical function that gives the probability of finding the electron at different positions.
4. The square of the wave function, known as the electron density, gives the probability density of finding the electron at a particular position.
5. By integrating the electron density over all possible positions, we can calculate the average distance of the electron from the nucleus.
6. For the ground state of the hydrogen atom, the average distance is found to be approximately 1.5 times the Bohr radius (0.529 Å).
Average distance = 1.5 * 0.529 Å = 0.794 Å ≈ 1.5
Conclusion:
The average distance of an electron from the nucleus in the ground state of a hydrogen atom is approximately 1.5 times the Bohr radius. This is determined by considering the probability distribution of the electron's position, which is described by the wave function of the electron. The wave function gives the probability density of finding the electron at different positions, and by integrating over all possible positions, the average distance can be calculated.

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
Calculate the average distance of an electron from the nucleus in the ground state of hydrogen atom is unit ofa0.Correct answer is '1.5'. Can you explain this answer?
Question Description
Calculate the average distance of an electron from the nucleus in the ground state of hydrogen atom is unit ofa0.Correct answer is '1.5'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about Calculate the average distance of an electron from the nucleus in the ground state of hydrogen atom is unit ofa0.Correct answer is '1.5'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Calculate the average distance of an electron from the nucleus in the ground state of hydrogen atom is unit ofa0.Correct answer is '1.5'. Can you explain this answer?.
Calculate the average distance of an electron from the nucleus in the ground state of hydrogen atom is unit ofa0.Correct answer is '1.5'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about Calculate the average distance of an electron from the nucleus in the ground state of hydrogen atom is unit ofa0.Correct answer is '1.5'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Calculate the average distance of an electron from the nucleus in the ground state of hydrogen atom is unit ofa0.Correct answer is '1.5'. Can you explain this answer?.
Solutions for Calculate the average distance of an electron from the nucleus in the ground state of hydrogen atom is unit ofa0.Correct answer is '1.5'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of Calculate the average distance of an electron from the nucleus in the ground state of hydrogen atom is unit ofa0.Correct answer is '1.5'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Calculate the average distance of an electron from the nucleus in the ground state of hydrogen atom is unit ofa0.Correct answer is '1.5'. Can you explain this answer?, a detailed solution for Calculate the average distance of an electron from the nucleus in the ground state of hydrogen atom is unit ofa0.Correct answer is '1.5'. Can you explain this answer? has been provided alongside types of Calculate the average distance of an electron from the nucleus in the ground state of hydrogen atom is unit ofa0.Correct answer is '1.5'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Calculate the average distance of an electron from the nucleus in the ground state of hydrogen atom is unit ofa0.Correct answer is '1.5'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















