Physics Exam > Physics Questions > The solutions of a free particle are :a)Momen...
Start Learning for Free
The solutions of a free particle are :
- a)Momentum eigen functions with only negative eigenvalues
- b)Energy and momentum eigen functions with both positive and negative momentum eigenvalues.
- c)Energy and momentum eigen functions with positive momentum eigenvalues
- d)Only energy eigen functions.
Correct answer is option 'B'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
The solutions of a free particle are :a)Momentum eigen functions with ...
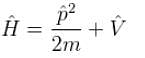
V = 0 for a free particle
∴
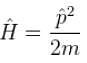
Schrödinger equation.
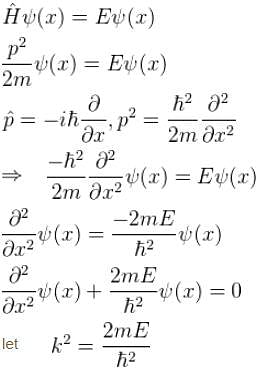
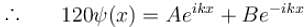 (General solution)
(General solution)
∴ Aeikx and Be–ikx are solution to the free particle

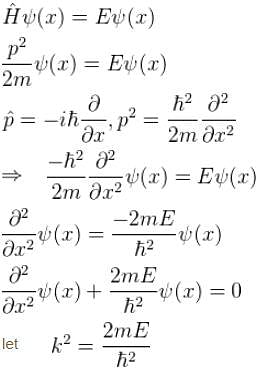
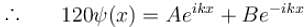 (General solution)
(General solution)∴ Aeikx and Be–ikx are solution to the free particle

The correct answer is: Energy and momentum eigen functions with both positive and negative momentum eigenvalues.
Most Upvoted Answer
The solutions of a free particle are :a)Momentum eigen functions with ...
Introduction:
The solutions of a free particle refer to the possible wavefunctions that describe the motion of a particle that is not subject to any external forces. These solutions are obtained by solving the time-independent Schrödinger equation for a particle with no potential energy.
Explanation:
The time-independent Schrödinger equation for a free particle is given by:
Ĥψ = Eψ
Where:
- Ĥ represents the Hamiltonian operator, which describes the total energy of the particle.
- ψ represents the wavefunction of the particle.
- E represents the energy eigenvalue associated with the wavefunction.
Energy eigenfunctions:
The solutions to the Schrödinger equation are known as energy eigenfunctions. These are wavefunctions that satisfy the equation and correspond to specific energy eigenvalues. In the case of a free particle, the energy eigenvalues are continuous and can take on any positive value. Therefore, the solutions include energy eigenfunctions with positive energy eigenvalues.
Momentum eigenfunctions:
In quantum mechanics, momentum is represented by the momentum operator, p. The momentum eigenfunctions are the solutions to the momentum operator acting on the wavefunction. For a free particle, the momentum eigenvalues can be positive or negative, as the particle can move in either direction. Therefore, the solutions include momentum eigenfunctions with both positive and negative momentum eigenvalues.
Energy and momentum eigenfunctions:
Since the solutions to the Schrödinger equation for a free particle include both energy and momentum eigenfunctions, option B is correct. These wavefunctions describe the particle's motion in terms of both its energy and momentum.
Momentum eigenfunctions with only negative eigenvalues:
Option A is incorrect because the solutions to the Schrödinger equation for a free particle include momentum eigenfunctions with both positive and negative eigenvalues.
Only energy eigenfunctions:
Option D is incorrect because the solutions to the Schrödinger equation for a free particle include both energy and momentum eigenfunctions.
Conclusion:
In summary, the solutions of a free particle include energy and momentum eigenfunctions with both positive and negative momentum eigenvalues. These wavefunctions describe the particle's motion in terms of both its energy and momentum and satisfy the time-independent Schrödinger equation.
The solutions of a free particle refer to the possible wavefunctions that describe the motion of a particle that is not subject to any external forces. These solutions are obtained by solving the time-independent Schrödinger equation for a particle with no potential energy.
Explanation:
The time-independent Schrödinger equation for a free particle is given by:
Ĥψ = Eψ
Where:
- Ĥ represents the Hamiltonian operator, which describes the total energy of the particle.
- ψ represents the wavefunction of the particle.
- E represents the energy eigenvalue associated with the wavefunction.
Energy eigenfunctions:
The solutions to the Schrödinger equation are known as energy eigenfunctions. These are wavefunctions that satisfy the equation and correspond to specific energy eigenvalues. In the case of a free particle, the energy eigenvalues are continuous and can take on any positive value. Therefore, the solutions include energy eigenfunctions with positive energy eigenvalues.
Momentum eigenfunctions:
In quantum mechanics, momentum is represented by the momentum operator, p. The momentum eigenfunctions are the solutions to the momentum operator acting on the wavefunction. For a free particle, the momentum eigenvalues can be positive or negative, as the particle can move in either direction. Therefore, the solutions include momentum eigenfunctions with both positive and negative momentum eigenvalues.
Energy and momentum eigenfunctions:
Since the solutions to the Schrödinger equation for a free particle include both energy and momentum eigenfunctions, option B is correct. These wavefunctions describe the particle's motion in terms of both its energy and momentum.
Momentum eigenfunctions with only negative eigenvalues:
Option A is incorrect because the solutions to the Schrödinger equation for a free particle include momentum eigenfunctions with both positive and negative eigenvalues.
Only energy eigenfunctions:
Option D is incorrect because the solutions to the Schrödinger equation for a free particle include both energy and momentum eigenfunctions.
Conclusion:
In summary, the solutions of a free particle include energy and momentum eigenfunctions with both positive and negative momentum eigenvalues. These wavefunctions describe the particle's motion in terms of both its energy and momentum and satisfy the time-independent Schrödinger equation.

|
Explore Courses for Physics exam
|

|
The solutions of a free particle are :a)Momentum eigen functions with only negative eigenvaluesb)Energy and momentum eigen functions with both positive and negative momentum eigenvalues.c)Energy and momentum eigen functions with positive momentum eigenvaluesd)Only energy eigen functions.Correct answer is option 'B'. Can you explain this answer?
Question Description
The solutions of a free particle are :a)Momentum eigen functions with only negative eigenvaluesb)Energy and momentum eigen functions with both positive and negative momentum eigenvalues.c)Energy and momentum eigen functions with positive momentum eigenvaluesd)Only energy eigen functions.Correct answer is option 'B'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about The solutions of a free particle are :a)Momentum eigen functions with only negative eigenvaluesb)Energy and momentum eigen functions with both positive and negative momentum eigenvalues.c)Energy and momentum eigen functions with positive momentum eigenvaluesd)Only energy eigen functions.Correct answer is option 'B'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The solutions of a free particle are :a)Momentum eigen functions with only negative eigenvaluesb)Energy and momentum eigen functions with both positive and negative momentum eigenvalues.c)Energy and momentum eigen functions with positive momentum eigenvaluesd)Only energy eigen functions.Correct answer is option 'B'. Can you explain this answer?.
The solutions of a free particle are :a)Momentum eigen functions with only negative eigenvaluesb)Energy and momentum eigen functions with both positive and negative momentum eigenvalues.c)Energy and momentum eigen functions with positive momentum eigenvaluesd)Only energy eigen functions.Correct answer is option 'B'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about The solutions of a free particle are :a)Momentum eigen functions with only negative eigenvaluesb)Energy and momentum eigen functions with both positive and negative momentum eigenvalues.c)Energy and momentum eigen functions with positive momentum eigenvaluesd)Only energy eigen functions.Correct answer is option 'B'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The solutions of a free particle are :a)Momentum eigen functions with only negative eigenvaluesb)Energy and momentum eigen functions with both positive and negative momentum eigenvalues.c)Energy and momentum eigen functions with positive momentum eigenvaluesd)Only energy eigen functions.Correct answer is option 'B'. Can you explain this answer?.
Solutions for The solutions of a free particle are :a)Momentum eigen functions with only negative eigenvaluesb)Energy and momentum eigen functions with both positive and negative momentum eigenvalues.c)Energy and momentum eigen functions with positive momentum eigenvaluesd)Only energy eigen functions.Correct answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of The solutions of a free particle are :a)Momentum eigen functions with only negative eigenvaluesb)Energy and momentum eigen functions with both positive and negative momentum eigenvalues.c)Energy and momentum eigen functions with positive momentum eigenvaluesd)Only energy eigen functions.Correct answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The solutions of a free particle are :a)Momentum eigen functions with only negative eigenvaluesb)Energy and momentum eigen functions with both positive and negative momentum eigenvalues.c)Energy and momentum eigen functions with positive momentum eigenvaluesd)Only energy eigen functions.Correct answer is option 'B'. Can you explain this answer?, a detailed solution for The solutions of a free particle are :a)Momentum eigen functions with only negative eigenvaluesb)Energy and momentum eigen functions with both positive and negative momentum eigenvalues.c)Energy and momentum eigen functions with positive momentum eigenvaluesd)Only energy eigen functions.Correct answer is option 'B'. Can you explain this answer? has been provided alongside types of The solutions of a free particle are :a)Momentum eigen functions with only negative eigenvaluesb)Energy and momentum eigen functions with both positive and negative momentum eigenvalues.c)Energy and momentum eigen functions with positive momentum eigenvaluesd)Only energy eigen functions.Correct answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The solutions of a free particle are :a)Momentum eigen functions with only negative eigenvaluesb)Energy and momentum eigen functions with both positive and negative momentum eigenvalues.c)Energy and momentum eigen functions with positive momentum eigenvaluesd)Only energy eigen functions.Correct answer is option 'B'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
















