Class 10 Exam > Class 10 Questions > A solid toy is in the form of a right circula...
Start Learning for Free
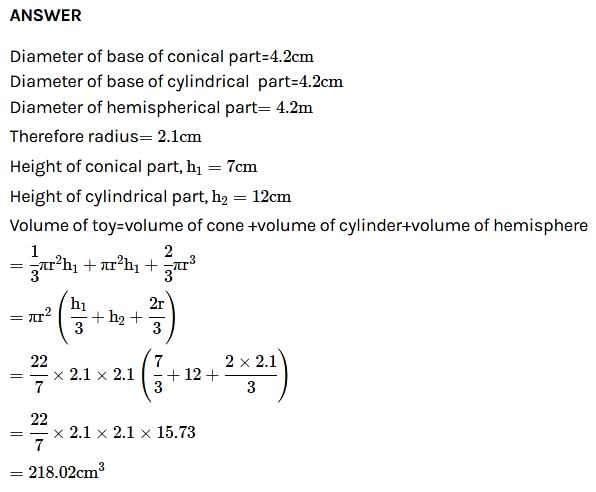
A solid toy is in the form of a right circular cylinder with a hemispherical shape at one end and a cone at the other end. Their common diameter is 4.2 cm and the heights of the cylindrical and conical portions are 12 cm and 7 cm respectively. The approximate volume of the toy will be
- a)250 cm3
- b)200 cm3
- c)300 cm3
- d)218 cm3
Correct answer is option 'D'. Can you explain this answer?
Most Upvoted Answer
A solid toy is in the form of a right circular cylinder with a hemisph...
Free Test
FREE
| Start Free Test |
Community Answer
A solid toy is in the form of a right circular cylinder with a hemisph...
To find the volume of the toy, we need to find the volumes of the cylindrical, hemispherical, and conical parts separately and then add them together.
1. Volume of the Cylinder:
The formula for the volume of a cylinder is V = πr²h, where r is the radius and h is the height. Since the diameter is given as 4.2 cm, the radius (r) is half of that, which is 2.1 cm. The height (h) is given as 12 cm. Substituting these values into the formula, we get:
Vcylinder = π(2.1 cm)²(12 cm)
Vcylinder ≈ 166.32 cm³
2. Volume of the Hemisphere:
The formula for the volume of a hemisphere is V = (2/3)πr³, where r is the radius. Since the diameter is given as 4.2 cm, the radius (r) is half of that, which is 2.1 cm. Substituting this value into the formula, we get:
Vhemisphere = (2/3)π(2.1 cm)³
Vhemisphere ≈ 24.572 cm³
3. Volume of the Cone:
The formula for the volume of a cone is V = (1/3)πr²h, where r is the radius and h is the height. Since the diameter is given as 4.2 cm, the radius (r) is half of that, which is 2.1 cm. The height (h) is given as 7 cm. Substituting these values into the formula, we get:
Vcone = (1/3)π(2.1 cm)²(7 cm)
Vcone ≈ 10.972 cm³
4. Total Volume:
To find the total volume of the toy, we add the volumes of the cylindrical, hemispherical, and conical parts together:
Vtotal = Vcylinder + Vhemisphere + Vcone
Vtotal ≈ 166.32 cm³ + 24.572 cm³ + 10.972 cm³
Vtotal ≈ 201.864 cm³
Since the volume of the toy is approximately 201.864 cm³, the closest option is 218 cm³ (option D).
1. Volume of the Cylinder:
The formula for the volume of a cylinder is V = πr²h, where r is the radius and h is the height. Since the diameter is given as 4.2 cm, the radius (r) is half of that, which is 2.1 cm. The height (h) is given as 12 cm. Substituting these values into the formula, we get:
Vcylinder = π(2.1 cm)²(12 cm)
Vcylinder ≈ 166.32 cm³
2. Volume of the Hemisphere:
The formula for the volume of a hemisphere is V = (2/3)πr³, where r is the radius. Since the diameter is given as 4.2 cm, the radius (r) is half of that, which is 2.1 cm. Substituting this value into the formula, we get:
Vhemisphere = (2/3)π(2.1 cm)³
Vhemisphere ≈ 24.572 cm³
3. Volume of the Cone:
The formula for the volume of a cone is V = (1/3)πr²h, where r is the radius and h is the height. Since the diameter is given as 4.2 cm, the radius (r) is half of that, which is 2.1 cm. The height (h) is given as 7 cm. Substituting these values into the formula, we get:
Vcone = (1/3)π(2.1 cm)²(7 cm)
Vcone ≈ 10.972 cm³
4. Total Volume:
To find the total volume of the toy, we add the volumes of the cylindrical, hemispherical, and conical parts together:
Vtotal = Vcylinder + Vhemisphere + Vcone
Vtotal ≈ 166.32 cm³ + 24.572 cm³ + 10.972 cm³
Vtotal ≈ 201.864 cm³
Since the volume of the toy is approximately 201.864 cm³, the closest option is 218 cm³ (option D).
|
Explore Courses for Class 10 exam
|
|
Top Courses for Class 10View all
Question Description
A solid toy is in the form of a right circular cylinder with a hemispherical shape at one end and a cone at the other end. Their common diameter is 4.2 cm and the heights of the cylindrical and conical portions are 12 cm and 7 cm respectively. The approximate volume of the toy will bea)250 cm3b)200 cm3c)300 cm3d)218 cm3Correct answer is option 'D'. Can you explain this answer? for Class 10 2025 is part of Class 10 preparation. The Question and answers have been prepared according to the Class 10 exam syllabus. Information about A solid toy is in the form of a right circular cylinder with a hemispherical shape at one end and a cone at the other end. Their common diameter is 4.2 cm and the heights of the cylindrical and conical portions are 12 cm and 7 cm respectively. The approximate volume of the toy will bea)250 cm3b)200 cm3c)300 cm3d)218 cm3Correct answer is option 'D'. Can you explain this answer? covers all topics & solutions for Class 10 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A solid toy is in the form of a right circular cylinder with a hemispherical shape at one end and a cone at the other end. Their common diameter is 4.2 cm and the heights of the cylindrical and conical portions are 12 cm and 7 cm respectively. The approximate volume of the toy will bea)250 cm3b)200 cm3c)300 cm3d)218 cm3Correct answer is option 'D'. Can you explain this answer?.
A solid toy is in the form of a right circular cylinder with a hemispherical shape at one end and a cone at the other end. Their common diameter is 4.2 cm and the heights of the cylindrical and conical portions are 12 cm and 7 cm respectively. The approximate volume of the toy will bea)250 cm3b)200 cm3c)300 cm3d)218 cm3Correct answer is option 'D'. Can you explain this answer? for Class 10 2025 is part of Class 10 preparation. The Question and answers have been prepared according to the Class 10 exam syllabus. Information about A solid toy is in the form of a right circular cylinder with a hemispherical shape at one end and a cone at the other end. Their common diameter is 4.2 cm and the heights of the cylindrical and conical portions are 12 cm and 7 cm respectively. The approximate volume of the toy will bea)250 cm3b)200 cm3c)300 cm3d)218 cm3Correct answer is option 'D'. Can you explain this answer? covers all topics & solutions for Class 10 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A solid toy is in the form of a right circular cylinder with a hemispherical shape at one end and a cone at the other end. Their common diameter is 4.2 cm and the heights of the cylindrical and conical portions are 12 cm and 7 cm respectively. The approximate volume of the toy will bea)250 cm3b)200 cm3c)300 cm3d)218 cm3Correct answer is option 'D'. Can you explain this answer?.
Solutions for A solid toy is in the form of a right circular cylinder with a hemispherical shape at one end and a cone at the other end. Their common diameter is 4.2 cm and the heights of the cylindrical and conical portions are 12 cm and 7 cm respectively. The approximate volume of the toy will bea)250 cm3b)200 cm3c)300 cm3d)218 cm3Correct answer is option 'D'. Can you explain this answer? in English & in Hindi are available as part of our courses for Class 10.
Download more important topics, notes, lectures and mock test series for Class 10 Exam by signing up for free.
Here you can find the meaning of A solid toy is in the form of a right circular cylinder with a hemispherical shape at one end and a cone at the other end. Their common diameter is 4.2 cm and the heights of the cylindrical and conical portions are 12 cm and 7 cm respectively. The approximate volume of the toy will bea)250 cm3b)200 cm3c)300 cm3d)218 cm3Correct answer is option 'D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A solid toy is in the form of a right circular cylinder with a hemispherical shape at one end and a cone at the other end. Their common diameter is 4.2 cm and the heights of the cylindrical and conical portions are 12 cm and 7 cm respectively. The approximate volume of the toy will bea)250 cm3b)200 cm3c)300 cm3d)218 cm3Correct answer is option 'D'. Can you explain this answer?, a detailed solution for A solid toy is in the form of a right circular cylinder with a hemispherical shape at one end and a cone at the other end. Their common diameter is 4.2 cm and the heights of the cylindrical and conical portions are 12 cm and 7 cm respectively. The approximate volume of the toy will bea)250 cm3b)200 cm3c)300 cm3d)218 cm3Correct answer is option 'D'. Can you explain this answer? has been provided alongside types of A solid toy is in the form of a right circular cylinder with a hemispherical shape at one end and a cone at the other end. Their common diameter is 4.2 cm and the heights of the cylindrical and conical portions are 12 cm and 7 cm respectively. The approximate volume of the toy will bea)250 cm3b)200 cm3c)300 cm3d)218 cm3Correct answer is option 'D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A solid toy is in the form of a right circular cylinder with a hemispherical shape at one end and a cone at the other end. Their common diameter is 4.2 cm and the heights of the cylindrical and conical portions are 12 cm and 7 cm respectively. The approximate volume of the toy will bea)250 cm3b)200 cm3c)300 cm3d)218 cm3Correct answer is option 'D'. Can you explain this answer? tests, examples and also practice Class 10 tests.
|
|
Explore Courses for Class 10 exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup