Chemical Engineering Exam > Chemical Engineering Questions > In a double-pipe heat exchanger, the cold flu...
Start Learning for Free
In a double-pipe heat exchanger, the cold fluid is water with inlet temperature of 20oC and mass flow rate of 20 kg/s and the hot fluid which also has water inlet temperature of 80oC and mass flow rate of 10 kg/s. For water cp = 4.2kj/kg oC.
. What is the maximum temperature to which the cold fluid can be heated in a parallel flow and in a counter flow heat exchanger?
- a)80°C in both parallel flow and counter flow
- b)40°C in parallel flow and 80°C in counter flow
- c)40°C in parallel flow and 50°C in counter flow
- d)50°C in both parallel flow and counter flow
Correct answer is option 'C'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
In a double-pipe heat exchanger, the cold fluid is water with inlet te...
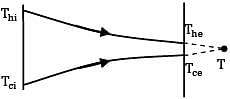
For parallel flow heat exchanger maximum temperature of cold fluid will be reached when both hot and cold fluid exit at the same temperature.
Thus by steady state flow energy equation
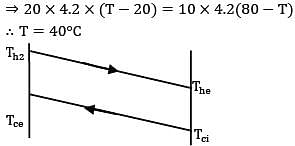
⇒ 20 x 4.2 x (T - 20) = 10 x 4.2 (80-T)
∴ T = 40oC
For counter flow heat exchanger, maximum exit temperature of cold fluid is achieved when effectiveness of the heat exchanger is 1
i.e.
ε = 1
i.e.
(mccpc)(Tco - Tci)/(mccpc)(Thi - Tci) =1
1 = 20 x 4.2 x [T - 20]/10 x 4.2 x [80 - 20]
T = 50oC
Most Upvoted Answer
In a double-pipe heat exchanger, the cold fluid is water with inlet te...
Given:
Cold fluid temperature, T1 = 20°C
Hot fluid temperature, T2 = 80°C
Mass flow rate of cold fluid, m1 = 20 kg/s
Mass flow rate of hot fluid, m2 = 10 kg/s
Specific heat of water, cp = 4.2 kJ/kg°C
To find:
Maximum temperature to which the cold fluid can be heated in a parallel flow and in a counter flow heat exchanger
Solution:
The maximum temperature to which the cold fluid can be heated in a heat exchanger depends on the effectiveness of the heat exchanger. The effectiveness of the heat exchanger depends on the flow arrangement of the fluids.
Parallel flow heat exchanger:
In a parallel flow heat exchanger, the hot and cold fluids flow in the same direction. The effectiveness of the parallel flow heat exchanger is given by:
ε = (T2 - T1)/(T2 - T1') ----(1)
where T1' is the outlet temperature of the cold fluid.
The maximum temperature to which the cold fluid can be heated in a parallel flow heat exchanger is obtained by setting ε = 1 in equation (1) and solving for T1':
1 = (T2 - T1)/(T2 - T1')
T1' = T2 - (T2 - T1)/1
T1' = 2T2 - T1
T1' = 2(80) - 20
T1' = 140°C
Therefore, the maximum temperature to which the cold fluid can be heated in a parallel flow heat exchanger is 140°C.
Counter flow heat exchanger:
In a counter flow heat exchanger, the hot and cold fluids flow in opposite directions. The effectiveness of the counter flow heat exchanger is given by:
ε = (T2 - T1)/(T2 - T1') * ln[(T2 - T1)/(T2 - T1')] / ln[(T2 - T1)/(T2 - T1'')] ----(2)
where T1'' is the outlet temperature of the hot fluid.
The maximum temperature to which the cold fluid can be heated in a counter flow heat exchanger is obtained by setting ε = 1 in equation (2) and solving for T1':
1 = (T2 - T1)/(T2 - T1') * ln[(T2 - T1)/(T2 - T1')] / ln[(T2 - T1)/(T2 - T1'')]
T1' = T2 - (T2 - T1)/[(1)(ln[(T2 - T1)/(T2 - T1')]/ln[(T2 - T1)/(T2 - T1'')])]
T1' = T2 - (T2 - T1)/(ln[(T2 - T1)/(T2 - T1'')]/ln[(T2 - T1)/(T2 - T1')])
T1' = T2 - (T2 - T1)/(ln[(T2 - T1)/(T2 - T1'')]/ln[(T2 - T1)/(T2 - T1')])
T1' = 2T2 - T1
T1' = 2(80) - 20
T1' = 140°C
Therefore, the maximum temperature to which the cold fluid
Cold fluid temperature, T1 = 20°C
Hot fluid temperature, T2 = 80°C
Mass flow rate of cold fluid, m1 = 20 kg/s
Mass flow rate of hot fluid, m2 = 10 kg/s
Specific heat of water, cp = 4.2 kJ/kg°C
To find:
Maximum temperature to which the cold fluid can be heated in a parallel flow and in a counter flow heat exchanger
Solution:
The maximum temperature to which the cold fluid can be heated in a heat exchanger depends on the effectiveness of the heat exchanger. The effectiveness of the heat exchanger depends on the flow arrangement of the fluids.
Parallel flow heat exchanger:
In a parallel flow heat exchanger, the hot and cold fluids flow in the same direction. The effectiveness of the parallel flow heat exchanger is given by:
ε = (T2 - T1)/(T2 - T1') ----(1)
where T1' is the outlet temperature of the cold fluid.
The maximum temperature to which the cold fluid can be heated in a parallel flow heat exchanger is obtained by setting ε = 1 in equation (1) and solving for T1':
1 = (T2 - T1)/(T2 - T1')
T1' = T2 - (T2 - T1)/1
T1' = 2T2 - T1
T1' = 2(80) - 20
T1' = 140°C
Therefore, the maximum temperature to which the cold fluid can be heated in a parallel flow heat exchanger is 140°C.
Counter flow heat exchanger:
In a counter flow heat exchanger, the hot and cold fluids flow in opposite directions. The effectiveness of the counter flow heat exchanger is given by:
ε = (T2 - T1)/(T2 - T1') * ln[(T2 - T1)/(T2 - T1')] / ln[(T2 - T1)/(T2 - T1'')] ----(2)
where T1'' is the outlet temperature of the hot fluid.
The maximum temperature to which the cold fluid can be heated in a counter flow heat exchanger is obtained by setting ε = 1 in equation (2) and solving for T1':
1 = (T2 - T1)/(T2 - T1') * ln[(T2 - T1)/(T2 - T1')] / ln[(T2 - T1)/(T2 - T1'')]
T1' = T2 - (T2 - T1)/[(1)(ln[(T2 - T1)/(T2 - T1')]/ln[(T2 - T1)/(T2 - T1'')])]
T1' = T2 - (T2 - T1)/(ln[(T2 - T1)/(T2 - T1'')]/ln[(T2 - T1)/(T2 - T1')])
T1' = T2 - (T2 - T1)/(ln[(T2 - T1)/(T2 - T1'')]/ln[(T2 - T1)/(T2 - T1')])
T1' = 2T2 - T1
T1' = 2(80) - 20
T1' = 140°C
Therefore, the maximum temperature to which the cold fluid

|
Explore Courses for Chemical Engineering exam
|

|
Similar Chemical Engineering Doubts
In a double-pipe heat exchanger, the cold fluid is water with inlet temperature of 20oC and mass flow rate of 20 kg/s and the hot fluid which also has water inlet temperature of 80oC and mass flow rate of 10 kg/s. For water cp = 4.2kj/kg oC.. What is the maximum temperature to which the cold fluid can be heated in a parallel flow and in a counter flow heat exchanger?a)80°C in both parallel flow and counter flowb)40°C in parallel flow and 80°C in counter flowc)40°C in parallel flow and 50°C in counter flowd)50°C in both parallel flow and counter flowCorrect answer is option 'C'. Can you explain this answer?
Question Description
In a double-pipe heat exchanger, the cold fluid is water with inlet temperature of 20oC and mass flow rate of 20 kg/s and the hot fluid which also has water inlet temperature of 80oC and mass flow rate of 10 kg/s. For water cp = 4.2kj/kg oC.. What is the maximum temperature to which the cold fluid can be heated in a parallel flow and in a counter flow heat exchanger?a)80°C in both parallel flow and counter flowb)40°C in parallel flow and 80°C in counter flowc)40°C in parallel flow and 50°C in counter flowd)50°C in both parallel flow and counter flowCorrect answer is option 'C'. Can you explain this answer? for Chemical Engineering 2024 is part of Chemical Engineering preparation. The Question and answers have been prepared according to the Chemical Engineering exam syllabus. Information about In a double-pipe heat exchanger, the cold fluid is water with inlet temperature of 20oC and mass flow rate of 20 kg/s and the hot fluid which also has water inlet temperature of 80oC and mass flow rate of 10 kg/s. For water cp = 4.2kj/kg oC.. What is the maximum temperature to which the cold fluid can be heated in a parallel flow and in a counter flow heat exchanger?a)80°C in both parallel flow and counter flowb)40°C in parallel flow and 80°C in counter flowc)40°C in parallel flow and 50°C in counter flowd)50°C in both parallel flow and counter flowCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Chemical Engineering 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In a double-pipe heat exchanger, the cold fluid is water with inlet temperature of 20oC and mass flow rate of 20 kg/s and the hot fluid which also has water inlet temperature of 80oC and mass flow rate of 10 kg/s. For water cp = 4.2kj/kg oC.. What is the maximum temperature to which the cold fluid can be heated in a parallel flow and in a counter flow heat exchanger?a)80°C in both parallel flow and counter flowb)40°C in parallel flow and 80°C in counter flowc)40°C in parallel flow and 50°C in counter flowd)50°C in both parallel flow and counter flowCorrect answer is option 'C'. Can you explain this answer?.
In a double-pipe heat exchanger, the cold fluid is water with inlet temperature of 20oC and mass flow rate of 20 kg/s and the hot fluid which also has water inlet temperature of 80oC and mass flow rate of 10 kg/s. For water cp = 4.2kj/kg oC.. What is the maximum temperature to which the cold fluid can be heated in a parallel flow and in a counter flow heat exchanger?a)80°C in both parallel flow and counter flowb)40°C in parallel flow and 80°C in counter flowc)40°C in parallel flow and 50°C in counter flowd)50°C in both parallel flow and counter flowCorrect answer is option 'C'. Can you explain this answer? for Chemical Engineering 2024 is part of Chemical Engineering preparation. The Question and answers have been prepared according to the Chemical Engineering exam syllabus. Information about In a double-pipe heat exchanger, the cold fluid is water with inlet temperature of 20oC and mass flow rate of 20 kg/s and the hot fluid which also has water inlet temperature of 80oC and mass flow rate of 10 kg/s. For water cp = 4.2kj/kg oC.. What is the maximum temperature to which the cold fluid can be heated in a parallel flow and in a counter flow heat exchanger?a)80°C in both parallel flow and counter flowb)40°C in parallel flow and 80°C in counter flowc)40°C in parallel flow and 50°C in counter flowd)50°C in both parallel flow and counter flowCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Chemical Engineering 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In a double-pipe heat exchanger, the cold fluid is water with inlet temperature of 20oC and mass flow rate of 20 kg/s and the hot fluid which also has water inlet temperature of 80oC and mass flow rate of 10 kg/s. For water cp = 4.2kj/kg oC.. What is the maximum temperature to which the cold fluid can be heated in a parallel flow and in a counter flow heat exchanger?a)80°C in both parallel flow and counter flowb)40°C in parallel flow and 80°C in counter flowc)40°C in parallel flow and 50°C in counter flowd)50°C in both parallel flow and counter flowCorrect answer is option 'C'. Can you explain this answer?.
Solutions for In a double-pipe heat exchanger, the cold fluid is water with inlet temperature of 20oC and mass flow rate of 20 kg/s and the hot fluid which also has water inlet temperature of 80oC and mass flow rate of 10 kg/s. For water cp = 4.2kj/kg oC.. What is the maximum temperature to which the cold fluid can be heated in a parallel flow and in a counter flow heat exchanger?a)80°C in both parallel flow and counter flowb)40°C in parallel flow and 80°C in counter flowc)40°C in parallel flow and 50°C in counter flowd)50°C in both parallel flow and counter flowCorrect answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for Chemical Engineering.
Download more important topics, notes, lectures and mock test series for Chemical Engineering Exam by signing up for free.
Here you can find the meaning of In a double-pipe heat exchanger, the cold fluid is water with inlet temperature of 20oC and mass flow rate of 20 kg/s and the hot fluid which also has water inlet temperature of 80oC and mass flow rate of 10 kg/s. For water cp = 4.2kj/kg oC.. What is the maximum temperature to which the cold fluid can be heated in a parallel flow and in a counter flow heat exchanger?a)80°C in both parallel flow and counter flowb)40°C in parallel flow and 80°C in counter flowc)40°C in parallel flow and 50°C in counter flowd)50°C in both parallel flow and counter flowCorrect answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
In a double-pipe heat exchanger, the cold fluid is water with inlet temperature of 20oC and mass flow rate of 20 kg/s and the hot fluid which also has water inlet temperature of 80oC and mass flow rate of 10 kg/s. For water cp = 4.2kj/kg oC.. What is the maximum temperature to which the cold fluid can be heated in a parallel flow and in a counter flow heat exchanger?a)80°C in both parallel flow and counter flowb)40°C in parallel flow and 80°C in counter flowc)40°C in parallel flow and 50°C in counter flowd)50°C in both parallel flow and counter flowCorrect answer is option 'C'. Can you explain this answer?, a detailed solution for In a double-pipe heat exchanger, the cold fluid is water with inlet temperature of 20oC and mass flow rate of 20 kg/s and the hot fluid which also has water inlet temperature of 80oC and mass flow rate of 10 kg/s. For water cp = 4.2kj/kg oC.. What is the maximum temperature to which the cold fluid can be heated in a parallel flow and in a counter flow heat exchanger?a)80°C in both parallel flow and counter flowb)40°C in parallel flow and 80°C in counter flowc)40°C in parallel flow and 50°C in counter flowd)50°C in both parallel flow and counter flowCorrect answer is option 'C'. Can you explain this answer? has been provided alongside types of In a double-pipe heat exchanger, the cold fluid is water with inlet temperature of 20oC and mass flow rate of 20 kg/s and the hot fluid which also has water inlet temperature of 80oC and mass flow rate of 10 kg/s. For water cp = 4.2kj/kg oC.. What is the maximum temperature to which the cold fluid can be heated in a parallel flow and in a counter flow heat exchanger?a)80°C in both parallel flow and counter flowb)40°C in parallel flow and 80°C in counter flowc)40°C in parallel flow and 50°C in counter flowd)50°C in both parallel flow and counter flowCorrect answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice In a double-pipe heat exchanger, the cold fluid is water with inlet temperature of 20oC and mass flow rate of 20 kg/s and the hot fluid which also has water inlet temperature of 80oC and mass flow rate of 10 kg/s. For water cp = 4.2kj/kg oC.. What is the maximum temperature to which the cold fluid can be heated in a parallel flow and in a counter flow heat exchanger?a)80°C in both parallel flow and counter flowb)40°C in parallel flow and 80°C in counter flowc)40°C in parallel flow and 50°C in counter flowd)50°C in both parallel flow and counter flowCorrect answer is option 'C'. Can you explain this answer? tests, examples and also practice Chemical Engineering tests.

|
Explore Courses for Chemical Engineering exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















