Class 10 Exam > Class 10 Questions > If AD and PM are medians of triangles ABC and...
Start Learning for Free
If AD and PM are medians of triangles ABC and PQR, respectively where ΔABC ~ ΔPQR prove that AB/PQ = AD/PM.?
Most Upvoted Answer
If AD and PM are medians of triangles ABC and PQR, respectively where ...
We know if one angle of a triangle is equal to one angle of the other triangle and the sides including these angles are proportional, then the two triangles are similar.
This is referred as SAS criterion for two triangles.
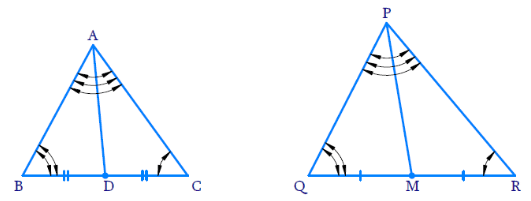
If AD and PM are medians of triangles ABC and PQR, respectively where ΔABC ~ ΔPQR, prove that AB/PQ = AD/PM
Given, ΔABC ∼ ΔPQR
Given, ΔABC ∼ ΔPQR
⇒ ∠ABC = ∠PQR (corresponding angles) --------- (1)
⇒ AB/PQ = BC/QR (corresponding sides)
⇒ AB/PQ = (BC/2) / (QR/2)
⇒ AB/PQ = BD/QM (D and M are mid-points of BC and QR) ------------ (2)
In ΔABD and ΔPQM,
∠ABD = ∠PQM (from 1)
AB/PQ = BD/QM (from 2)
⇒ ΔABD ∼ ΔPQM (SAS criterion)
⇒ AB/PQ = BD/QM = AD/PM (corresponding sides)
⇒ AB/PQ = AD/PM
hence proved.
Community Answer
If AD and PM are medians of triangles ABC and PQR, respectively where ...
**Proof:**
Given, ΔABC ~ ΔPQR
We need to prove AB/PQ = AD/PM
**Step 1:**
Let's first understand the concept of medians.
A median of a triangle is a line segment that connects a vertex of the triangle to the midpoint of the opposite side.
In triangle ABC, AD is a median. So, it passes through the midpoint of BC.
Similarly, in triangle PQR, PM is a median. So, it passes through the midpoint of QR.
**Step 2:**
Since ΔABC ~ ΔPQR, we know that the corresponding sides of the two triangles are proportional.
So, we can write:
AB/PQ = BC/QR
**Step 3:**
Now, let's look at the triangles formed by the medians.
In triangle ABC, let M be the midpoint of AD.
Similarly, in triangle PQR, let N be the midpoint of PM.
**Step 4:**
We know that the medians of a triangle divide it into six smaller triangles of equal area.
So, we can write:
Area(ΔABM) = Area(ΔACM) = 1/6 Area(ΔABC)
Area(ΔPNM) = Area(ΔPQM) = 1/6 Area(ΔPQR)
**Step 5:**
Let's now look at the ratio of the areas of the two triangles ΔABM and ΔPNM.
We know that the corresponding sides of the two triangles are proportional. So, we can write:
AB/PQ = BC/QR = AC/PR
Therefore, we have:
AB/AC = PQ/PR
**Step 6:**
We can now use the formula for the area of a triangle, which is:
Area = 1/2 base × height
In triangle ΔABM, the height is AD and the base is AB.
So, we have:
Area(ΔABM) = 1/2 × AB × AD
Similarly, in triangle ΔPNM, the height is PM and the base is PQ.
So, we have:
Area(ΔPNM) = 1/2 × PQ × PM
**Step 7:**
We can now substitute the values of the areas in terms of the bases and heights and simplify the expression.
We get:
AB/PQ = (2 × Area(ΔABM))/AD × (1/2)/(2 × Area(ΔPNM))/PM × (1/2)
AB/PQ = (Area(ΔABM))/AD × (1/Area(ΔPNM))/PM
AB/PQ = AD/PM
Hence, proved.
Therefore, AB/PQ = AD/PM.
Given, ΔABC ~ ΔPQR
We need to prove AB/PQ = AD/PM
**Step 1:**
Let's first understand the concept of medians.
A median of a triangle is a line segment that connects a vertex of the triangle to the midpoint of the opposite side.
In triangle ABC, AD is a median. So, it passes through the midpoint of BC.
Similarly, in triangle PQR, PM is a median. So, it passes through the midpoint of QR.
**Step 2:**
Since ΔABC ~ ΔPQR, we know that the corresponding sides of the two triangles are proportional.
So, we can write:
AB/PQ = BC/QR
**Step 3:**
Now, let's look at the triangles formed by the medians.
In triangle ABC, let M be the midpoint of AD.
Similarly, in triangle PQR, let N be the midpoint of PM.
**Step 4:**
We know that the medians of a triangle divide it into six smaller triangles of equal area.
So, we can write:
Area(ΔABM) = Area(ΔACM) = 1/6 Area(ΔABC)
Area(ΔPNM) = Area(ΔPQM) = 1/6 Area(ΔPQR)
**Step 5:**
Let's now look at the ratio of the areas of the two triangles ΔABM and ΔPNM.
We know that the corresponding sides of the two triangles are proportional. So, we can write:
AB/PQ = BC/QR = AC/PR
Therefore, we have:
AB/AC = PQ/PR
**Step 6:**
We can now use the formula for the area of a triangle, which is:
Area = 1/2 base × height
In triangle ΔABM, the height is AD and the base is AB.
So, we have:
Area(ΔABM) = 1/2 × AB × AD
Similarly, in triangle ΔPNM, the height is PM and the base is PQ.
So, we have:
Area(ΔPNM) = 1/2 × PQ × PM
**Step 7:**
We can now substitute the values of the areas in terms of the bases and heights and simplify the expression.
We get:
AB/PQ = (2 × Area(ΔABM))/AD × (1/2)/(2 × Area(ΔPNM))/PM × (1/2)
AB/PQ = (Area(ΔABM))/AD × (1/Area(ΔPNM))/PM
AB/PQ = AD/PM
Hence, proved.
Therefore, AB/PQ = AD/PM.

|
Explore Courses for Class 10 exam
|

|
Similar Class 10 Doubts
If AD and PM are medians of triangles ABC and PQR, respectively where ΔABC ~ ΔPQR prove that AB/PQ = AD/PM.?
Question Description
If AD and PM are medians of triangles ABC and PQR, respectively where ΔABC ~ ΔPQR prove that AB/PQ = AD/PM.? for Class 10 2025 is part of Class 10 preparation. The Question and answers have been prepared according to the Class 10 exam syllabus. Information about If AD and PM are medians of triangles ABC and PQR, respectively where ΔABC ~ ΔPQR prove that AB/PQ = AD/PM.? covers all topics & solutions for Class 10 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for If AD and PM are medians of triangles ABC and PQR, respectively where ΔABC ~ ΔPQR prove that AB/PQ = AD/PM.?.
If AD and PM are medians of triangles ABC and PQR, respectively where ΔABC ~ ΔPQR prove that AB/PQ = AD/PM.? for Class 10 2025 is part of Class 10 preparation. The Question and answers have been prepared according to the Class 10 exam syllabus. Information about If AD and PM are medians of triangles ABC and PQR, respectively where ΔABC ~ ΔPQR prove that AB/PQ = AD/PM.? covers all topics & solutions for Class 10 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for If AD and PM are medians of triangles ABC and PQR, respectively where ΔABC ~ ΔPQR prove that AB/PQ = AD/PM.?.
Solutions for If AD and PM are medians of triangles ABC and PQR, respectively where ΔABC ~ ΔPQR prove that AB/PQ = AD/PM.? in English & in Hindi are available as part of our courses for Class 10.
Download more important topics, notes, lectures and mock test series for Class 10 Exam by signing up for free.
Here you can find the meaning of If AD and PM are medians of triangles ABC and PQR, respectively where ΔABC ~ ΔPQR prove that AB/PQ = AD/PM.? defined & explained in the simplest way possible. Besides giving the explanation of
If AD and PM are medians of triangles ABC and PQR, respectively where ΔABC ~ ΔPQR prove that AB/PQ = AD/PM.?, a detailed solution for If AD and PM are medians of triangles ABC and PQR, respectively where ΔABC ~ ΔPQR prove that AB/PQ = AD/PM.? has been provided alongside types of If AD and PM are medians of triangles ABC and PQR, respectively where ΔABC ~ ΔPQR prove that AB/PQ = AD/PM.? theory, EduRev gives you an
ample number of questions to practice If AD and PM are medians of triangles ABC and PQR, respectively where ΔABC ~ ΔPQR prove that AB/PQ = AD/PM.? tests, examples and also practice Class 10 tests.

|
Explore Courses for Class 10 exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























