Electrical Engineering (EE) Exam > Electrical Engineering (EE) Questions > The load-flow solution is always assured in c...
Start Learning for Free
The load-flow solution is always assured in case of
- a)Newton-Raphson method
- b)Gauss-Seidel method
- c)Fast Decoupled method
- d)None of these methods guarantee
Correct answer is option 'A'. Can you explain this answer?
Most Upvoted Answer
The load-flow solution is always assured in case ofa)Newton-Raphson me...
Load Flow Solution and its Methods
The load flow solution, also known as power flow analysis, is a fundamental analysis in power system engineering. It is used to determine the steady-state operating conditions of a power system, including voltage magnitudes, voltage angles, active power flows, and reactive power flows. The load flow solution is essential for optimal power system planning and operation.
There are several methods available to solve the load flow problem, each with its own advantages and limitations. The options provided in the question are Newton-Raphson method, Gauss-Seidel method, and Fast Decoupled method. Let's discuss each method and understand why the correct answer is option 'A' - Newton-Raphson method.
1. Newton-Raphson Method:
The Newton-Raphson method is an iterative numerical method used to solve nonlinear equations. In the context of load flow analysis, it is used to solve the power flow equations. The method utilizes the Jacobian matrix, which represents the partial derivatives of the power flow equations with respect to the voltage magnitudes and angles. The Newton-Raphson method has several advantages, including fast convergence and good numerical stability. It is widely used in power system analysis due to its efficiency and accuracy.
2. Gauss-Seidel Method:
The Gauss-Seidel method is another iterative method used to solve the power flow equations. Unlike the Newton-Raphson method, the Gauss-Seidel method updates the voltage magnitudes and angles sequentially, one at a time. The updated values are used immediately in subsequent calculations, leading to a slower convergence compared to the Newton-Raphson method. However, the Gauss-Seidel method is computationally simpler and requires less memory, making it suitable for small to medium-sized power systems.
3. Fast Decoupled Method:
The Fast Decoupled method is an enhancement of the Gauss-Seidel method that reduces the computational burden by approximating the Jacobian matrix. It decouples the power flow equations into separate equations for voltage magnitudes and angles, simplifying the iterative process. The Fast Decoupled method provides faster convergence compared to the Gauss-Seidel method while maintaining computational efficiency. It is commonly used for large-scale power systems where the Newton-Raphson method may be computationally expensive.
Conclusion:
While all three methods - Newton-Raphson, Gauss-Seidel, and Fast Decoupled - can be used to solve the load flow problem, the Newton-Raphson method is the most reliable and robust. It guarantees convergence to a solution under normal operating conditions and is capable of handling a wide range of power system configurations. The Gauss-Seidel method and Fast Decoupled method may not guarantee convergence or accuracy in all scenarios. Therefore, the correct answer is option 'A' - Newton-Raphson method.
The load flow solution, also known as power flow analysis, is a fundamental analysis in power system engineering. It is used to determine the steady-state operating conditions of a power system, including voltage magnitudes, voltage angles, active power flows, and reactive power flows. The load flow solution is essential for optimal power system planning and operation.
There are several methods available to solve the load flow problem, each with its own advantages and limitations. The options provided in the question are Newton-Raphson method, Gauss-Seidel method, and Fast Decoupled method. Let's discuss each method and understand why the correct answer is option 'A' - Newton-Raphson method.
1. Newton-Raphson Method:
The Newton-Raphson method is an iterative numerical method used to solve nonlinear equations. In the context of load flow analysis, it is used to solve the power flow equations. The method utilizes the Jacobian matrix, which represents the partial derivatives of the power flow equations with respect to the voltage magnitudes and angles. The Newton-Raphson method has several advantages, including fast convergence and good numerical stability. It is widely used in power system analysis due to its efficiency and accuracy.
2. Gauss-Seidel Method:
The Gauss-Seidel method is another iterative method used to solve the power flow equations. Unlike the Newton-Raphson method, the Gauss-Seidel method updates the voltage magnitudes and angles sequentially, one at a time. The updated values are used immediately in subsequent calculations, leading to a slower convergence compared to the Newton-Raphson method. However, the Gauss-Seidel method is computationally simpler and requires less memory, making it suitable for small to medium-sized power systems.
3. Fast Decoupled Method:
The Fast Decoupled method is an enhancement of the Gauss-Seidel method that reduces the computational burden by approximating the Jacobian matrix. It decouples the power flow equations into separate equations for voltage magnitudes and angles, simplifying the iterative process. The Fast Decoupled method provides faster convergence compared to the Gauss-Seidel method while maintaining computational efficiency. It is commonly used for large-scale power systems where the Newton-Raphson method may be computationally expensive.
Conclusion:
While all three methods - Newton-Raphson, Gauss-Seidel, and Fast Decoupled - can be used to solve the load flow problem, the Newton-Raphson method is the most reliable and robust. It guarantees convergence to a solution under normal operating conditions and is capable of handling a wide range of power system configurations. The Gauss-Seidel method and Fast Decoupled method may not guarantee convergence or accuracy in all scenarios. Therefore, the correct answer is option 'A' - Newton-Raphson method.
Free Test
FREE
| Start Free Test |
Community Answer
The load-flow solution is always assured in case ofa)Newton-Raphson me...
Difference between the Gauss-Seidel method and Newton-Raphson method:
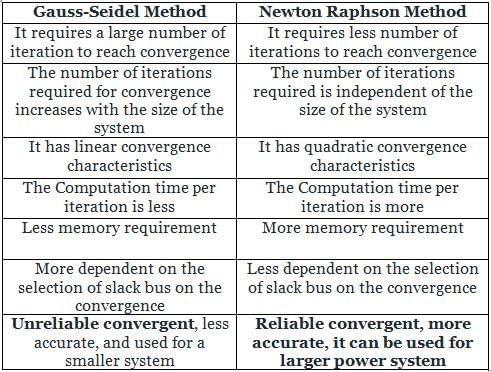
As accuracy is more Newton-Raphson method have assured load flow solution

As accuracy is more Newton-Raphson method have assured load flow solution

|
Explore Courses for Electrical Engineering (EE) exam
|

|
Similar Electrical Engineering (EE) Doubts
The load-flow solution is always assured in case ofa)Newton-Raphson methodb)Gauss-Seidel methodc)Fast Decoupled methodd)None of these methods guaranteeCorrect answer is option 'A'. Can you explain this answer?
Question Description
The load-flow solution is always assured in case ofa)Newton-Raphson methodb)Gauss-Seidel methodc)Fast Decoupled methodd)None of these methods guaranteeCorrect answer is option 'A'. Can you explain this answer? for Electrical Engineering (EE) 2025 is part of Electrical Engineering (EE) preparation. The Question and answers have been prepared according to the Electrical Engineering (EE) exam syllabus. Information about The load-flow solution is always assured in case ofa)Newton-Raphson methodb)Gauss-Seidel methodc)Fast Decoupled methodd)None of these methods guaranteeCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for Electrical Engineering (EE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The load-flow solution is always assured in case ofa)Newton-Raphson methodb)Gauss-Seidel methodc)Fast Decoupled methodd)None of these methods guaranteeCorrect answer is option 'A'. Can you explain this answer?.
The load-flow solution is always assured in case ofa)Newton-Raphson methodb)Gauss-Seidel methodc)Fast Decoupled methodd)None of these methods guaranteeCorrect answer is option 'A'. Can you explain this answer? for Electrical Engineering (EE) 2025 is part of Electrical Engineering (EE) preparation. The Question and answers have been prepared according to the Electrical Engineering (EE) exam syllabus. Information about The load-flow solution is always assured in case ofa)Newton-Raphson methodb)Gauss-Seidel methodc)Fast Decoupled methodd)None of these methods guaranteeCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for Electrical Engineering (EE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The load-flow solution is always assured in case ofa)Newton-Raphson methodb)Gauss-Seidel methodc)Fast Decoupled methodd)None of these methods guaranteeCorrect answer is option 'A'. Can you explain this answer?.
Solutions for The load-flow solution is always assured in case ofa)Newton-Raphson methodb)Gauss-Seidel methodc)Fast Decoupled methodd)None of these methods guaranteeCorrect answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for Electrical Engineering (EE).
Download more important topics, notes, lectures and mock test series for Electrical Engineering (EE) Exam by signing up for free.
Here you can find the meaning of The load-flow solution is always assured in case ofa)Newton-Raphson methodb)Gauss-Seidel methodc)Fast Decoupled methodd)None of these methods guaranteeCorrect answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The load-flow solution is always assured in case ofa)Newton-Raphson methodb)Gauss-Seidel methodc)Fast Decoupled methodd)None of these methods guaranteeCorrect answer is option 'A'. Can you explain this answer?, a detailed solution for The load-flow solution is always assured in case ofa)Newton-Raphson methodb)Gauss-Seidel methodc)Fast Decoupled methodd)None of these methods guaranteeCorrect answer is option 'A'. Can you explain this answer? has been provided alongside types of The load-flow solution is always assured in case ofa)Newton-Raphson methodb)Gauss-Seidel methodc)Fast Decoupled methodd)None of these methods guaranteeCorrect answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The load-flow solution is always assured in case ofa)Newton-Raphson methodb)Gauss-Seidel methodc)Fast Decoupled methodd)None of these methods guaranteeCorrect answer is option 'A'. Can you explain this answer? tests, examples and also practice Electrical Engineering (EE) tests.

|
Explore Courses for Electrical Engineering (EE) exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


























