Civil Engineering (CE) Exam > Civil Engineering (CE) Questions > The flow of glycerine (kinematic viscosity, ...
Start Learning for Free
The flow of glycerine (kinematic viscosity, vg = 5 × 10-4 m2/s) in an open channel is to be modelled in a laboratory flume using water (vw = 10-6 m2/s) as the flowing fluid. If both gravity and viscosity are important, what should be the length scale (i.e. ratio of prototype to model dimensions) for maintaining dynamic similarity? (Answer up to the nearest integer)
Correct answer is '63'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Most Upvoted Answer
The flow of glycerine (kinematic viscosity, vg = 5 × 10-4 m2/s) in an...
From Froud model law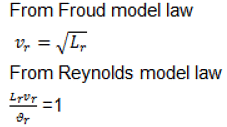
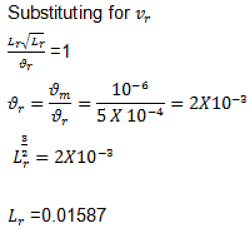
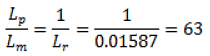
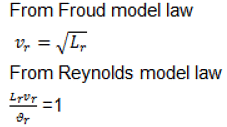
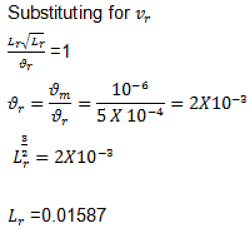
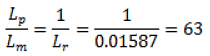
Free Test
FREE
| Start Free Test |
Community Answer
The flow of glycerine (kinematic viscosity, vg = 5 × 10-4 m2/s) in an...
Introduction:
In the laboratory flume, the flow of glycerine is being modeled using water as the flowing fluid. In order to ensure dynamic similarity between the prototype (glycerine) and the model (water) flow, it is necessary to determine the appropriate length scale. If both gravity and viscosity are important, the length scale can be calculated based on the kinematic viscosity of the fluids.
Dynamic Similarity:
Dynamic similarity is achieved when the forces acting on the prototype and the model are proportional. In fluid dynamics, Reynolds number is commonly used to determine dynamic similarity. Reynolds number (Re) is the ratio of inertial forces to viscous forces in a flowing fluid.
Reynolds Number:
The Reynolds number can be calculated using the formula: Re = (ρ * V * L) / μ, where ρ is the density of the fluid, V is the velocity of the flow, L is the characteristic length, and μ is the viscosity of the fluid.
Length Scale:
To maintain dynamic similarity between the prototype (glycerine) and the model (water) flow, the Reynolds number of the prototype and model should be equal. Since the density and velocity of the fluids are not given, we can assume that they are the same for both fluids.
We can set up the equation for the Reynolds number as follows:
(ρ * V * Lg) / μg = (ρ * V * Lw) / μw
Simplifying the equation, we get:
(μw/μg) = (Lg/Lw)
Since the kinematic viscosity is given for both glycerine (vg) and water (vw), we can substitute the values:
(5 × 10^-4) / (10^-6) = (Lg/Lw)
Simplifying further, we get:
Lg/Lw = 500
Calculation of Length Scale:
To determine the length scale (Lg/Lw), we need to find the ratio of the prototype (glycerine) dimension to the model (water) dimension.
Since the question does not provide any specific dimensions for the prototype and model, we can assume that the length scale is the same for all dimensions (length, width, and height).
Therefore, Lg/Lw = 500 can be written as:
Lg = 500 * Lw
To find the length scale, we need to find a value for which Lg is an integer. By trial and error, we can find that Lg = 500 and Lw = 8 will result in an integer value for Lg.
Therefore, the length scale (Lg/Lw) for maintaining dynamic similarity is 500/8 ≈ 63.
In the laboratory flume, the flow of glycerine is being modeled using water as the flowing fluid. In order to ensure dynamic similarity between the prototype (glycerine) and the model (water) flow, it is necessary to determine the appropriate length scale. If both gravity and viscosity are important, the length scale can be calculated based on the kinematic viscosity of the fluids.
Dynamic Similarity:
Dynamic similarity is achieved when the forces acting on the prototype and the model are proportional. In fluid dynamics, Reynolds number is commonly used to determine dynamic similarity. Reynolds number (Re) is the ratio of inertial forces to viscous forces in a flowing fluid.
Reynolds Number:
The Reynolds number can be calculated using the formula: Re = (ρ * V * L) / μ, where ρ is the density of the fluid, V is the velocity of the flow, L is the characteristic length, and μ is the viscosity of the fluid.
Length Scale:
To maintain dynamic similarity between the prototype (glycerine) and the model (water) flow, the Reynolds number of the prototype and model should be equal. Since the density and velocity of the fluids are not given, we can assume that they are the same for both fluids.
We can set up the equation for the Reynolds number as follows:
(ρ * V * Lg) / μg = (ρ * V * Lw) / μw
Simplifying the equation, we get:
(μw/μg) = (Lg/Lw)
Since the kinematic viscosity is given for both glycerine (vg) and water (vw), we can substitute the values:
(5 × 10^-4) / (10^-6) = (Lg/Lw)
Simplifying further, we get:
Lg/Lw = 500
Calculation of Length Scale:
To determine the length scale (Lg/Lw), we need to find the ratio of the prototype (glycerine) dimension to the model (water) dimension.
Since the question does not provide any specific dimensions for the prototype and model, we can assume that the length scale is the same for all dimensions (length, width, and height).
Therefore, Lg/Lw = 500 can be written as:
Lg = 500 * Lw
To find the length scale, we need to find a value for which Lg is an integer. By trial and error, we can find that Lg = 500 and Lw = 8 will result in an integer value for Lg.
Therefore, the length scale (Lg/Lw) for maintaining dynamic similarity is 500/8 ≈ 63.
Attention Civil Engineering (CE) Students!
To make sure you are not studying endlessly, EduRev has designed Civil Engineering (CE) study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Civil Engineering (CE).

|
Explore Courses for Civil Engineering (CE) exam
|

|
Similar Civil Engineering (CE) Doubts
The flow of glycerine (kinematic viscosity, vg = 5 × 10-4 m2/s) in an open channel is to be modelled in a laboratory flume using water (vw = 10-6 m2/s) as the flowing fluid. If both gravity and viscosity are important, what should be the length scale (i.e. ratio of prototype to model dimensions) for maintaining dynamic similarity? (Answer up to the nearest integer)Correct answer is '63'. Can you explain this answer?
Question Description
The flow of glycerine (kinematic viscosity, vg = 5 × 10-4 m2/s) in an open channel is to be modelled in a laboratory flume using water (vw = 10-6 m2/s) as the flowing fluid. If both gravity and viscosity are important, what should be the length scale (i.e. ratio of prototype to model dimensions) for maintaining dynamic similarity? (Answer up to the nearest integer)Correct answer is '63'. Can you explain this answer? for Civil Engineering (CE) 2024 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about The flow of glycerine (kinematic viscosity, vg = 5 × 10-4 m2/s) in an open channel is to be modelled in a laboratory flume using water (vw = 10-6 m2/s) as the flowing fluid. If both gravity and viscosity are important, what should be the length scale (i.e. ratio of prototype to model dimensions) for maintaining dynamic similarity? (Answer up to the nearest integer)Correct answer is '63'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The flow of glycerine (kinematic viscosity, vg = 5 × 10-4 m2/s) in an open channel is to be modelled in a laboratory flume using water (vw = 10-6 m2/s) as the flowing fluid. If both gravity and viscosity are important, what should be the length scale (i.e. ratio of prototype to model dimensions) for maintaining dynamic similarity? (Answer up to the nearest integer)Correct answer is '63'. Can you explain this answer?.
The flow of glycerine (kinematic viscosity, vg = 5 × 10-4 m2/s) in an open channel is to be modelled in a laboratory flume using water (vw = 10-6 m2/s) as the flowing fluid. If both gravity and viscosity are important, what should be the length scale (i.e. ratio of prototype to model dimensions) for maintaining dynamic similarity? (Answer up to the nearest integer)Correct answer is '63'. Can you explain this answer? for Civil Engineering (CE) 2024 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about The flow of glycerine (kinematic viscosity, vg = 5 × 10-4 m2/s) in an open channel is to be modelled in a laboratory flume using water (vw = 10-6 m2/s) as the flowing fluid. If both gravity and viscosity are important, what should be the length scale (i.e. ratio of prototype to model dimensions) for maintaining dynamic similarity? (Answer up to the nearest integer)Correct answer is '63'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The flow of glycerine (kinematic viscosity, vg = 5 × 10-4 m2/s) in an open channel is to be modelled in a laboratory flume using water (vw = 10-6 m2/s) as the flowing fluid. If both gravity and viscosity are important, what should be the length scale (i.e. ratio of prototype to model dimensions) for maintaining dynamic similarity? (Answer up to the nearest integer)Correct answer is '63'. Can you explain this answer?.
Solutions for The flow of glycerine (kinematic viscosity, vg = 5 × 10-4 m2/s) in an open channel is to be modelled in a laboratory flume using water (vw = 10-6 m2/s) as the flowing fluid. If both gravity and viscosity are important, what should be the length scale (i.e. ratio of prototype to model dimensions) for maintaining dynamic similarity? (Answer up to the nearest integer)Correct answer is '63'. Can you explain this answer? in English & in Hindi are available as part of our courses for Civil Engineering (CE).
Download more important topics, notes, lectures and mock test series for Civil Engineering (CE) Exam by signing up for free.
Here you can find the meaning of The flow of glycerine (kinematic viscosity, vg = 5 × 10-4 m2/s) in an open channel is to be modelled in a laboratory flume using water (vw = 10-6 m2/s) as the flowing fluid. If both gravity and viscosity are important, what should be the length scale (i.e. ratio of prototype to model dimensions) for maintaining dynamic similarity? (Answer up to the nearest integer)Correct answer is '63'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The flow of glycerine (kinematic viscosity, vg = 5 × 10-4 m2/s) in an open channel is to be modelled in a laboratory flume using water (vw = 10-6 m2/s) as the flowing fluid. If both gravity and viscosity are important, what should be the length scale (i.e. ratio of prototype to model dimensions) for maintaining dynamic similarity? (Answer up to the nearest integer)Correct answer is '63'. Can you explain this answer?, a detailed solution for The flow of glycerine (kinematic viscosity, vg = 5 × 10-4 m2/s) in an open channel is to be modelled in a laboratory flume using water (vw = 10-6 m2/s) as the flowing fluid. If both gravity and viscosity are important, what should be the length scale (i.e. ratio of prototype to model dimensions) for maintaining dynamic similarity? (Answer up to the nearest integer)Correct answer is '63'. Can you explain this answer? has been provided alongside types of The flow of glycerine (kinematic viscosity, vg = 5 × 10-4 m2/s) in an open channel is to be modelled in a laboratory flume using water (vw = 10-6 m2/s) as the flowing fluid. If both gravity and viscosity are important, what should be the length scale (i.e. ratio of prototype to model dimensions) for maintaining dynamic similarity? (Answer up to the nearest integer)Correct answer is '63'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The flow of glycerine (kinematic viscosity, vg = 5 × 10-4 m2/s) in an open channel is to be modelled in a laboratory flume using water (vw = 10-6 m2/s) as the flowing fluid. If both gravity and viscosity are important, what should be the length scale (i.e. ratio of prototype to model dimensions) for maintaining dynamic similarity? (Answer up to the nearest integer)Correct answer is '63'. Can you explain this answer? tests, examples and also practice Civil Engineering (CE) tests.

|
Explore Courses for Civil Engineering (CE) exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























