**Basic Proportionality Theorem: An Explanation**

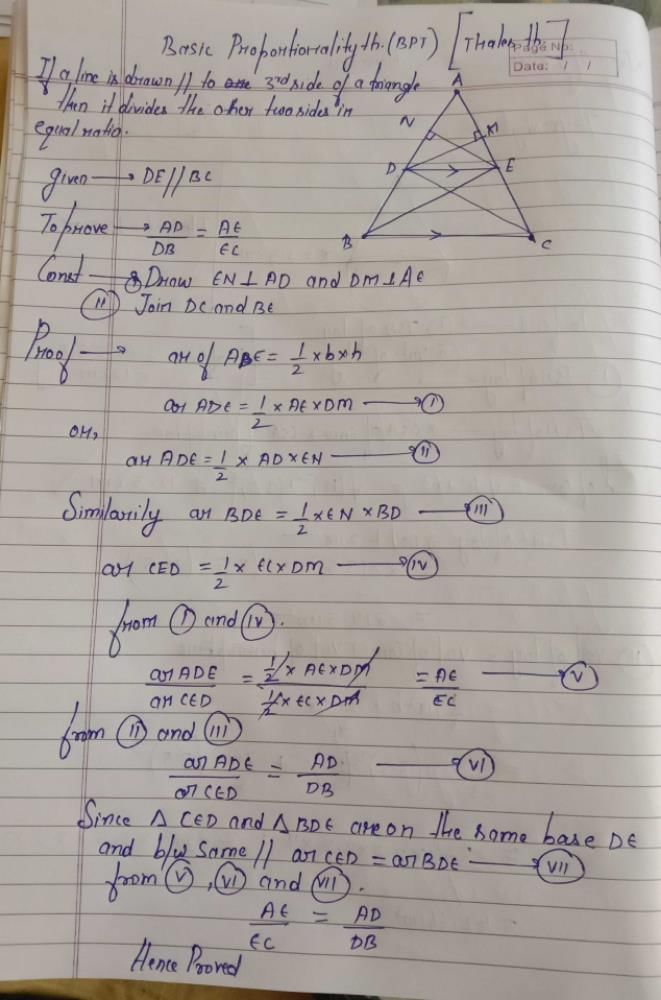
The Basic Proportionality Theorem, also known as the Thales' Theorem or the Intercept Theorem, is a fundamental geometric concept that relates the lengths of intersecting line segments within a triangle. It states that if a line is drawn parallel to one side of a triangle, it will intersect the other two sides in two distinct points, dividing those sides proportionally.

**Understanding the Theorem**

To better understand the Basic Proportionality Theorem, let's consider a triangle ABC and a line parallel to side BC passing through point A. This line intersects side AB at point D and side AC at point E. According to the theorem, the ratio of the lengths of the line segments AD and DB will be equal to the ratio of the lengths of the line segments AE and EC. In mathematical terms, this can be expressed as:

AD/DB = AE/EC

**Proof of the Theorem**

To prove the Basic Proportionality Theorem, we can use similar triangles. By drawing a line segment from point D to point E, we create two triangles: ADE and ABC. Since the line segment DE is parallel to side BC, we have corresponding angles that are equal. Therefore, triangle ADE is similar to triangle ABC.

Now, let's denote the lengths of the line segments as follows:
AD = x
DB = y
AE = p
EC = q

Since triangle ADE is similar to triangle ABC, we can write the following ratios:
AD/AB = DE/BC
x/(x + y) = p/(p + q)

Cross multiplying the equation gives us:
x(p + q) = p(x + y)

Expanding and rearranging the equation gives us:
px + qx = px + py

Canceling out the common terms, we are left with:
qx = py

Dividing both sides by y gives us:
q/y = p/x

Thus, we have proved that AD/DB = AE/EC, which verifies the Basic Proportionality Theorem.

**Application of the Theorem**

The Basic Proportionality Theorem finds applications in various fields, including physics, engineering, and architecture. It helps in solving problems involving similar triangles and allows us to determine unknown lengths or proportions within a triangle. Additionally, it is used to establish the concept of parallel lines and their relationship with intersecting line segments.

In conclusion, the Basic Proportionality Theorem provides a fundamental understanding of the relationship between intersecting line segments within a triangle. By drawing a line parallel to one side of the triangle, we can determine the proportional lengths of the line segments intersecting the other two sides. This theorem is widely used in various mathematical and practical applications and serves as a building block for more complex geometric concepts.