NEET Exam > NEET Questions > A fluid is in streamline flow across a horizo...
Start Learning for Free
A fluid is in streamline flow across a horizontal pipe of variable area of cross section. For this which of the following statements is correct? [NEET Kar. 2013]
- a)The velocity is minimum at the narrowest part of the pipe and the pressure is minimum at the widest part of the pipe
- b)The velocity is maximum at the narrowest part of the pipe and pressure is maximum at the widest part of the pipe
- c)Velocity and pressure both are maximum at the narrowest part of the pipe
- d)Velocity and pressure both are maximum at the widest part of the pipe
Correct answer is option 'B'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A fluid is in streamline flow across a horizontal pipe of variable are...
According to Bernoulli’s theorem,
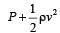= constant and Av = constantIf A is minimum, v is maximum, P is minimum.
Most Upvoted Answer
A fluid is in streamline flow across a horizontal pipe of variable are...
Yes, I try to explain , According to continuity equation that is based on mass reservation
(AV)enter =(AV} which come out .
so,greater the area lesser the velocity .
According to,
bernoulli's equation
P+1dv²/2 +dgh =constant .
so, by above conclusion we know that on increasing the area, velocity decreases due to decrease in velocity in bernoulli's equation to keep the equation constant we have to increase pressure.
(AV)enter =(AV} which come out .
so,greater the area lesser the velocity .
According to,
bernoulli's equation
P+1dv²/2 +dgh =constant .
so, by above conclusion we know that on increasing the area, velocity decreases due to decrease in velocity in bernoulli's equation to keep the equation constant we have to increase pressure.
Free Test
| FREE | Start Free Test |
Community Answer
A fluid is in streamline flow across a horizontal pipe of variable are...
Streamline flow in a horizontal pipe of variable cross-sectional area
In streamline flow, the fluid particles move in such a way that their paths do not cross each other. This means that the velocity of the fluid remains constant along any streamline. When a fluid flows through a pipe of variable cross-sectional area, the velocity and pressure of the fluid change.
Understanding the correct statement
The correct statement is option B: The velocity is maximum at the narrowest part of the pipe and the pressure is maximum at the widest part of the pipe. Let's understand why this statement is correct.
Velocity and cross-sectional area relationship
According to the principle of continuity, the volume flow rate of an incompressible fluid remains constant along a streamline. Mathematically, it can be expressed as:
A1v1 = A2v2
Where A1 and A2 are the cross-sectional areas of the pipe at two different points, and v1 and v2 are the velocities of the fluid at those points.
Velocity and cross-sectional area relationship in a narrowing pipe
When the pipe narrows, the cross-sectional area decreases. According to the principle of continuity, if the cross-sectional area decreases, the velocity of the fluid must increase to maintain a constant volume flow rate. This means that the velocity is maximum at the narrowest part of the pipe.
Pressure and cross-sectional area relationship
According to Bernoulli's principle, as the velocity of a fluid increases, its pressure decreases. This can be expressed as:
P1 + (1/2)ρv1^2 + ρgh1 = P2 + (1/2)ρv2^2 + ρgh2
Where P1 and P2 are the pressures at two different points, ρ is the density of the fluid, v1 and v2 are the velocities at those points, g is the acceleration due to gravity, and h1 and h2 are the heights at those points.
Pressure and cross-sectional area relationship in a narrowing pipe
When the pipe narrows, the velocity of the fluid increases. According to Bernoulli's principle, if the velocity increases, the pressure decreases. Therefore, the pressure is maximum at the widest part of the pipe.
Conclusion
In conclusion, in a horizontal pipe of variable cross-sectional area, the velocity is maximum at the narrowest part of the pipe, and the pressure is maximum at the widest part of the pipe. This is because of the relationship between velocity, cross-sectional area, and pressure in a fluid flow.
In streamline flow, the fluid particles move in such a way that their paths do not cross each other. This means that the velocity of the fluid remains constant along any streamline. When a fluid flows through a pipe of variable cross-sectional area, the velocity and pressure of the fluid change.
Understanding the correct statement
The correct statement is option B: The velocity is maximum at the narrowest part of the pipe and the pressure is maximum at the widest part of the pipe. Let's understand why this statement is correct.
Velocity and cross-sectional area relationship
According to the principle of continuity, the volume flow rate of an incompressible fluid remains constant along a streamline. Mathematically, it can be expressed as:
A1v1 = A2v2
Where A1 and A2 are the cross-sectional areas of the pipe at two different points, and v1 and v2 are the velocities of the fluid at those points.
Velocity and cross-sectional area relationship in a narrowing pipe
When the pipe narrows, the cross-sectional area decreases. According to the principle of continuity, if the cross-sectional area decreases, the velocity of the fluid must increase to maintain a constant volume flow rate. This means that the velocity is maximum at the narrowest part of the pipe.
Pressure and cross-sectional area relationship
According to Bernoulli's principle, as the velocity of a fluid increases, its pressure decreases. This can be expressed as:
P1 + (1/2)ρv1^2 + ρgh1 = P2 + (1/2)ρv2^2 + ρgh2
Where P1 and P2 are the pressures at two different points, ρ is the density of the fluid, v1 and v2 are the velocities at those points, g is the acceleration due to gravity, and h1 and h2 are the heights at those points.
Pressure and cross-sectional area relationship in a narrowing pipe
When the pipe narrows, the velocity of the fluid increases. According to Bernoulli's principle, if the velocity increases, the pressure decreases. Therefore, the pressure is maximum at the widest part of the pipe.
Conclusion
In conclusion, in a horizontal pipe of variable cross-sectional area, the velocity is maximum at the narrowest part of the pipe, and the pressure is maximum at the widest part of the pipe. This is because of the relationship between velocity, cross-sectional area, and pressure in a fluid flow.
Attention NEET Students!
To make sure you are not studying endlessly, EduRev has designed NEET study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in NEET.
|
Explore Courses for NEET exam
|
|
Similar NEET Doubts
Top Courses for NEETView all
A fluid is in streamline flow across a horizontal pipe of variable area of cross section. For this which of the following statements is correct? [NEET Kar. 2013]a)The velocity is minimum at the narrowest part of the pipe and the pressure is minimum at the widest part of the pipeb)The velocity is maximum at the narrowest part of the pipe and pressure is maximum at the widest part of the pipec)Velocity and pressure both are maximum at the narrowest part of the piped)Velocity and pressure both are maximum at the widest part of the pipeCorrect answer is option 'B'. Can you explain this answer?
Question Description
A fluid is in streamline flow across a horizontal pipe of variable area of cross section. For this which of the following statements is correct? [NEET Kar. 2013]a)The velocity is minimum at the narrowest part of the pipe and the pressure is minimum at the widest part of the pipeb)The velocity is maximum at the narrowest part of the pipe and pressure is maximum at the widest part of the pipec)Velocity and pressure both are maximum at the narrowest part of the piped)Velocity and pressure both are maximum at the widest part of the pipeCorrect answer is option 'B'. Can you explain this answer? for NEET 2024 is part of NEET preparation. The Question and answers have been prepared according to the NEET exam syllabus. Information about A fluid is in streamline flow across a horizontal pipe of variable area of cross section. For this which of the following statements is correct? [NEET Kar. 2013]a)The velocity is minimum at the narrowest part of the pipe and the pressure is minimum at the widest part of the pipeb)The velocity is maximum at the narrowest part of the pipe and pressure is maximum at the widest part of the pipec)Velocity and pressure both are maximum at the narrowest part of the piped)Velocity and pressure both are maximum at the widest part of the pipeCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for NEET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A fluid is in streamline flow across a horizontal pipe of variable area of cross section. For this which of the following statements is correct? [NEET Kar. 2013]a)The velocity is minimum at the narrowest part of the pipe and the pressure is minimum at the widest part of the pipeb)The velocity is maximum at the narrowest part of the pipe and pressure is maximum at the widest part of the pipec)Velocity and pressure both are maximum at the narrowest part of the piped)Velocity and pressure both are maximum at the widest part of the pipeCorrect answer is option 'B'. Can you explain this answer?.
A fluid is in streamline flow across a horizontal pipe of variable area of cross section. For this which of the following statements is correct? [NEET Kar. 2013]a)The velocity is minimum at the narrowest part of the pipe and the pressure is minimum at the widest part of the pipeb)The velocity is maximum at the narrowest part of the pipe and pressure is maximum at the widest part of the pipec)Velocity and pressure both are maximum at the narrowest part of the piped)Velocity and pressure both are maximum at the widest part of the pipeCorrect answer is option 'B'. Can you explain this answer? for NEET 2024 is part of NEET preparation. The Question and answers have been prepared according to the NEET exam syllabus. Information about A fluid is in streamline flow across a horizontal pipe of variable area of cross section. For this which of the following statements is correct? [NEET Kar. 2013]a)The velocity is minimum at the narrowest part of the pipe and the pressure is minimum at the widest part of the pipeb)The velocity is maximum at the narrowest part of the pipe and pressure is maximum at the widest part of the pipec)Velocity and pressure both are maximum at the narrowest part of the piped)Velocity and pressure both are maximum at the widest part of the pipeCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for NEET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A fluid is in streamline flow across a horizontal pipe of variable area of cross section. For this which of the following statements is correct? [NEET Kar. 2013]a)The velocity is minimum at the narrowest part of the pipe and the pressure is minimum at the widest part of the pipeb)The velocity is maximum at the narrowest part of the pipe and pressure is maximum at the widest part of the pipec)Velocity and pressure both are maximum at the narrowest part of the piped)Velocity and pressure both are maximum at the widest part of the pipeCorrect answer is option 'B'. Can you explain this answer?.
Solutions for A fluid is in streamline flow across a horizontal pipe of variable area of cross section. For this which of the following statements is correct? [NEET Kar. 2013]a)The velocity is minimum at the narrowest part of the pipe and the pressure is minimum at the widest part of the pipeb)The velocity is maximum at the narrowest part of the pipe and pressure is maximum at the widest part of the pipec)Velocity and pressure both are maximum at the narrowest part of the piped)Velocity and pressure both are maximum at the widest part of the pipeCorrect answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for NEET.
Download more important topics, notes, lectures and mock test series for NEET Exam by signing up for free.
Here you can find the meaning of A fluid is in streamline flow across a horizontal pipe of variable area of cross section. For this which of the following statements is correct? [NEET Kar. 2013]a)The velocity is minimum at the narrowest part of the pipe and the pressure is minimum at the widest part of the pipeb)The velocity is maximum at the narrowest part of the pipe and pressure is maximum at the widest part of the pipec)Velocity and pressure both are maximum at the narrowest part of the piped)Velocity and pressure both are maximum at the widest part of the pipeCorrect answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A fluid is in streamline flow across a horizontal pipe of variable area of cross section. For this which of the following statements is correct? [NEET Kar. 2013]a)The velocity is minimum at the narrowest part of the pipe and the pressure is minimum at the widest part of the pipeb)The velocity is maximum at the narrowest part of the pipe and pressure is maximum at the widest part of the pipec)Velocity and pressure both are maximum at the narrowest part of the piped)Velocity and pressure both are maximum at the widest part of the pipeCorrect answer is option 'B'. Can you explain this answer?, a detailed solution for A fluid is in streamline flow across a horizontal pipe of variable area of cross section. For this which of the following statements is correct? [NEET Kar. 2013]a)The velocity is minimum at the narrowest part of the pipe and the pressure is minimum at the widest part of the pipeb)The velocity is maximum at the narrowest part of the pipe and pressure is maximum at the widest part of the pipec)Velocity and pressure both are maximum at the narrowest part of the piped)Velocity and pressure both are maximum at the widest part of the pipeCorrect answer is option 'B'. Can you explain this answer? has been provided alongside types of A fluid is in streamline flow across a horizontal pipe of variable area of cross section. For this which of the following statements is correct? [NEET Kar. 2013]a)The velocity is minimum at the narrowest part of the pipe and the pressure is minimum at the widest part of the pipeb)The velocity is maximum at the narrowest part of the pipe and pressure is maximum at the widest part of the pipec)Velocity and pressure both are maximum at the narrowest part of the piped)Velocity and pressure both are maximum at the widest part of the pipeCorrect answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A fluid is in streamline flow across a horizontal pipe of variable area of cross section. For this which of the following statements is correct? [NEET Kar. 2013]a)The velocity is minimum at the narrowest part of the pipe and the pressure is minimum at the widest part of the pipeb)The velocity is maximum at the narrowest part of the pipe and pressure is maximum at the widest part of the pipec)Velocity and pressure both are maximum at the narrowest part of the piped)Velocity and pressure both are maximum at the widest part of the pipeCorrect answer is option 'B'. Can you explain this answer? tests, examples and also practice NEET tests.
|
|
Explore Courses for NEET exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup