NEET Exam > NEET Questions > Consider a sphere of radius R having charge Q...
Start Learning for Free
Consider a sphere of radius R having charge Q uniformly distributed inside it at what minimum distance from its surface the electric potential is half of the electric potential as its centre?
Verified Answer
Consider a sphere of radius R having charge Q uniformly distributed in...
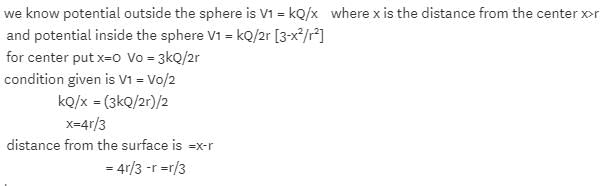
 This question is part of UPSC exam. View all NEET courses
This question is part of UPSC exam. View all NEET courses
Most Upvoted Answer
Consider a sphere of radius R having charge Q uniformly distributed in...
Electric Potential of a Charged Sphere
The electric potential at a point is defined as the amount of work done in bringing a unit positive charge from infinity to that point, divided by the magnitude of the charge. In the case of a charged sphere, the electric potential is given by the equation:
V = kQ/r
where V is the electric potential, k is the electrostatic constant, Q is the charge of the sphere, and r is the distance from the center of the sphere.
Determining the Minimum Distance
To find the minimum distance from the surface of the sphere where the electric potential is half of the potential at the center, we need to set up an equation and solve for r.
Let V_c be the electric potential at the center of the sphere, which is equal to V_c = kQ/R, where R is the radius of the sphere. The electric potential at a distance r from the center is given by V = kQ/r.
We can set up the equation:
V = 1/2 * V_c
Substituting the values, we get:
kQ/r = 1/2 * kQ/R
Simplifying the Equation
To simplify the equation, we can cancel out the kQ terms:
1/r = 1/2R
Cross-multiplying, we have:
2R = r
Therefore, the minimum distance from the surface of the sphere where the electric potential is half of the potential at the center is equal to twice the radius of the sphere.
Summary
The minimum distance from the surface of a charged sphere, where the electric potential is half of the potential at the center, is equal to twice the radius of the sphere. This can be derived by setting up an equation using the electric potential formula and solving for the distance. The equation simplifies to 2R = r, where R is the radius of the sphere.
The electric potential at a point is defined as the amount of work done in bringing a unit positive charge from infinity to that point, divided by the magnitude of the charge. In the case of a charged sphere, the electric potential is given by the equation:
V = kQ/r
where V is the electric potential, k is the electrostatic constant, Q is the charge of the sphere, and r is the distance from the center of the sphere.
Determining the Minimum Distance
To find the minimum distance from the surface of the sphere where the electric potential is half of the potential at the center, we need to set up an equation and solve for r.
Let V_c be the electric potential at the center of the sphere, which is equal to V_c = kQ/R, where R is the radius of the sphere. The electric potential at a distance r from the center is given by V = kQ/r.
We can set up the equation:
V = 1/2 * V_c
Substituting the values, we get:
kQ/r = 1/2 * kQ/R
Simplifying the Equation
To simplify the equation, we can cancel out the kQ terms:
1/r = 1/2R
Cross-multiplying, we have:
2R = r
Therefore, the minimum distance from the surface of the sphere where the electric potential is half of the potential at the center is equal to twice the radius of the sphere.
Summary
The minimum distance from the surface of a charged sphere, where the electric potential is half of the potential at the center, is equal to twice the radius of the sphere. This can be derived by setting up an equation using the electric potential formula and solving for the distance. The equation simplifies to 2R = r, where R is the radius of the sphere.
Attention NEET Students!
To make sure you are not studying endlessly, EduRev has designed NEET study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in NEET.

|
Explore Courses for NEET exam
|

|
Similar NEET Doubts
Consider a sphere of radius R having charge Q uniformly distributed inside it at what minimum distance from its surface the electric potential is half of the electric potential as its centre?
Question Description
Consider a sphere of radius R having charge Q uniformly distributed inside it at what minimum distance from its surface the electric potential is half of the electric potential as its centre? for NEET 2024 is part of NEET preparation. The Question and answers have been prepared according to the NEET exam syllabus. Information about Consider a sphere of radius R having charge Q uniformly distributed inside it at what minimum distance from its surface the electric potential is half of the electric potential as its centre? covers all topics & solutions for NEET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Consider a sphere of radius R having charge Q uniformly distributed inside it at what minimum distance from its surface the electric potential is half of the electric potential as its centre?.
Consider a sphere of radius R having charge Q uniformly distributed inside it at what minimum distance from its surface the electric potential is half of the electric potential as its centre? for NEET 2024 is part of NEET preparation. The Question and answers have been prepared according to the NEET exam syllabus. Information about Consider a sphere of radius R having charge Q uniformly distributed inside it at what minimum distance from its surface the electric potential is half of the electric potential as its centre? covers all topics & solutions for NEET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Consider a sphere of radius R having charge Q uniformly distributed inside it at what minimum distance from its surface the electric potential is half of the electric potential as its centre?.
Solutions for Consider a sphere of radius R having charge Q uniformly distributed inside it at what minimum distance from its surface the electric potential is half of the electric potential as its centre? in English & in Hindi are available as part of our courses for NEET.
Download more important topics, notes, lectures and mock test series for NEET Exam by signing up for free.
Here you can find the meaning of Consider a sphere of radius R having charge Q uniformly distributed inside it at what minimum distance from its surface the electric potential is half of the electric potential as its centre? defined & explained in the simplest way possible. Besides giving the explanation of
Consider a sphere of radius R having charge Q uniformly distributed inside it at what minimum distance from its surface the electric potential is half of the electric potential as its centre?, a detailed solution for Consider a sphere of radius R having charge Q uniformly distributed inside it at what minimum distance from its surface the electric potential is half of the electric potential as its centre? has been provided alongside types of Consider a sphere of radius R having charge Q uniformly distributed inside it at what minimum distance from its surface the electric potential is half of the electric potential as its centre? theory, EduRev gives you an
ample number of questions to practice Consider a sphere of radius R having charge Q uniformly distributed inside it at what minimum distance from its surface the electric potential is half of the electric potential as its centre? tests, examples and also practice NEET tests.

|
Explore Courses for NEET exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.

























