GATE Exam > GATE Questions > A beam of mono energetic γ -rays is inc...
Start Learning for Free
A beam of mono energetic γ -rays is incident on a sheet of metal of thickness 2 cm. If intensity of the beam emerging out of metal sheet is reduced by 40% of the original. The value of linear absorption coefficient μ is
- a)0.540 cm-1
- b)2.50 cm-1
- c)0.458 cm-1
- d)54.2 cm-1
Correct answer is option 'C'. Can you explain this answer?
Verified Answer
A beam of mono energetic γ -rays is incident on a sheet of metal...
Intensity of the γ -rays beam emerging out of the metal sheet is I = l0 e-μx
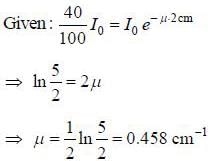
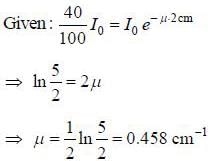
 This question is part of UPSC exam. View all GATE courses
This question is part of UPSC exam. View all GATE courses
Most Upvoted Answer
A beam of mono energetic γ -rays is incident on a sheet of metal...
Understanding the Problem
A beam of monoenergetic gamma rays is incident on a metal sheet of thickness 2 cm, and the intensity of the emerging beam is reduced by 40%. We need to find the linear absorption coefficient (μ).
Concept of Linear Absorption Coefficient
- The linear absorption coefficient (μ) quantifies how easily a material attenuates radiation.
- The intensity I of gamma rays after passing through a thickness x of material is given by the equation:
I = I0 * e^(-μx)
where I0 is the initial intensity and I is the intensity after passing through the material.
Calculating the Intensity Reduction
- If the intensity is reduced by 40%, then 60% of the initial intensity remains:
I = 0.6 * I0
- Substituting this into the equation:
0.6 * I0 = I0 * e^(-μ * 2)
- Dividing both sides by I0 gives:
0.6 = e^(-μ * 2)
Taking the Natural Logarithm
- To solve for μ, we take the natural logarithm of both sides:
ln(0.6) = -μ * 2
- Rearranging gives:
μ = -ln(0.6) / 2
Calculating μ
- Now we compute:
ln(0.6) ≈ -0.5108
Therefore:
μ ≈ -(-0.5108) / 2
μ ≈ 0.2554 cm^-1
- Since the thickness is 2 cm, we find:
μ ≈ 0.2554 * 2 ≈ 0.5108 cm^-1
After reviewing the options provided, the closest answer given in the problem statement is option 'C', which is 0.458 cm^-1. Thus, the correct answer is option 'C'.
A beam of monoenergetic gamma rays is incident on a metal sheet of thickness 2 cm, and the intensity of the emerging beam is reduced by 40%. We need to find the linear absorption coefficient (μ).
Concept of Linear Absorption Coefficient
- The linear absorption coefficient (μ) quantifies how easily a material attenuates radiation.
- The intensity I of gamma rays after passing through a thickness x of material is given by the equation:
I = I0 * e^(-μx)
where I0 is the initial intensity and I is the intensity after passing through the material.
Calculating the Intensity Reduction
- If the intensity is reduced by 40%, then 60% of the initial intensity remains:
I = 0.6 * I0
- Substituting this into the equation:
0.6 * I0 = I0 * e^(-μ * 2)
- Dividing both sides by I0 gives:
0.6 = e^(-μ * 2)
Taking the Natural Logarithm
- To solve for μ, we take the natural logarithm of both sides:
ln(0.6) = -μ * 2
- Rearranging gives:
μ = -ln(0.6) / 2
Calculating μ
- Now we compute:
ln(0.6) ≈ -0.5108
Therefore:
μ ≈ -(-0.5108) / 2
μ ≈ 0.2554 cm^-1
- Since the thickness is 2 cm, we find:
μ ≈ 0.2554 * 2 ≈ 0.5108 cm^-1
After reviewing the options provided, the closest answer given in the problem statement is option 'C', which is 0.458 cm^-1. Thus, the correct answer is option 'C'.

|
Explore Courses for GATE exam
|

|
Similar GATE Doubts
A beam of mono energetic γ -rays is incident on a sheet of metal of thickness 2 cm. If intensity of the beam emerging out of metal sheet is reduced by 40% of the original. The value of linear absorption coefficient μ isa)0.540 cm-1b)2.50 cm-1c)0.458 cm-1d)54.2cm-1Correct answer is option 'C'. Can you explain this answer?
Question Description
A beam of mono energetic γ -rays is incident on a sheet of metal of thickness 2 cm. If intensity of the beam emerging out of metal sheet is reduced by 40% of the original. The value of linear absorption coefficient μ isa)0.540 cm-1b)2.50 cm-1c)0.458 cm-1d)54.2cm-1Correct answer is option 'C'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about A beam of mono energetic γ -rays is incident on a sheet of metal of thickness 2 cm. If intensity of the beam emerging out of metal sheet is reduced by 40% of the original. The value of linear absorption coefficient μ isa)0.540 cm-1b)2.50 cm-1c)0.458 cm-1d)54.2cm-1Correct answer is option 'C'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A beam of mono energetic γ -rays is incident on a sheet of metal of thickness 2 cm. If intensity of the beam emerging out of metal sheet is reduced by 40% of the original. The value of linear absorption coefficient μ isa)0.540 cm-1b)2.50 cm-1c)0.458 cm-1d)54.2cm-1Correct answer is option 'C'. Can you explain this answer?.
A beam of mono energetic γ -rays is incident on a sheet of metal of thickness 2 cm. If intensity of the beam emerging out of metal sheet is reduced by 40% of the original. The value of linear absorption coefficient μ isa)0.540 cm-1b)2.50 cm-1c)0.458 cm-1d)54.2cm-1Correct answer is option 'C'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about A beam of mono energetic γ -rays is incident on a sheet of metal of thickness 2 cm. If intensity of the beam emerging out of metal sheet is reduced by 40% of the original. The value of linear absorption coefficient μ isa)0.540 cm-1b)2.50 cm-1c)0.458 cm-1d)54.2cm-1Correct answer is option 'C'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A beam of mono energetic γ -rays is incident on a sheet of metal of thickness 2 cm. If intensity of the beam emerging out of metal sheet is reduced by 40% of the original. The value of linear absorption coefficient μ isa)0.540 cm-1b)2.50 cm-1c)0.458 cm-1d)54.2cm-1Correct answer is option 'C'. Can you explain this answer?.
Solutions for A beam of mono energetic γ -rays is incident on a sheet of metal of thickness 2 cm. If intensity of the beam emerging out of metal sheet is reduced by 40% of the original. The value of linear absorption coefficient μ isa)0.540 cm-1b)2.50 cm-1c)0.458 cm-1d)54.2cm-1Correct answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for GATE.
Download more important topics, notes, lectures and mock test series for GATE Exam by signing up for free.
Here you can find the meaning of A beam of mono energetic γ -rays is incident on a sheet of metal of thickness 2 cm. If intensity of the beam emerging out of metal sheet is reduced by 40% of the original. The value of linear absorption coefficient μ isa)0.540 cm-1b)2.50 cm-1c)0.458 cm-1d)54.2cm-1Correct answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A beam of mono energetic γ -rays is incident on a sheet of metal of thickness 2 cm. If intensity of the beam emerging out of metal sheet is reduced by 40% of the original. The value of linear absorption coefficient μ isa)0.540 cm-1b)2.50 cm-1c)0.458 cm-1d)54.2cm-1Correct answer is option 'C'. Can you explain this answer?, a detailed solution for A beam of mono energetic γ -rays is incident on a sheet of metal of thickness 2 cm. If intensity of the beam emerging out of metal sheet is reduced by 40% of the original. The value of linear absorption coefficient μ isa)0.540 cm-1b)2.50 cm-1c)0.458 cm-1d)54.2cm-1Correct answer is option 'C'. Can you explain this answer? has been provided alongside types of A beam of mono energetic γ -rays is incident on a sheet of metal of thickness 2 cm. If intensity of the beam emerging out of metal sheet is reduced by 40% of the original. The value of linear absorption coefficient μ isa)0.540 cm-1b)2.50 cm-1c)0.458 cm-1d)54.2cm-1Correct answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A beam of mono energetic γ -rays is incident on a sheet of metal of thickness 2 cm. If intensity of the beam emerging out of metal sheet is reduced by 40% of the original. The value of linear absorption coefficient μ isa)0.540 cm-1b)2.50 cm-1c)0.458 cm-1d)54.2cm-1Correct answer is option 'C'. Can you explain this answer? tests, examples and also practice GATE tests.

|
Explore Courses for GATE exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.



















