NEET Exam > NEET Questions > A mild steel wire of length 2l m cross sectio...
Start Learning for Free
A mild steel wire of length 2l m cross sectional area A m^2 is fixed horizontally between two pillars. A small mass m kg is suspended from mild point of wire. If extension in wire are within elastic limit. Then depression at the midpoint of wire will be.?
Most Upvoted Answer
A mild steel wire of length 2l m cross sectional area A m^2 is fixed h...
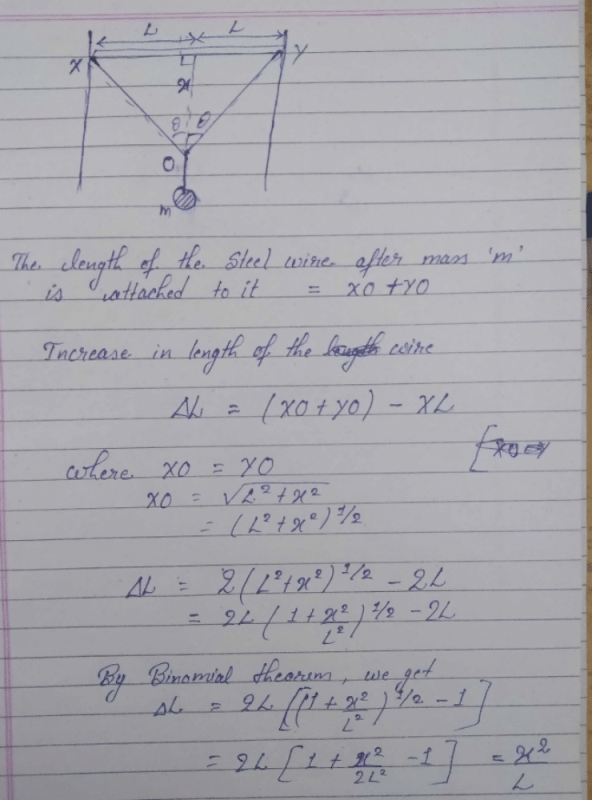
Community Answer
A mild steel wire of length 2l m cross sectional area A m^2 is fixed h...
Depression at the Midpoint of a Mild Steel Wire
To find the depression at the midpoint of a mild steel wire under the influence of a small mass suspended from its midpoint, we need to consider the properties of the material and the forces acting on the wire.
1. Material Properties:
- Mild Steel: The wire is made of mild steel, which is known for its high tensile strength and elasticity.
- Elastic Limit: The wire is assumed to operate within its elastic limit, meaning that it will return to its original shape once the applied forces are removed.
2. Forces Acting on the Wire:
- Tension: The wire is fixed horizontally between two pillars and is under tension due to its own weight and the weight of the suspended mass.
- Compression: The weight of the suspended mass causes compression in the lower half of the wire.
3. Analysis:
- Symmetry: Due to the symmetry of the system, the wire will deform in the shape of a parabola.
- Midpoint Depression: The midpoint of the wire will experience the maximum depression due to the compression forces acting on it.
To calculate the depression at the midpoint, we can use the following steps:
1. Determine the tension in the wire:
- The tension in the wire can be found by considering the equilibrium of forces acting on the wire. The weight of the suspended mass will be balanced by the tension in the wire.
2. Calculate the compression force:
- The weight of the suspended mass will cause a compression force in the lower half of the wire. This force can be calculated using the formula: F = mg, where m is the mass and g is the acceleration due to gravity.
3. Find the depression at the midpoint:
- The depression at the midpoint can be found by considering the geometry of the parabolic shape formed by the wire. The depression at the midpoint will be half of the maximum depression.
4. Substitute values and calculate:
- Substitute the known values, such as the length of the wire, cross-sectional area, mass, and acceleration due to gravity, into the relevant formulas to calculate the tension, compression force, and depression at the midpoint.
Remember to use appropriate unit conversions and consider the given units for each parameter.
In conclusion, to find the depression at the midpoint of a mild steel wire under the influence of a suspended mass, we need to consider the material properties, forces acting on the wire, and the geometry of the wire's deformation. By analyzing these factors and applying relevant formulas, we can calculate the depression at the midpoint.
To find the depression at the midpoint of a mild steel wire under the influence of a small mass suspended from its midpoint, we need to consider the properties of the material and the forces acting on the wire.
1. Material Properties:
- Mild Steel: The wire is made of mild steel, which is known for its high tensile strength and elasticity.
- Elastic Limit: The wire is assumed to operate within its elastic limit, meaning that it will return to its original shape once the applied forces are removed.
2. Forces Acting on the Wire:
- Tension: The wire is fixed horizontally between two pillars and is under tension due to its own weight and the weight of the suspended mass.
- Compression: The weight of the suspended mass causes compression in the lower half of the wire.
3. Analysis:
- Symmetry: Due to the symmetry of the system, the wire will deform in the shape of a parabola.
- Midpoint Depression: The midpoint of the wire will experience the maximum depression due to the compression forces acting on it.
To calculate the depression at the midpoint, we can use the following steps:
1. Determine the tension in the wire:
- The tension in the wire can be found by considering the equilibrium of forces acting on the wire. The weight of the suspended mass will be balanced by the tension in the wire.
2. Calculate the compression force:
- The weight of the suspended mass will cause a compression force in the lower half of the wire. This force can be calculated using the formula: F = mg, where m is the mass and g is the acceleration due to gravity.
3. Find the depression at the midpoint:
- The depression at the midpoint can be found by considering the geometry of the parabolic shape formed by the wire. The depression at the midpoint will be half of the maximum depression.
4. Substitute values and calculate:
- Substitute the known values, such as the length of the wire, cross-sectional area, mass, and acceleration due to gravity, into the relevant formulas to calculate the tension, compression force, and depression at the midpoint.
Remember to use appropriate unit conversions and consider the given units for each parameter.
In conclusion, to find the depression at the midpoint of a mild steel wire under the influence of a suspended mass, we need to consider the material properties, forces acting on the wire, and the geometry of the wire's deformation. By analyzing these factors and applying relevant formulas, we can calculate the depression at the midpoint.
Attention NEET Students!
To make sure you are not studying endlessly, EduRev has designed NEET study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in NEET.

|
Explore Courses for NEET exam
|

|
Similar NEET Doubts
A mild steel wire of length 2l m cross sectional area A m^2 is fixed horizontally between two pillars. A small mass m kg is suspended from mild point of wire. If extension in wire are within elastic limit. Then depression at the midpoint of wire will be.?
Question Description
A mild steel wire of length 2l m cross sectional area A m^2 is fixed horizontally between two pillars. A small mass m kg is suspended from mild point of wire. If extension in wire are within elastic limit. Then depression at the midpoint of wire will be.? for NEET 2024 is part of NEET preparation. The Question and answers have been prepared according to the NEET exam syllabus. Information about A mild steel wire of length 2l m cross sectional area A m^2 is fixed horizontally between two pillars. A small mass m kg is suspended from mild point of wire. If extension in wire are within elastic limit. Then depression at the midpoint of wire will be.? covers all topics & solutions for NEET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A mild steel wire of length 2l m cross sectional area A m^2 is fixed horizontally between two pillars. A small mass m kg is suspended from mild point of wire. If extension in wire are within elastic limit. Then depression at the midpoint of wire will be.?.
A mild steel wire of length 2l m cross sectional area A m^2 is fixed horizontally between two pillars. A small mass m kg is suspended from mild point of wire. If extension in wire are within elastic limit. Then depression at the midpoint of wire will be.? for NEET 2024 is part of NEET preparation. The Question and answers have been prepared according to the NEET exam syllabus. Information about A mild steel wire of length 2l m cross sectional area A m^2 is fixed horizontally between two pillars. A small mass m kg is suspended from mild point of wire. If extension in wire are within elastic limit. Then depression at the midpoint of wire will be.? covers all topics & solutions for NEET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A mild steel wire of length 2l m cross sectional area A m^2 is fixed horizontally between two pillars. A small mass m kg is suspended from mild point of wire. If extension in wire are within elastic limit. Then depression at the midpoint of wire will be.?.
Solutions for A mild steel wire of length 2l m cross sectional area A m^2 is fixed horizontally between two pillars. A small mass m kg is suspended from mild point of wire. If extension in wire are within elastic limit. Then depression at the midpoint of wire will be.? in English & in Hindi are available as part of our courses for NEET.
Download more important topics, notes, lectures and mock test series for NEET Exam by signing up for free.
Here you can find the meaning of A mild steel wire of length 2l m cross sectional area A m^2 is fixed horizontally between two pillars. A small mass m kg is suspended from mild point of wire. If extension in wire are within elastic limit. Then depression at the midpoint of wire will be.? defined & explained in the simplest way possible. Besides giving the explanation of
A mild steel wire of length 2l m cross sectional area A m^2 is fixed horizontally between two pillars. A small mass m kg is suspended from mild point of wire. If extension in wire are within elastic limit. Then depression at the midpoint of wire will be.?, a detailed solution for A mild steel wire of length 2l m cross sectional area A m^2 is fixed horizontally between two pillars. A small mass m kg is suspended from mild point of wire. If extension in wire are within elastic limit. Then depression at the midpoint of wire will be.? has been provided alongside types of A mild steel wire of length 2l m cross sectional area A m^2 is fixed horizontally between two pillars. A small mass m kg is suspended from mild point of wire. If extension in wire are within elastic limit. Then depression at the midpoint of wire will be.? theory, EduRev gives you an
ample number of questions to practice A mild steel wire of length 2l m cross sectional area A m^2 is fixed horizontally between two pillars. A small mass m kg is suspended from mild point of wire. If extension in wire are within elastic limit. Then depression at the midpoint of wire will be.? tests, examples and also practice NEET tests.

|
Explore Courses for NEET exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.

























