GATE Exam > GATE Questions > The degree sequence of a simple graph is the ...
Start Learning for Free
The degree sequence of a simple graph is the sequence of the degrees of the nodes in the graph in decreasing order. Which of the following sequences can not be the degree sequence of any graph?
I. 7, 6, 5, 4, 4, 3, 2, 1
lI. 6, 6,6, 6, 3, 3, 2, 2
III. 7, 6, 6, 4, 4, 3, 2, 2
IV. 8, 7, 7, 6, 4,2, 1,1
I. 7, 6, 5, 4, 4, 3, 2, 1
lI. 6, 6,6, 6, 3, 3, 2, 2
III. 7, 6, 6, 4, 4, 3, 2, 2
IV. 8, 7, 7, 6, 4,2, 1,1
- a)I and II
- b)Ill and IV
- c)IV only
- d)II and IV
Correct answer is option 'D'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
The degree sequence of a simple graph is the sequence of the degrees o...
Havell-Hakimi algorithm can be used to check whether a given degree sequence is a graph or not.
The algorithm is
1. Remove top node of the sequence.
2. Subtract "1" from as m any nodes in remaining sequence as the degree of top node that was removed.
3. Rearrange this sequence in non increasing order.
4. Check if resulting sequence is a graph.
5. Proceed again to step 1.
If the given sequence is not a graph we will see a violation in step 4, such as presence of negative degrees in the sequence. Otherwise the algorithm will bottom out with a degree sequence consisting of only even number of 1 ’s and any number of 0’s.
Now applying the algorithm to the degree sequences I, II, III and IV, one by one:

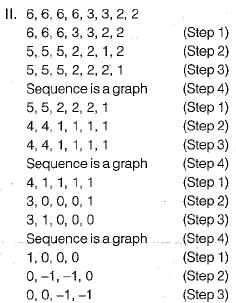
The sequence is not a graph (Step 4), since negative degrees not possible in a valid graph. So, algorithm ends.
II is cannot be the degree sequence of any graph. Similarly we can show that III is degree sequence of some graph and IV is not a degree sequence of any graph.
The algorithm is
1. Remove top node of the sequence.
2. Subtract "1" from as m any nodes in remaining sequence as the degree of top node that was removed.
3. Rearrange this sequence in non increasing order.
4. Check if resulting sequence is a graph.
5. Proceed again to step 1.
If the given sequence is not a graph we will see a violation in step 4, such as presence of negative degrees in the sequence. Otherwise the algorithm will bottom out with a degree sequence consisting of only even number of 1 ’s and any number of 0’s.
Now applying the algorithm to the degree sequences I, II, III and IV, one by one:


The sequence is not a graph (Step 4), since negative degrees not possible in a valid graph. So, algorithm ends.
II is cannot be the degree sequence of any graph. Similarly we can show that III is degree sequence of some graph and IV is not a degree sequence of any graph.
Most Upvoted Answer
The degree sequence of a simple graph is the sequence of the degrees o...
Solution:
To determine which sequence can not be the degree sequence of any graph, we can use the Havel-Hakimi algorithm. This algorithm works by repeatedly removing the largest degree node and subtracting one from the degrees of the next largest degree nodes until either a graph is formed or it is determined that the degree sequence is not graphical.
I. 7, 6, 5, 4, 4, 3, 2, 1
1. Start with the largest degree, which is 7. Remove 7 and subtract 1 from the next 7 largest degrees.
6, 5, 4, 3, 3, 2, 1
2. The next largest degree is 6. Remove 6 and subtract 1 from the next 6 largest degrees.
4, 4, 3, 2, 2, 1
3. The next largest degree is 4. Remove 4 and subtract 1 from the next 4 largest degrees.
3, 3, 2, 1, 1
4. The next largest degree is 3. Remove 3 and subtract 1 from the next 3 largest degrees.
2, 2, 1, 0
5. Since we have a degree of 0, this sequence is not graphical.
II. 6, 6, 6, 6, 3, 3, 2, 2
1. Start with the largest degree, which is 6. Remove 6 and subtract 1 from the next 6 largest degrees.
5, 5, 5, 2, 2, 1, 1
2. The next largest degree is 5. Remove 5 and subtract 1 from the next 5 largest degrees.
4, 4, 1, 1, 0, 0
3. The next largest degree is 4. Remove 4 and subtract 1 from the next 4 largest degrees.
3, 0, 0, -1, -1
4. Since we have a negative degree, this sequence is not graphical.
III. 7, 6, 6, 4, 4, 3, 2, 2
1. Start with the largest degree, which is 7. Remove 7 and subtract 1 from the next 7 largest degrees.
5, 5, 3, 3, 2, 1, 1
2. The next largest degree is 5. Remove 5 and subtract 1 from the next 5 largest degrees.
4, 2, 2, 1, 0, 0
3. The next largest degree is 4. Remove 4 and subtract 1 from the next 4 largest degrees.
1, 1, 0, 0, -1
4. The next largest degree is 1. Remove 1 and subtract 1 from the next 1 largest degree.
0, -1, 0, -2
5. Since we have a negative degree, this sequence is not graphical.
IV. 8, 7, 7, 6, 4, 2, 1, 1
1. Start with the largest degree, which is 8. Remove 8 and subtract 1 from the
To determine which sequence can not be the degree sequence of any graph, we can use the Havel-Hakimi algorithm. This algorithm works by repeatedly removing the largest degree node and subtracting one from the degrees of the next largest degree nodes until either a graph is formed or it is determined that the degree sequence is not graphical.
I. 7, 6, 5, 4, 4, 3, 2, 1
1. Start with the largest degree, which is 7. Remove 7 and subtract 1 from the next 7 largest degrees.
6, 5, 4, 3, 3, 2, 1
2. The next largest degree is 6. Remove 6 and subtract 1 from the next 6 largest degrees.
4, 4, 3, 2, 2, 1
3. The next largest degree is 4. Remove 4 and subtract 1 from the next 4 largest degrees.
3, 3, 2, 1, 1
4. The next largest degree is 3. Remove 3 and subtract 1 from the next 3 largest degrees.
2, 2, 1, 0
5. Since we have a degree of 0, this sequence is not graphical.
II. 6, 6, 6, 6, 3, 3, 2, 2
1. Start with the largest degree, which is 6. Remove 6 and subtract 1 from the next 6 largest degrees.
5, 5, 5, 2, 2, 1, 1
2. The next largest degree is 5. Remove 5 and subtract 1 from the next 5 largest degrees.
4, 4, 1, 1, 0, 0
3. The next largest degree is 4. Remove 4 and subtract 1 from the next 4 largest degrees.
3, 0, 0, -1, -1
4. Since we have a negative degree, this sequence is not graphical.
III. 7, 6, 6, 4, 4, 3, 2, 2
1. Start with the largest degree, which is 7. Remove 7 and subtract 1 from the next 7 largest degrees.
5, 5, 3, 3, 2, 1, 1
2. The next largest degree is 5. Remove 5 and subtract 1 from the next 5 largest degrees.
4, 2, 2, 1, 0, 0
3. The next largest degree is 4. Remove 4 and subtract 1 from the next 4 largest degrees.
1, 1, 0, 0, -1
4. The next largest degree is 1. Remove 1 and subtract 1 from the next 1 largest degree.
0, -1, 0, -2
5. Since we have a negative degree, this sequence is not graphical.
IV. 8, 7, 7, 6, 4, 2, 1, 1
1. Start with the largest degree, which is 8. Remove 8 and subtract 1 from the

|
Explore Courses for GATE exam
|

|
The degree sequence of a simple graph is the sequence of the degrees of the nodes in the graph in decreasing order. Which of the following sequences can not be the degree sequence of any graph?I. 7, 6, 5, 4, 4, 3, 2, 1lI. 6, 6,6, 6, 3, 3, 2, 2III. 7, 6, 6, 4, 4, 3, 2, 2IV. 8, 7, 7, 6, 4,2, 1,1a)I and IIb)Ill and IVc)IV onlyd)II and IVCorrect answer is option 'D'. Can you explain this answer?
Question Description
The degree sequence of a simple graph is the sequence of the degrees of the nodes in the graph in decreasing order. Which of the following sequences can not be the degree sequence of any graph?I. 7, 6, 5, 4, 4, 3, 2, 1lI. 6, 6,6, 6, 3, 3, 2, 2III. 7, 6, 6, 4, 4, 3, 2, 2IV. 8, 7, 7, 6, 4,2, 1,1a)I and IIb)Ill and IVc)IV onlyd)II and IVCorrect answer is option 'D'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about The degree sequence of a simple graph is the sequence of the degrees of the nodes in the graph in decreasing order. Which of the following sequences can not be the degree sequence of any graph?I. 7, 6, 5, 4, 4, 3, 2, 1lI. 6, 6,6, 6, 3, 3, 2, 2III. 7, 6, 6, 4, 4, 3, 2, 2IV. 8, 7, 7, 6, 4,2, 1,1a)I and IIb)Ill and IVc)IV onlyd)II and IVCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The degree sequence of a simple graph is the sequence of the degrees of the nodes in the graph in decreasing order. Which of the following sequences can not be the degree sequence of any graph?I. 7, 6, 5, 4, 4, 3, 2, 1lI. 6, 6,6, 6, 3, 3, 2, 2III. 7, 6, 6, 4, 4, 3, 2, 2IV. 8, 7, 7, 6, 4,2, 1,1a)I and IIb)Ill and IVc)IV onlyd)II and IVCorrect answer is option 'D'. Can you explain this answer?.
The degree sequence of a simple graph is the sequence of the degrees of the nodes in the graph in decreasing order. Which of the following sequences can not be the degree sequence of any graph?I. 7, 6, 5, 4, 4, 3, 2, 1lI. 6, 6,6, 6, 3, 3, 2, 2III. 7, 6, 6, 4, 4, 3, 2, 2IV. 8, 7, 7, 6, 4,2, 1,1a)I and IIb)Ill and IVc)IV onlyd)II and IVCorrect answer is option 'D'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about The degree sequence of a simple graph is the sequence of the degrees of the nodes in the graph in decreasing order. Which of the following sequences can not be the degree sequence of any graph?I. 7, 6, 5, 4, 4, 3, 2, 1lI. 6, 6,6, 6, 3, 3, 2, 2III. 7, 6, 6, 4, 4, 3, 2, 2IV. 8, 7, 7, 6, 4,2, 1,1a)I and IIb)Ill and IVc)IV onlyd)II and IVCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The degree sequence of a simple graph is the sequence of the degrees of the nodes in the graph in decreasing order. Which of the following sequences can not be the degree sequence of any graph?I. 7, 6, 5, 4, 4, 3, 2, 1lI. 6, 6,6, 6, 3, 3, 2, 2III. 7, 6, 6, 4, 4, 3, 2, 2IV. 8, 7, 7, 6, 4,2, 1,1a)I and IIb)Ill and IVc)IV onlyd)II and IVCorrect answer is option 'D'. Can you explain this answer?.
Solutions for The degree sequence of a simple graph is the sequence of the degrees of the nodes in the graph in decreasing order. Which of the following sequences can not be the degree sequence of any graph?I. 7, 6, 5, 4, 4, 3, 2, 1lI. 6, 6,6, 6, 3, 3, 2, 2III. 7, 6, 6, 4, 4, 3, 2, 2IV. 8, 7, 7, 6, 4,2, 1,1a)I and IIb)Ill and IVc)IV onlyd)II and IVCorrect answer is option 'D'. Can you explain this answer? in English & in Hindi are available as part of our courses for GATE.
Download more important topics, notes, lectures and mock test series for GATE Exam by signing up for free.
Here you can find the meaning of The degree sequence of a simple graph is the sequence of the degrees of the nodes in the graph in decreasing order. Which of the following sequences can not be the degree sequence of any graph?I. 7, 6, 5, 4, 4, 3, 2, 1lI. 6, 6,6, 6, 3, 3, 2, 2III. 7, 6, 6, 4, 4, 3, 2, 2IV. 8, 7, 7, 6, 4,2, 1,1a)I and IIb)Ill and IVc)IV onlyd)II and IVCorrect answer is option 'D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The degree sequence of a simple graph is the sequence of the degrees of the nodes in the graph in decreasing order. Which of the following sequences can not be the degree sequence of any graph?I. 7, 6, 5, 4, 4, 3, 2, 1lI. 6, 6,6, 6, 3, 3, 2, 2III. 7, 6, 6, 4, 4, 3, 2, 2IV. 8, 7, 7, 6, 4,2, 1,1a)I and IIb)Ill and IVc)IV onlyd)II and IVCorrect answer is option 'D'. Can you explain this answer?, a detailed solution for The degree sequence of a simple graph is the sequence of the degrees of the nodes in the graph in decreasing order. Which of the following sequences can not be the degree sequence of any graph?I. 7, 6, 5, 4, 4, 3, 2, 1lI. 6, 6,6, 6, 3, 3, 2, 2III. 7, 6, 6, 4, 4, 3, 2, 2IV. 8, 7, 7, 6, 4,2, 1,1a)I and IIb)Ill and IVc)IV onlyd)II and IVCorrect answer is option 'D'. Can you explain this answer? has been provided alongside types of The degree sequence of a simple graph is the sequence of the degrees of the nodes in the graph in decreasing order. Which of the following sequences can not be the degree sequence of any graph?I. 7, 6, 5, 4, 4, 3, 2, 1lI. 6, 6,6, 6, 3, 3, 2, 2III. 7, 6, 6, 4, 4, 3, 2, 2IV. 8, 7, 7, 6, 4,2, 1,1a)I and IIb)Ill and IVc)IV onlyd)II and IVCorrect answer is option 'D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The degree sequence of a simple graph is the sequence of the degrees of the nodes in the graph in decreasing order. Which of the following sequences can not be the degree sequence of any graph?I. 7, 6, 5, 4, 4, 3, 2, 1lI. 6, 6,6, 6, 3, 3, 2, 2III. 7, 6, 6, 4, 4, 3, 2, 2IV. 8, 7, 7, 6, 4,2, 1,1a)I and IIb)Ill and IVc)IV onlyd)II and IVCorrect answer is option 'D'. Can you explain this answer? tests, examples and also practice GATE tests.

|
Explore Courses for GATE exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
















