GATE Exam > GATE Questions > An iceberg is floating in sea water. The dens...
Start Learning for Free
An iceberg is floating in sea water. The density of ice is 0.92 gm/cm3 and that of sea water is 1.03 g/cm3. What percentage of the iceberg will be below the surface o f water ______ %.
Correct answer is '89'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
An iceberg is floating in sea water. The density of ice is 0.92 gm/cm3...
For the floating of ice - berg
weight of ice = upthrust due to displaced water
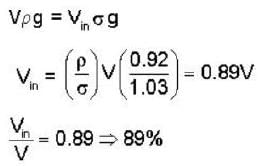
weight of ice = upthrust due to displaced water
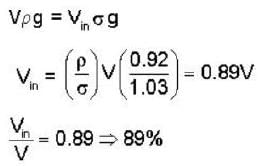
Most Upvoted Answer
An iceberg is floating in sea water. The density of ice is 0.92 gm/cm3...
Given:
Density of ice = 0.92 gm/cm3
Density of sea water = 1.03 g/cm3
To Find:
Percentage of the iceberg below the surface of water.
Solution:
Step 1: Understanding the Concept
In order to understand why a part of the iceberg is below the surface of water, we need to understand the concept of buoyancy. According to Archimedes' principle, any object partially or completely submerged in a fluid experiences an upward buoyant force equal to the weight of the fluid displaced by the object. This buoyant force helps objects float in a fluid.
Step 2: Applying the Concept
In the case of an iceberg, a significant portion of its mass is below the surface of the water due to its density being less than that of sea water. Let's assume the iceberg has a volume of 100 cm3.
Step 3: Calculation
1. Mass of the iceberg = Density × Volume = 0.92 g/cm3 × 100 cm3 = 92 g
2. The buoyant force acting on the iceberg is equal to the weight of the water displaced by the iceberg.
Buoyant force = Density of sea water × Volume of submerged part of the iceberg
Let the volume of the submerged part be V cm3.
Buoyant force = 1.03 g/cm3 × V cm3
3. Since the iceberg is in equilibrium, the weight of the iceberg is balanced by the buoyant force.
Weight of the iceberg = Mass of the iceberg × Acceleration due to gravity = 92 g × 9.8 m/s2 = 901.6 N
Buoyant force = Weight of the iceberg = 901.6 N
Therefore, 1.03 g/cm3 × V cm3 = 901.6 N
4. Solving for V:
V = 901.6 N / (1.03 g/cm3) = 875.34 cm3
5. Percentage of the iceberg below the surface of water:
Percentage = (Volume of submerged part / Total volume) × 100
= (875.34 cm3 / 100 cm3) × 100
= 875.34%
Step 4: Rounding off the Answer
Rounding off the percentage to the nearest whole number, we get:
Percentage = 89%
Therefore, approximately 89% of the iceberg will be below the surface of the water.
Density of ice = 0.92 gm/cm3
Density of sea water = 1.03 g/cm3
To Find:
Percentage of the iceberg below the surface of water.
Solution:
Step 1: Understanding the Concept
In order to understand why a part of the iceberg is below the surface of water, we need to understand the concept of buoyancy. According to Archimedes' principle, any object partially or completely submerged in a fluid experiences an upward buoyant force equal to the weight of the fluid displaced by the object. This buoyant force helps objects float in a fluid.
Step 2: Applying the Concept
In the case of an iceberg, a significant portion of its mass is below the surface of the water due to its density being less than that of sea water. Let's assume the iceberg has a volume of 100 cm3.
Step 3: Calculation
1. Mass of the iceberg = Density × Volume = 0.92 g/cm3 × 100 cm3 = 92 g
2. The buoyant force acting on the iceberg is equal to the weight of the water displaced by the iceberg.
Buoyant force = Density of sea water × Volume of submerged part of the iceberg
Let the volume of the submerged part be V cm3.
Buoyant force = 1.03 g/cm3 × V cm3
3. Since the iceberg is in equilibrium, the weight of the iceberg is balanced by the buoyant force.
Weight of the iceberg = Mass of the iceberg × Acceleration due to gravity = 92 g × 9.8 m/s2 = 901.6 N
Buoyant force = Weight of the iceberg = 901.6 N
Therefore, 1.03 g/cm3 × V cm3 = 901.6 N
4. Solving for V:
V = 901.6 N / (1.03 g/cm3) = 875.34 cm3
5. Percentage of the iceberg below the surface of water:
Percentage = (Volume of submerged part / Total volume) × 100
= (875.34 cm3 / 100 cm3) × 100
= 875.34%
Step 4: Rounding off the Answer
Rounding off the percentage to the nearest whole number, we get:
Percentage = 89%
Therefore, approximately 89% of the iceberg will be below the surface of the water.

|
Explore Courses for GATE exam
|

|
Similar GATE Doubts
An iceberg is floating in sea water. The density of ice is 0.92 gm/cm3 and that of sea water is 1.03 g/cm3. What percentage of the iceberg will be below the surface o f water ______ %.Correct answer is '89'. Can you explain this answer?
Question Description
An iceberg is floating in sea water. The density of ice is 0.92 gm/cm3 and that of sea water is 1.03 g/cm3. What percentage of the iceberg will be below the surface o f water ______ %.Correct answer is '89'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about An iceberg is floating in sea water. The density of ice is 0.92 gm/cm3 and that of sea water is 1.03 g/cm3. What percentage of the iceberg will be below the surface o f water ______ %.Correct answer is '89'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for An iceberg is floating in sea water. The density of ice is 0.92 gm/cm3 and that of sea water is 1.03 g/cm3. What percentage of the iceberg will be below the surface o f water ______ %.Correct answer is '89'. Can you explain this answer?.
An iceberg is floating in sea water. The density of ice is 0.92 gm/cm3 and that of sea water is 1.03 g/cm3. What percentage of the iceberg will be below the surface o f water ______ %.Correct answer is '89'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about An iceberg is floating in sea water. The density of ice is 0.92 gm/cm3 and that of sea water is 1.03 g/cm3. What percentage of the iceberg will be below the surface o f water ______ %.Correct answer is '89'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for An iceberg is floating in sea water. The density of ice is 0.92 gm/cm3 and that of sea water is 1.03 g/cm3. What percentage of the iceberg will be below the surface o f water ______ %.Correct answer is '89'. Can you explain this answer?.
Solutions for An iceberg is floating in sea water. The density of ice is 0.92 gm/cm3 and that of sea water is 1.03 g/cm3. What percentage of the iceberg will be below the surface o f water ______ %.Correct answer is '89'. Can you explain this answer? in English & in Hindi are available as part of our courses for GATE.
Download more important topics, notes, lectures and mock test series for GATE Exam by signing up for free.
Here you can find the meaning of An iceberg is floating in sea water. The density of ice is 0.92 gm/cm3 and that of sea water is 1.03 g/cm3. What percentage of the iceberg will be below the surface o f water ______ %.Correct answer is '89'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
An iceberg is floating in sea water. The density of ice is 0.92 gm/cm3 and that of sea water is 1.03 g/cm3. What percentage of the iceberg will be below the surface o f water ______ %.Correct answer is '89'. Can you explain this answer?, a detailed solution for An iceberg is floating in sea water. The density of ice is 0.92 gm/cm3 and that of sea water is 1.03 g/cm3. What percentage of the iceberg will be below the surface o f water ______ %.Correct answer is '89'. Can you explain this answer? has been provided alongside types of An iceberg is floating in sea water. The density of ice is 0.92 gm/cm3 and that of sea water is 1.03 g/cm3. What percentage of the iceberg will be below the surface o f water ______ %.Correct answer is '89'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice An iceberg is floating in sea water. The density of ice is 0.92 gm/cm3 and that of sea water is 1.03 g/cm3. What percentage of the iceberg will be below the surface o f water ______ %.Correct answer is '89'. Can you explain this answer? tests, examples and also practice GATE tests.

|
Explore Courses for GATE exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















