GATE Exam > GATE Questions > A strain gauge of resistance 120 and gauge fa...
Start Learning for Free
A strain gauge of resistance 120 and gauge factor 2.0 is at zero strain condition A 200 kΩ
fixed resistance is connected in parallel with it. Then the combination will represent an equivalent
strain of
- a)+ 5290 μm/m
- b)zero
- c)– 123.8 μm/m
- d)– 300 μm/m
Correct answer is option 'D'. Can you explain this answer?
Verified Answer
A strain gauge of resistance 120 and gauge factor 2.0 is at zero strai...
Gauge Factor, Gf =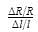 
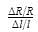
2 =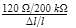 
= 
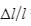= -300 μm/m
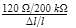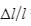= = -300 μm/mNegative Sign shows compressive strain.
Most Upvoted Answer
A strain gauge of resistance 120 and gauge factor 2.0 is at zero strai...
Gauge Factor, Gf =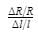 
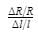
2 =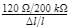 
= 
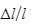= -300 μm/m
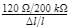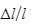= = -300 μm/mNegative Sign shows compressive strain.
Free Test
FREE
| Start Free Test |
Community Answer
A strain gauge of resistance 120 and gauge factor 2.0 is at zero strai...
Calculation:
- The equivalent resistance of the parallel combination of the strain gauge (120 ohms) and the fixed resistance (200 kΩ) can be calculated using the formula:
\[
R_{eq} = \frac{R_1 \times R_2}{R_1 + R_2}
\]
- where \(R_1 = 120 \Omega\) and \(R_2 = 200 k\Omega = 200,000 \Omega\).
- Substituting the values into the formula:
\[
R_{eq} = \frac{120 \times 200,000}{120 + 200,000} = \frac{24,000,000}{200,120} \approx 119.95 \Omega
\]
Strain Calculation:
- The gauge factor (GF) is given as 2.0, which means that for a strain of 1 unit, the resistance changes by a factor of 2.0 times the initial resistance.
- The change in resistance (\(\Delta R\)) due to strain can be calculated using the formula:
\[
\Delta R = GF \times R_0 \times \epsilon
\]
- where \(R_0 = 120 \Omega\) is the initial resistance and \(\epsilon\) is the strain.
- In this case, the equivalent resistance (\(R_{eq}\)) of the parallel combination is 119.95 ohms.
- Substituting the values into the formula:
\[
119.95 = 2.0 \times 120 \times \epsilon
\]
- Solving for \(\epsilon\):
\[
\epsilon = \frac{119.95}{240} \approx 0.4998
\]
- The equivalent strain is -0.4998, which is approximately -300 μm/m.
Therefore, the correct answer is option D: \(-300 \mu m/m\).
- The equivalent resistance of the parallel combination of the strain gauge (120 ohms) and the fixed resistance (200 kΩ) can be calculated using the formula:
\[
R_{eq} = \frac{R_1 \times R_2}{R_1 + R_2}
\]
- where \(R_1 = 120 \Omega\) and \(R_2 = 200 k\Omega = 200,000 \Omega\).
- Substituting the values into the formula:
\[
R_{eq} = \frac{120 \times 200,000}{120 + 200,000} = \frac{24,000,000}{200,120} \approx 119.95 \Omega
\]
Strain Calculation:
- The gauge factor (GF) is given as 2.0, which means that for a strain of 1 unit, the resistance changes by a factor of 2.0 times the initial resistance.
- The change in resistance (\(\Delta R\)) due to strain can be calculated using the formula:
\[
\Delta R = GF \times R_0 \times \epsilon
\]
- where \(R_0 = 120 \Omega\) is the initial resistance and \(\epsilon\) is the strain.
- In this case, the equivalent resistance (\(R_{eq}\)) of the parallel combination is 119.95 ohms.
- Substituting the values into the formula:
\[
119.95 = 2.0 \times 120 \times \epsilon
\]
- Solving for \(\epsilon\):
\[
\epsilon = \frac{119.95}{240} \approx 0.4998
\]
- The equivalent strain is -0.4998, which is approximately -300 μm/m.
Therefore, the correct answer is option D: \(-300 \mu m/m\).
|
Explore Courses for GATE exam
|
|
Similar GATE Doubts
A strain gauge of resistance 120 and gauge factor 2.0 is at zero strain condition A 200 kΩfixed resistance is connected in parallel with it. Then the combination will represent an equivalentstrain ofa)+ 5290 μm/mb)zeroc)– 123.8 μm/md)– 300 μm/mCorrect answer is option 'D'. Can you explain this answer?
Question Description
A strain gauge of resistance 120 and gauge factor 2.0 is at zero strain condition A 200 kΩfixed resistance is connected in parallel with it. Then the combination will represent an equivalentstrain ofa)+ 5290 μm/mb)zeroc)– 123.8 μm/md)– 300 μm/mCorrect answer is option 'D'. Can you explain this answer? for GATE 2025 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about A strain gauge of resistance 120 and gauge factor 2.0 is at zero strain condition A 200 kΩfixed resistance is connected in parallel with it. Then the combination will represent an equivalentstrain ofa)+ 5290 μm/mb)zeroc)– 123.8 μm/md)– 300 μm/mCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for GATE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A strain gauge of resistance 120 and gauge factor 2.0 is at zero strain condition A 200 kΩfixed resistance is connected in parallel with it. Then the combination will represent an equivalentstrain ofa)+ 5290 μm/mb)zeroc)– 123.8 μm/md)– 300 μm/mCorrect answer is option 'D'. Can you explain this answer?.
A strain gauge of resistance 120 and gauge factor 2.0 is at zero strain condition A 200 kΩfixed resistance is connected in parallel with it. Then the combination will represent an equivalentstrain ofa)+ 5290 μm/mb)zeroc)– 123.8 μm/md)– 300 μm/mCorrect answer is option 'D'. Can you explain this answer? for GATE 2025 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about A strain gauge of resistance 120 and gauge factor 2.0 is at zero strain condition A 200 kΩfixed resistance is connected in parallel with it. Then the combination will represent an equivalentstrain ofa)+ 5290 μm/mb)zeroc)– 123.8 μm/md)– 300 μm/mCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for GATE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A strain gauge of resistance 120 and gauge factor 2.0 is at zero strain condition A 200 kΩfixed resistance is connected in parallel with it. Then the combination will represent an equivalentstrain ofa)+ 5290 μm/mb)zeroc)– 123.8 μm/md)– 300 μm/mCorrect answer is option 'D'. Can you explain this answer?.
Solutions for A strain gauge of resistance 120 and gauge factor 2.0 is at zero strain condition A 200 kΩfixed resistance is connected in parallel with it. Then the combination will represent an equivalentstrain ofa)+ 5290 μm/mb)zeroc)– 123.8 μm/md)– 300 μm/mCorrect answer is option 'D'. Can you explain this answer? in English & in Hindi are available as part of our courses for GATE.
Download more important topics, notes, lectures and mock test series for GATE Exam by signing up for free.
Here you can find the meaning of A strain gauge of resistance 120 and gauge factor 2.0 is at zero strain condition A 200 kΩfixed resistance is connected in parallel with it. Then the combination will represent an equivalentstrain ofa)+ 5290 μm/mb)zeroc)– 123.8 μm/md)– 300 μm/mCorrect answer is option 'D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A strain gauge of resistance 120 and gauge factor 2.0 is at zero strain condition A 200 kΩfixed resistance is connected in parallel with it. Then the combination will represent an equivalentstrain ofa)+ 5290 μm/mb)zeroc)– 123.8 μm/md)– 300 μm/mCorrect answer is option 'D'. Can you explain this answer?, a detailed solution for A strain gauge of resistance 120 and gauge factor 2.0 is at zero strain condition A 200 kΩfixed resistance is connected in parallel with it. Then the combination will represent an equivalentstrain ofa)+ 5290 μm/mb)zeroc)– 123.8 μm/md)– 300 μm/mCorrect answer is option 'D'. Can you explain this answer? has been provided alongside types of A strain gauge of resistance 120 and gauge factor 2.0 is at zero strain condition A 200 kΩfixed resistance is connected in parallel with it. Then the combination will represent an equivalentstrain ofa)+ 5290 μm/mb)zeroc)– 123.8 μm/md)– 300 μm/mCorrect answer is option 'D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A strain gauge of resistance 120 and gauge factor 2.0 is at zero strain condition A 200 kΩfixed resistance is connected in parallel with it. Then the combination will represent an equivalentstrain ofa)+ 5290 μm/mb)zeroc)– 123.8 μm/md)– 300 μm/mCorrect answer is option 'D'. Can you explain this answer? tests, examples and also practice GATE tests.
|
|
Explore Courses for GATE exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup