Class 10 Exam > Class 10 Questions > What is the probability of getting 53 Tuesday...
Start Learning for Free
What is the probability of getting 53 Tuesday in a leap year?
- a)
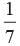
- b)
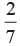
- c)
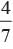
- d)None of these
Correct answer is option 'B'. Can you explain this answer?
Most Upvoted Answer
What is the probability of getting 53 Tuesday in a leap year?a)b)c)d)N...
In a leap year, there are 52 weeks and 2 days.
These two days may be (Monday, Tuesday) (Tuesday, Wednesday), (Wednesday, Thursday) (Thursday, Friday), (Friday, Saturday), (Saturday, Sunday), (Sunday, Monday).
These two days may be (Monday, Tuesday) (Tuesday, Wednesday), (Wednesday, Thursday) (Thursday, Friday), (Friday, Saturday), (Saturday, Sunday), (Sunday, Monday).
Free Test
FREE
| Start Free Test |
Community Answer
What is the probability of getting 53 Tuesday in a leap year?a)b)c)d)N...
To find the probability of getting 53 Tuesdays in a leap year, we need to consider two primary factors: the number of days in a leap year and the number of Tuesdays in a year.
1. Number of days in a leap year:
In a leap year, there are 366 days instead of the usual 365 days. This additional day is added to keep the calendar year synchronized with the solar year. This extra day is known as a leap day and is added to the month of February, making it 29 days instead of the usual 28 days.
2. Number of Tuesdays in a year:
To determine the number of Tuesdays in a year, we need to consider the total number of days and the pattern of the days of the week throughout the year. In a non-leap year, there are 52 weeks and 1 day, resulting in 52 Tuesdays. However, in a leap year, there are 52 weeks and 2 days (since there is an extra day in February), resulting in 53 Tuesdays.
Therefore, the probability of getting 53 Tuesdays in a leap year can be calculated as follows:
Probability = Number of favorable outcomes / Total number of outcomes
Number of favorable outcomes:
In a leap year, the favorable outcome is getting exactly 53 Tuesdays. Since there is only one leap day in February, we need to ensure that the first day of the year is not a Tuesday, as this would result in 54 Tuesdays. Therefore, the favorable outcome is having the first day of the year as any day other than Tuesday and having 53 Tuesdays in total.
Total number of outcomes:
The total number of outcomes is equal to the total number of possible arrangements of the days of the year, which is 7 (the number of days in a week).
Hence, the probability of getting 53 Tuesdays in a leap year is 1/7 or option B.
1. Number of days in a leap year:
In a leap year, there are 366 days instead of the usual 365 days. This additional day is added to keep the calendar year synchronized with the solar year. This extra day is known as a leap day and is added to the month of February, making it 29 days instead of the usual 28 days.
2. Number of Tuesdays in a year:
To determine the number of Tuesdays in a year, we need to consider the total number of days and the pattern of the days of the week throughout the year. In a non-leap year, there are 52 weeks and 1 day, resulting in 52 Tuesdays. However, in a leap year, there are 52 weeks and 2 days (since there is an extra day in February), resulting in 53 Tuesdays.
Therefore, the probability of getting 53 Tuesdays in a leap year can be calculated as follows:
Probability = Number of favorable outcomes / Total number of outcomes
Number of favorable outcomes:
In a leap year, the favorable outcome is getting exactly 53 Tuesdays. Since there is only one leap day in February, we need to ensure that the first day of the year is not a Tuesday, as this would result in 54 Tuesdays. Therefore, the favorable outcome is having the first day of the year as any day other than Tuesday and having 53 Tuesdays in total.
Total number of outcomes:
The total number of outcomes is equal to the total number of possible arrangements of the days of the year, which is 7 (the number of days in a week).
Hence, the probability of getting 53 Tuesdays in a leap year is 1/7 or option B.

|
Explore Courses for Class 10 exam
|

|
Question Description
What is the probability of getting 53 Tuesday in a leap year?a)b)c)d)None of theseCorrect answer is option 'B'. Can you explain this answer? for Class 10 2025 is part of Class 10 preparation. The Question and answers have been prepared according to the Class 10 exam syllabus. Information about What is the probability of getting 53 Tuesday in a leap year?a)b)c)d)None of theseCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Class 10 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for What is the probability of getting 53 Tuesday in a leap year?a)b)c)d)None of theseCorrect answer is option 'B'. Can you explain this answer?.
What is the probability of getting 53 Tuesday in a leap year?a)b)c)d)None of theseCorrect answer is option 'B'. Can you explain this answer? for Class 10 2025 is part of Class 10 preparation. The Question and answers have been prepared according to the Class 10 exam syllabus. Information about What is the probability of getting 53 Tuesday in a leap year?a)b)c)d)None of theseCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Class 10 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for What is the probability of getting 53 Tuesday in a leap year?a)b)c)d)None of theseCorrect answer is option 'B'. Can you explain this answer?.
Solutions for What is the probability of getting 53 Tuesday in a leap year?a)b)c)d)None of theseCorrect answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Class 10.
Download more important topics, notes, lectures and mock test series for Class 10 Exam by signing up for free.
Here you can find the meaning of What is the probability of getting 53 Tuesday in a leap year?a)b)c)d)None of theseCorrect answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
What is the probability of getting 53 Tuesday in a leap year?a)b)c)d)None of theseCorrect answer is option 'B'. Can you explain this answer?, a detailed solution for What is the probability of getting 53 Tuesday in a leap year?a)b)c)d)None of theseCorrect answer is option 'B'. Can you explain this answer? has been provided alongside types of What is the probability of getting 53 Tuesday in a leap year?a)b)c)d)None of theseCorrect answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice What is the probability of getting 53 Tuesday in a leap year?a)b)c)d)None of theseCorrect answer is option 'B'. Can you explain this answer? tests, examples and also practice Class 10 tests.

|
Explore Courses for Class 10 exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















