NEET Exam > NEET Questions > Consider three charges q1,q2,q3 each equal to...
Start Learning for Free
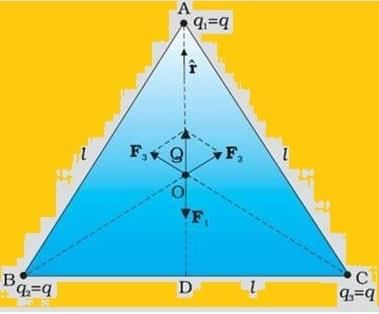
Consider three charges q1,q2,q3 each equal to q at the vertices of an equilateral triangle of side l. What is the force on a charge Q (with the same sign as q) placed at the centroid of the triangle.?
Verified Answer
Consider three charges q1,q2,q3 each equal to q at the vertices of an ...
Using symmetry here we see that that all froces
=) F1 = F2 = F3 = kQq/r^2
here r = AO = BO = CO
using trigonometry we can find " r "
Here we can see that length of each side of ∆ ABC is " L "
=) In ∆ BOD
Angle OBD = 30degree
and BD = L/2
COS 30degree = L/2r =) r = L/2 x sec30degree
=). r = L/2 x 2/√3 = ( 1/√3 ) L -----(1)
so we can see tha all forces are equal
F1 = F2 = F3 = kQq/ (L/√3)^2 = 3kQq/L^2
and angle between each forces is 120degree
using Lami's theorem if all forces are equal in magnitude and angle between then is also equal and planar then resultant force is 0
=) Fnet = F1 +F2 + F3 = 0
=) here we add forces using vectors to get net force = 0
so Net force on charge " Q " placed at centroid is zero.
 This question is part of UPSC exam. View all NEET courses
This question is part of UPSC exam. View all NEET courses
Most Upvoted Answer
Consider three charges q1,q2,q3 each equal to q at the vertices of an ...
Net force on charqe Q is zero as all the vector will cut off each other
Community Answer
Consider three charges q1,q2,q3 each equal to q at the vertices of an ...
Force on a Charge at the Centroid of an Equilateral Triangle
The force experienced by a charge at the centroid of an equilateral triangle can be determined by considering the individual forces exerted by each of the charges q1, q2, and q3.
Definition of Centroid
The centroid of a triangle is the point where all three medians intersect. A median is a line segment that connects a vertex of a triangle to the midpoint of the opposing side. In an equilateral triangle, all three medians are concurrent at a single point, which is the centroid.
Calculating the Force
To determine the force on a charge Q placed at the centroid, we need to consider the forces exerted by each of the charges q1, q2, and q3 individually and then find their vector sum.
1. Force due to q1:
The force exerted by q1 on Q can be calculated using Coulomb's Law, which states that the force between two charges is directly proportional to the product of their charges and inversely proportional to the square of the distance between them.
- The distance between q1 and Q is l/√3, as the centroid divides each median in a 2:1 ratio.
- The force exerted by q1 on Q is given by F1 = k * (Q * q1) / (l/√3)^2, where k is the electrostatic constant.
2. Force due to q2:
Similar to q1, the force exerted by q2 on Q can be calculated using Coulomb's Law.
- The distance between q2 and Q is also l/√3.
- The force exerted by q2 on Q is given by F2 = k * (Q * q2) / (l/√3)^2.
3. Force due to q3:
The force exerted by q3 on Q can be found using Coulomb's Law as well.
- The distance between q3 and Q is also l/√3.
- The force exerted by q3 on Q is given by F3 = k * (Q * q3) / (l/√3)^2.
Vector Sum of Forces
The force on Q at the centroid is the vector sum of the forces exerted by q1, q2, and q3.
- The total force on Q is given by the vector sum: F_total = F1 + F2 + F3.
Explanation
When charges q1, q2, and q3 are placed at the vertices of an equilateral triangle, they create an electric field around them. This electric field exerts forces on any other charges placed within it. The force experienced by a charge at the centroid of the triangle is the result of the combined effect of the electric fields created by q1, q2, and q3.
By calculating the individual forces exerted by each charge using Coulomb's Law and then summing them as vectors, we can determine the total force on the charge Q at the centroid.
It is important to note that the forces on Q due to q1, q2, and q3 will have both magnitude and direction. The direction of each force will depend on the relative positions of the charges and the sign
The force experienced by a charge at the centroid of an equilateral triangle can be determined by considering the individual forces exerted by each of the charges q1, q2, and q3.
Definition of Centroid
The centroid of a triangle is the point where all three medians intersect. A median is a line segment that connects a vertex of a triangle to the midpoint of the opposing side. In an equilateral triangle, all three medians are concurrent at a single point, which is the centroid.
Calculating the Force
To determine the force on a charge Q placed at the centroid, we need to consider the forces exerted by each of the charges q1, q2, and q3 individually and then find their vector sum.
1. Force due to q1:
The force exerted by q1 on Q can be calculated using Coulomb's Law, which states that the force between two charges is directly proportional to the product of their charges and inversely proportional to the square of the distance between them.
- The distance between q1 and Q is l/√3, as the centroid divides each median in a 2:1 ratio.
- The force exerted by q1 on Q is given by F1 = k * (Q * q1) / (l/√3)^2, where k is the electrostatic constant.
2. Force due to q2:
Similar to q1, the force exerted by q2 on Q can be calculated using Coulomb's Law.
- The distance between q2 and Q is also l/√3.
- The force exerted by q2 on Q is given by F2 = k * (Q * q2) / (l/√3)^2.
3. Force due to q3:
The force exerted by q3 on Q can be found using Coulomb's Law as well.
- The distance between q3 and Q is also l/√3.
- The force exerted by q3 on Q is given by F3 = k * (Q * q3) / (l/√3)^2.
Vector Sum of Forces
The force on Q at the centroid is the vector sum of the forces exerted by q1, q2, and q3.
- The total force on Q is given by the vector sum: F_total = F1 + F2 + F3.
Explanation
When charges q1, q2, and q3 are placed at the vertices of an equilateral triangle, they create an electric field around them. This electric field exerts forces on any other charges placed within it. The force experienced by a charge at the centroid of the triangle is the result of the combined effect of the electric fields created by q1, q2, and q3.
By calculating the individual forces exerted by each charge using Coulomb's Law and then summing them as vectors, we can determine the total force on the charge Q at the centroid.
It is important to note that the forces on Q due to q1, q2, and q3 will have both magnitude and direction. The direction of each force will depend on the relative positions of the charges and the sign
Attention NEET Students!
To make sure you are not studying endlessly, EduRev has designed NEET study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in NEET.

|
Explore Courses for NEET exam
|

|
Similar NEET Doubts
Consider three charges q1,q2,q3 each equal to q at the vertices of an equilateral triangle of side l. What is the force on a charge Q (with the same sign as q) placed at the centroid of the triangle.?
Question Description
Consider three charges q1,q2,q3 each equal to q at the vertices of an equilateral triangle of side l. What is the force on a charge Q (with the same sign as q) placed at the centroid of the triangle.? for NEET 2024 is part of NEET preparation. The Question and answers have been prepared according to the NEET exam syllabus. Information about Consider three charges q1,q2,q3 each equal to q at the vertices of an equilateral triangle of side l. What is the force on a charge Q (with the same sign as q) placed at the centroid of the triangle.? covers all topics & solutions for NEET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Consider three charges q1,q2,q3 each equal to q at the vertices of an equilateral triangle of side l. What is the force on a charge Q (with the same sign as q) placed at the centroid of the triangle.?.
Consider three charges q1,q2,q3 each equal to q at the vertices of an equilateral triangle of side l. What is the force on a charge Q (with the same sign as q) placed at the centroid of the triangle.? for NEET 2024 is part of NEET preparation. The Question and answers have been prepared according to the NEET exam syllabus. Information about Consider three charges q1,q2,q3 each equal to q at the vertices of an equilateral triangle of side l. What is the force on a charge Q (with the same sign as q) placed at the centroid of the triangle.? covers all topics & solutions for NEET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Consider three charges q1,q2,q3 each equal to q at the vertices of an equilateral triangle of side l. What is the force on a charge Q (with the same sign as q) placed at the centroid of the triangle.?.
Solutions for Consider three charges q1,q2,q3 each equal to q at the vertices of an equilateral triangle of side l. What is the force on a charge Q (with the same sign as q) placed at the centroid of the triangle.? in English & in Hindi are available as part of our courses for NEET.
Download more important topics, notes, lectures and mock test series for NEET Exam by signing up for free.
Here you can find the meaning of Consider three charges q1,q2,q3 each equal to q at the vertices of an equilateral triangle of side l. What is the force on a charge Q (with the same sign as q) placed at the centroid of the triangle.? defined & explained in the simplest way possible. Besides giving the explanation of
Consider three charges q1,q2,q3 each equal to q at the vertices of an equilateral triangle of side l. What is the force on a charge Q (with the same sign as q) placed at the centroid of the triangle.?, a detailed solution for Consider three charges q1,q2,q3 each equal to q at the vertices of an equilateral triangle of side l. What is the force on a charge Q (with the same sign as q) placed at the centroid of the triangle.? has been provided alongside types of Consider three charges q1,q2,q3 each equal to q at the vertices of an equilateral triangle of side l. What is the force on a charge Q (with the same sign as q) placed at the centroid of the triangle.? theory, EduRev gives you an
ample number of questions to practice Consider three charges q1,q2,q3 each equal to q at the vertices of an equilateral triangle of side l. What is the force on a charge Q (with the same sign as q) placed at the centroid of the triangle.? tests, examples and also practice NEET tests.

|
Explore Courses for NEET exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.

























