GATE Exam > GATE Questions > Vehicles arriving at an intersection from one...
Start Learning for Free
Vehicles arriving at an intersection from one of the approach road follow the Poisson distribution. The mean rate of arrival is 900 vehicles per hour. If a gap is defined as the time difference between two successive vehicle arrivals (with vehicles assumed to be points), the probability (up to four decimal places) that the gap is greater than 8 seconds is__
- a)0.1354
- b)0.164
- c)0.174
- d)none
Correct answer is option 'A'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
Vehicles arriving at an intersection from one of the approach road fol...
By Poission’s distribution
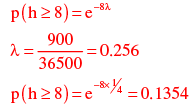
Most Upvoted Answer
Vehicles arriving at an intersection from one of the approach road fol...
Given:
- The arrival of vehicles at an intersection from one of the approach roads follows a Poisson distribution.
- The mean rate of arrival is 900 vehicles per hour.
To find:
- The probability that the gap between two successive vehicle arrivals is greater than 8 seconds.
Solution:
First, we need to convert the mean rate of arrival from vehicles per hour to vehicles per second. Since there are 3600 seconds in an hour, the mean rate of arrival can be calculated as follows:
Mean rate of arrival = 900 vehicles per hour = 900 / 3600 vehicles per second = 0.25 vehicles per second
Poisson distribution:
The Poisson distribution is a discrete probability distribution that expresses the probability of a given number of events occurring in a fixed interval of time or space if these events occur with a known constant mean rate and are independent of the time since the last event.
In this case, the gap between two successive vehicle arrivals follows a Poisson distribution with a mean rate of 0.25 vehicles per second.
Calculating the probability:
To calculate the probability that the gap between two successive vehicle arrivals is greater than 8 seconds, we need to calculate the cumulative probability of the Poisson distribution for a gap of 8 seconds or less and subtract it from 1.
Let's denote the random variable for the gap between two successive vehicle arrivals as X.
P(X > 8) = 1 - P(X ≤ 8)
Using the Poisson distribution formula, the probability mass function (PMF) for X is given by:
P(X = x) = (e^(-λ) * λ^x) / x!
Where λ is the mean rate of arrival and x is the number of events.
To calculate P(X ≤ 8), we sum up the probabilities for x = 0, 1, 2, ..., 8.
P(X ≤ 8) = P(X = 0) + P(X = 1) + P(X = 2) + ... + P(X = 8)
Using the Poisson distribution formula, we can calculate each individual term and sum them up.
After calculating P(X ≤ 8), we can substitute this value into the equation:
P(X > 8) = 1 - P(X ≤ 8)
Calculating this value will give us the probability that the gap between two successive vehicle arrivals is greater than 8 seconds.
Answer:
The correct answer is option 'A' (0.1354).
- The arrival of vehicles at an intersection from one of the approach roads follows a Poisson distribution.
- The mean rate of arrival is 900 vehicles per hour.
To find:
- The probability that the gap between two successive vehicle arrivals is greater than 8 seconds.
Solution:
First, we need to convert the mean rate of arrival from vehicles per hour to vehicles per second. Since there are 3600 seconds in an hour, the mean rate of arrival can be calculated as follows:
Mean rate of arrival = 900 vehicles per hour = 900 / 3600 vehicles per second = 0.25 vehicles per second
Poisson distribution:
The Poisson distribution is a discrete probability distribution that expresses the probability of a given number of events occurring in a fixed interval of time or space if these events occur with a known constant mean rate and are independent of the time since the last event.
In this case, the gap between two successive vehicle arrivals follows a Poisson distribution with a mean rate of 0.25 vehicles per second.
Calculating the probability:
To calculate the probability that the gap between two successive vehicle arrivals is greater than 8 seconds, we need to calculate the cumulative probability of the Poisson distribution for a gap of 8 seconds or less and subtract it from 1.
Let's denote the random variable for the gap between two successive vehicle arrivals as X.
P(X > 8) = 1 - P(X ≤ 8)
Using the Poisson distribution formula, the probability mass function (PMF) for X is given by:
P(X = x) = (e^(-λ) * λ^x) / x!
Where λ is the mean rate of arrival and x is the number of events.
To calculate P(X ≤ 8), we sum up the probabilities for x = 0, 1, 2, ..., 8.
P(X ≤ 8) = P(X = 0) + P(X = 1) + P(X = 2) + ... + P(X = 8)
Using the Poisson distribution formula, we can calculate each individual term and sum them up.
After calculating P(X ≤ 8), we can substitute this value into the equation:
P(X > 8) = 1 - P(X ≤ 8)
Calculating this value will give us the probability that the gap between two successive vehicle arrivals is greater than 8 seconds.
Answer:
The correct answer is option 'A' (0.1354).

|
Explore Courses for GATE exam
|

|
Similar GATE Doubts
Vehicles arriving at an intersection from one of the approach road follow the Poisson distribution. The mean rate of arrival is900 vehicles per hour. If a gap is defined as the timedifference between two successive vehicle arrivals (with vehicles assumed to be points), the probability (up to four decimal places) that the gap is greater than 8 seconds is__a)0.1354b)0.164c)0.174d)noneCorrect answer is option 'A'. Can you explain this answer?
Question Description
Vehicles arriving at an intersection from one of the approach road follow the Poisson distribution. The mean rate of arrival is900 vehicles per hour. If a gap is defined as the timedifference between two successive vehicle arrivals (with vehicles assumed to be points), the probability (up to four decimal places) that the gap is greater than 8 seconds is__a)0.1354b)0.164c)0.174d)noneCorrect answer is option 'A'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about Vehicles arriving at an intersection from one of the approach road follow the Poisson distribution. The mean rate of arrival is900 vehicles per hour. If a gap is defined as the timedifference between two successive vehicle arrivals (with vehicles assumed to be points), the probability (up to four decimal places) that the gap is greater than 8 seconds is__a)0.1354b)0.164c)0.174d)noneCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Vehicles arriving at an intersection from one of the approach road follow the Poisson distribution. The mean rate of arrival is900 vehicles per hour. If a gap is defined as the timedifference between two successive vehicle arrivals (with vehicles assumed to be points), the probability (up to four decimal places) that the gap is greater than 8 seconds is__a)0.1354b)0.164c)0.174d)noneCorrect answer is option 'A'. Can you explain this answer?.
Vehicles arriving at an intersection from one of the approach road follow the Poisson distribution. The mean rate of arrival is900 vehicles per hour. If a gap is defined as the timedifference between two successive vehicle arrivals (with vehicles assumed to be points), the probability (up to four decimal places) that the gap is greater than 8 seconds is__a)0.1354b)0.164c)0.174d)noneCorrect answer is option 'A'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about Vehicles arriving at an intersection from one of the approach road follow the Poisson distribution. The mean rate of arrival is900 vehicles per hour. If a gap is defined as the timedifference between two successive vehicle arrivals (with vehicles assumed to be points), the probability (up to four decimal places) that the gap is greater than 8 seconds is__a)0.1354b)0.164c)0.174d)noneCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Vehicles arriving at an intersection from one of the approach road follow the Poisson distribution. The mean rate of arrival is900 vehicles per hour. If a gap is defined as the timedifference between two successive vehicle arrivals (with vehicles assumed to be points), the probability (up to four decimal places) that the gap is greater than 8 seconds is__a)0.1354b)0.164c)0.174d)noneCorrect answer is option 'A'. Can you explain this answer?.
Solutions for Vehicles arriving at an intersection from one of the approach road follow the Poisson distribution. The mean rate of arrival is900 vehicles per hour. If a gap is defined as the timedifference between two successive vehicle arrivals (with vehicles assumed to be points), the probability (up to four decimal places) that the gap is greater than 8 seconds is__a)0.1354b)0.164c)0.174d)noneCorrect answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for GATE.
Download more important topics, notes, lectures and mock test series for GATE Exam by signing up for free.
Here you can find the meaning of Vehicles arriving at an intersection from one of the approach road follow the Poisson distribution. The mean rate of arrival is900 vehicles per hour. If a gap is defined as the timedifference between two successive vehicle arrivals (with vehicles assumed to be points), the probability (up to four decimal places) that the gap is greater than 8 seconds is__a)0.1354b)0.164c)0.174d)noneCorrect answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Vehicles arriving at an intersection from one of the approach road follow the Poisson distribution. The mean rate of arrival is900 vehicles per hour. If a gap is defined as the timedifference between two successive vehicle arrivals (with vehicles assumed to be points), the probability (up to four decimal places) that the gap is greater than 8 seconds is__a)0.1354b)0.164c)0.174d)noneCorrect answer is option 'A'. Can you explain this answer?, a detailed solution for Vehicles arriving at an intersection from one of the approach road follow the Poisson distribution. The mean rate of arrival is900 vehicles per hour. If a gap is defined as the timedifference between two successive vehicle arrivals (with vehicles assumed to be points), the probability (up to four decimal places) that the gap is greater than 8 seconds is__a)0.1354b)0.164c)0.174d)noneCorrect answer is option 'A'. Can you explain this answer? has been provided alongside types of Vehicles arriving at an intersection from one of the approach road follow the Poisson distribution. The mean rate of arrival is900 vehicles per hour. If a gap is defined as the timedifference between two successive vehicle arrivals (with vehicles assumed to be points), the probability (up to four decimal places) that the gap is greater than 8 seconds is__a)0.1354b)0.164c)0.174d)noneCorrect answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Vehicles arriving at an intersection from one of the approach road follow the Poisson distribution. The mean rate of arrival is900 vehicles per hour. If a gap is defined as the timedifference between two successive vehicle arrivals (with vehicles assumed to be points), the probability (up to four decimal places) that the gap is greater than 8 seconds is__a)0.1354b)0.164c)0.174d)noneCorrect answer is option 'A'. Can you explain this answer? tests, examples and also practice GATE tests.

|
Explore Courses for GATE exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















