All Exams >
UPSC >
CSAT Preparation >
All Questions
All questions of Quadratic Equations for UPSC CSE Exam
I. x2 + 5x + 6 = 0,
II. y2 + 9y +14 = 0 to solve both the equations to find the values of x and y?
- a)If x < y
- b)If x > y
- c)If x ≤ y
- d)If x = y or the relationship between x and y cannot be established.
- e)If x ≥ y
Correct answer is option 'D'. Can you explain this answer?
I. x2 + 5x + 6 = 0,
II. y2 + 9y +14 = 0 to solve both the equations to find the values of x and y?
II. y2 + 9y +14 = 0 to solve both the equations to find the values of x and y?
a)
If x < y
b)
If x > y
c)
If x ≤ y
d)
If x = y or the relationship between x and y cannot be established.
e)
If x ≥ y
|
Kavya Saxena answered |
I. x2 + 3x + 2x + 6 = 0
=> (x + 3)(x + 2) = 0 => x = -3 or -2
II. y2 + 7y + 2y + 14 = 0
=> (y + 7)(y + 2) = 0 => y = -7 or -2
No relationship can be established between x and y.
=> (x + 3)(x + 2) = 0 => x = -3 or -2
II. y2 + 7y + 2y + 14 = 0
=> (y + 7)(y + 2) = 0 => y = -7 or -2
No relationship can be established between x and y.
Practice Quiz or MCQ (Multiple Choice Questions) with solutions are available for Practice, which would help you prepare for "Quadratic Equations" under Quantitative Aptitude. You can practice these practice quizzes as per your speed and improvise the topic. The same topic is covered under various competitive examinations like - CAT, GMAT, Bank PO, SSC and other competitive examinations. Q. Find the roots of the quadratic equation: x2 + 2x - 15 = 0?- a)-5, 3
- b)3, 5
- c)-3, 5
- d) -3, -5
- e)5, 2
Correct answer is option 'A'. Can you explain this answer?
Practice Quiz or MCQ (Multiple Choice Questions) with solutions are available for Practice, which would help you prepare for "Quadratic Equations" under Quantitative Aptitude. You can practice these practice quizzes as per your speed and improvise the topic. The same topic is covered under various competitive examinations like - CAT, GMAT, Bank PO, SSC and other competitive examinations.
Q. Find the roots of the quadratic equation: x2 + 2x - 15 = 0?
a)
-5, 3
b)
3, 5
c)
-3, 5
d)
-3, -5
e)
5, 2
|
Manoj Ghosh answered |
Rules for factorising:
ax^2 + bx + c = 0
m x n = c
m + n = b
∴(x+m)(x+n)=0
In this case: 5 x −3 = −15 = c
5 + −3 = 2 = b
∴ x^2 + 2x −15 = 0 → (x+5)(x−3) = 0
Either of the brackets must be equal to 0.
Assuming (x+5) = 0:
x = −5
Assuming (x-3) = 0:
x= 3
I. a2 - 2a - 8 = 0,
II. b2 = 9 to solve both the equations to find the values of a and b?- a)If a < b
- b)If a ≤ b
- c)If the relationship between a and b cannot be established
- d)If a > b
- e)If a ≥ b
Correct answer is option 'C'. Can you explain this answer?
I. a2 - 2a - 8 = 0,
II. b2 = 9 to solve both the equations to find the values of a and b?
II. b2 = 9 to solve both the equations to find the values of a and b?
a)
If a < b
b)
If a ≤ b
c)
If the relationship between a and b cannot be established
d)
If a > b
e)
If a ≥ b
|
|
Kavya Saxena answered |
Explanation:
I. (a - 4)(a + 2) = 0
=> a = 4, -2
II. b2 = 9
=> b = ± 3
-2 < 3, -2 > -3, 4 > 3, 4 > -3,
No relation can be established between a and b.
=> a = 4, -2
II. b2 = 9
=> b = ± 3
-2 < 3, -2 > -3, 4 > 3, 4 > -3,
No relation can be established between a and b.
The sum and the product of the roots of the quadratic equation x2 + 20x + 3 = 0 are?- a)10, 3
- b) -10, 3
- c)20, -3
- d)-10, -3
- e)None of these
Correct answer is option 'E'. Can you explain this answer?
The sum and the product of the roots of the quadratic equation x2 + 20x + 3 = 0 are?
a)
10, 3
b)
-10, 3
c)
20, -3
d)
-10, -3
e)
None of these
|
Niharika Dey answered |
Explanation:
Sum of the roots and the product of the roots are -20 and 3 respectively.
I. a2 + 11a + 30 = 0,
II. b2 + 6b + 5 = 0 to solve both the equations to find the values of a and b?- a)If a < b
- b)If a ≤ b
- c)If the relationship between a and b cannot be established
- d)If a > b
- e)If a ≥ b
Correct answer is option 'B'. Can you explain this answer?
I. a2 + 11a + 30 = 0,
II. b2 + 6b + 5 = 0 to solve both the equations to find the values of a and b?
II. b2 + 6b + 5 = 0 to solve both the equations to find the values of a and b?
a)
If a < b
b)
If a ≤ b
c)
If the relationship between a and b cannot be established
d)
If a > b
e)
If a ≥ b
|
Yash Patel answered |
Explanation:
I. (a + 6)(a + 5) = 0
=> a = -6, -5
II. (b + 5)(b + 1) = 0
=> b = -5, -1 => a ≤ b
=> a = -6, -5
II. (b + 5)(b + 1) = 0
=> b = -5, -1 => a ≤ b
I. a2 - 9a + 20 = 0,
II. 2b2 - 5b - 12 = 0 to solve both the equations to find the values of a and b?- a)If a < b
- b)If a ≤ b
- c)If the relationship between a and b cannot be established
- d)If a > b
- e)If a ≥ b
Correct answer is option 'E'. Can you explain this answer?
I. a2 - 9a + 20 = 0,
II. 2b2 - 5b - 12 = 0 to solve both the equations to find the values of a and b?
II. 2b2 - 5b - 12 = 0 to solve both the equations to find the values of a and b?
a)
If a < b
b)
If a ≤ b
c)
If the relationship between a and b cannot be established
d)
If a > b
e)
If a ≥ b
|
Anaya Patel answered |
Explanation:
I. (a - 5)(a - 4) = 0
=> a = 5, 4
II. (2b + 3)(b - 4) = 0
=> b = 4, -3/2 => a ≥ b
=> a = 5, 4
II. (2b + 3)(b - 4) = 0
=> b = 4, -3/2 => a ≥ b
Find the quadratic equations whose roots are the reciprocals of the roots of 2x2 + 5x + 3 = 0?- a)3x2 + 5x - 2 = 0
- b)3x2 + 5x + 2 = 0
- c)3x2 - 5x + 2 = 0
- d)3x2 - 5x - 2 = 0
- e)None of these
Correct answer is option 'B'. Can you explain this answer?
Find the quadratic equations whose roots are the reciprocals of the roots of 2x2 + 5x + 3 = 0?
a)
3x2 + 5x - 2 = 0
b)
3x2 + 5x + 2 = 0
c)
3x2 - 5x + 2 = 0
d)
3x2 - 5x - 2 = 0
e)
None of these
|
|
Yash Patel answered |
Explanation:
The quadratic equation whose roots are reciprocal of 2x2 + 5x + 3 = 0 can be obtained by replacing x by 1/x.
Hence, 2(1/x)2 + 5(1/x) + 3 = 0
=> 3x2 + 5x + 2 = 0
Hence, 2(1/x)2 + 5(1/x) + 3 = 0
=> 3x2 + 5x + 2 = 0
The roots of the equation 3x2 - 12x + 10 = 0 are?- a)rational and unequal
- b) complex
- c)real and equal
- d)irrational and unequal
- e)rational and equal
Correct answer is option 'D'. Can you explain this answer?
The roots of the equation 3x2 - 12x + 10 = 0 are?
a)
rational and unequal
b)
complex
c)
real and equal
d)
irrational and unequal
e)
rational and equal
|
Gowri Chakraborty answered |
The discriminant of the quadratic equation is (-12)2 - 4(3)(10) i.e., 24. As this is positive but not a perfect square, the roots are irrational and unequal.
A man could buy a certain number of notebooks for Rs.300. If each notebook cost is Rs.5 more, he could have bought 10 notebooks less for the same amount. Find the price of each notebook?- a)10
- b)8
- c)15
- d)7.50
- e)None of these
Correct answer is option 'A'. Can you explain this answer?
A man could buy a certain number of notebooks for Rs.300. If each notebook cost is Rs.5 more, he could have bought 10 notebooks less for the same amount. Find the price of each notebook?
a)
10
b)
8
c)
15
d)
7.50
e)
None of these
|
Nikita Singh answered |
Explanation:
Let the price of each note book be Rs.x.
Let the number of note books which can be brought for Rs.300 each at a price of Rs.x be y.
Hence xy = 300
=> y = 300/x
(x + 5)(y - 10) = 300 => xy + 5y - 10x - 50 = xy
=>5(300/x) - 10x - 50 = 0 => -150 + x2 + 5x = 0
multiplying both sides by -1/10x
=> x2 + 15x - 10x - 150 = 0
=> x(x + 15) - 10(x + 15) = 0
=> x = 10 or -15
As x>0, x = 10.
Let the number of note books which can be brought for Rs.300 each at a price of Rs.x be y.
Hence xy = 300
=> y = 300/x
(x + 5)(y - 10) = 300 => xy + 5y - 10x - 50 = xy
=>5(300/x) - 10x - 50 = 0 => -150 + x2 + 5x = 0
multiplying both sides by -1/10x
=> x2 + 15x - 10x - 150 = 0
=> x(x + 15) - 10(x + 15) = 0
=> x = 10 or -15
As x>0, x = 10.
The sum of the squares of two consecutive positive integers exceeds their product by 91. Find the integers?- a)9, 10
- b) 10, 11
- c)11, 12
- d)12, 13
- e)None of these
Correct answer is option 'A'. Can you explain this answer?
The sum of the squares of two consecutive positive integers exceeds their product by 91. Find the integers?
a)
9, 10
b)
10, 11
c)
11, 12
d)
12, 13
e)
None of these
|
Dhruv Mehra answered |
Let the two consecutive positive integers be x and x + 1
x2 + (x + 1)2 - x(x + 1) = 91
x2 + x - 90 = 0
(x + 10)(x - 9) = 0 => x = -10 or 9.
As x is positive x = 9
Hence the two consecutive positive integers are 9 and 10.
The angry Arjun carried some arrows for fighting with Bheeshma. With half the arrows, he cut down the arrows thrown by Bheeshma on him and with six other arrows he killed the rath driver of Bheeshma. With one arrow each he knocked down respectively the rath, flag and bow of Bheeshma. Finally with one more than four times the square root of arrows he laid Bheeshma unconscious on an arrow bed. The total number of arrows that Arjun had is- a)80
- b)100
- c)96
- d)120
Correct answer is option 'B'. Can you explain this answer?
The angry Arjun carried some arrows for fighting with Bheeshma. With half the arrows, he cut down the arrows thrown by Bheeshma on him and with six other arrows he killed the rath driver of Bheeshma. With one arrow each he knocked down respectively the rath, flag and bow of Bheeshma. Finally with one more than four times the square root of arrows he laid Bheeshma unconscious on an arrow bed. The total number of arrows that Arjun had is
a)
80
b)
100
c)
96
d)
120
|
Prateek Gupta answered |
Therefore, Arjun had 100 arrows.
Find the value of a/b + b/a, if a and b are the roots of the quadratic equation x2 + 8x + 4 = 0?- a)15
- b)14
- c)24
- d)26
- e)None of these
Correct answer is option 'B'. Can you explain this answer?
Find the value of a/b + b/a, if a and b are the roots of the quadratic equation x2 + 8x + 4 = 0?
a)
15
b)
14
c)
24
d)
26
e)
None of these
|
|
Manoj Ghosh answered |
a/b + b/a = (a2 + b2)/ab = (a2 + b2 + a + b)/ab
= [(a + b)2 - 2ab]/ab
a + b = -8/1 = -8
ab = 4/1 = 4
Hence a/b + b/a = [(-8)2 - 2(4)]/4 = 56/4 = 14.
In a cricket match Kumble took three wickets less than twice the number of wickets taken by Srinath. The product of the number of wickets taken by these two is 20, then the number of wickets taken by Kumble is- a)2
- b)4
- c)10
- d)5
Correct answer is option 'D'. Can you explain this answer?
In a cricket match Kumble took three wickets less than twice the number of wickets taken by Srinath. The product of the number of wickets taken by these two is 20, then the number of wickets taken by Kumble is
a)
2
b)
4
c)
10
d)
5
|
|
Prateek Gupta answered |
Explanation:
Let the number of wickets taken by Srinath be x then the number of wickets taken by Kumble will be 2x−3
According to question, x(2x−3)=20
According to question, x(2x−3)=20
I. a2 - 7a + 12 = 0,
II. b2 - 3b + 2 = 0 to solve both the equations to find the values of a and b?- a)if a < b
- b)if a ≤ b
- c)if the relationship between a and b cannot be established.
- d)if a > b
- e) if a ≥ b
Correct answer is option 'D'. Can you explain this answer?
I. a2 - 7a + 12 = 0,
II. b2 - 3b + 2 = 0 to solve both the equations to find the values of a and b?
II. b2 - 3b + 2 = 0 to solve both the equations to find the values of a and b?
a)
if a < b
b)
if a ≤ b
c)
if the relationship between a and b cannot be established.
d)
if a > b
e)
if a ≥ b
|
|
Gowri Chakraborty answered |
I.(a - 3)(a - 4) = 0
=> a = 3, 4
II. (b - 2)(b - 1) = 0
=> b = 1, 2
=> a > b
One root of the quadratic equation x2 - 12x + a = 0, is thrice the other. Find the value of a?- a)29
- b)-27
- c)28
- d)7
- e)None of these
Correct answer is option 'E'. Can you explain this answer?
One root of the quadratic equation x2 - 12x + a = 0, is thrice the other. Find the value of a?
a)
29
b)
-27
c)
28
d)
7
e)
None of these
|
|
Surbhi Sen answered |
Explanation:
Let the roots of the quadratic equation be x and 3x.
Sum of roots = -(-12) = 12
a + 3a = 4a = 12 => a = 3
Product of the roots = 3a2 = 3(3)2 = 27.
Sum of roots = -(-12) = 12
a + 3a = 4a = 12 => a = 3
Product of the roots = 3a2 = 3(3)2 = 27.
I. a2 + 8a + 16 = 0,
II. b2 - 4b + 3 = 0 to solve both the equations to find the values of a and b?- a)If a < b
- b)If a ≤ b
- c)If the relationship between a and b cannot be established
- d)If a > b
- e)If a ≥ b
Correct answer is option 'A'. Can you explain this answer?
I. a2 + 8a + 16 = 0,
II. b2 - 4b + 3 = 0 to solve both the equations to find the values of a and b?
II. b2 - 4b + 3 = 0 to solve both the equations to find the values of a and b?
a)
If a < b
b)
If a ≤ b
c)
If the relationship between a and b cannot be established
d)
If a > b
e)
If a ≥ b
|
Sagar Sharma answered |
I. To solve the equation a^2 + 8a + 16 = 0, we can use the quadratic formula:
a = (-b ± √(b^2 - 4ac)) / (2a)
In this case, a = 1, b = 8, and c = 16. Plugging these values into the quadratic formula:
a = (-8 ± √(8^2 - 4(1)(16))) / (2(1))
Simplifying:
a = (-8 ± √(64 - 64)) / 2
a = (-8 ± √0) / 2
a = -8 / 2
a = -4
So, the value of a is -4.
II. To solve the equation b^2 - 4b + 3 = 0, we can factorize it:
(b - 1)(b - 3) = 0
Setting each factor equal to zero:
b - 1 = 0 or b - 3 = 0
b = 1 or b = 3
So, the values of b are 1 and 3.
Therefore, the values of a and b are -4, 1, and 3.
a = (-b ± √(b^2 - 4ac)) / (2a)
In this case, a = 1, b = 8, and c = 16. Plugging these values into the quadratic formula:
a = (-8 ± √(8^2 - 4(1)(16))) / (2(1))
Simplifying:
a = (-8 ± √(64 - 64)) / 2
a = (-8 ± √0) / 2
a = -8 / 2
a = -4
So, the value of a is -4.
II. To solve the equation b^2 - 4b + 3 = 0, we can factorize it:
(b - 1)(b - 3) = 0
Setting each factor equal to zero:
b - 1 = 0 or b - 3 = 0
b = 1 or b = 3
So, the values of b are 1 and 3.
Therefore, the values of a and b are -4, 1, and 3.
If the roots of a quadratic equation are 20 and -7, then find the equation?- a)x2 + 13x - 140 = 0
- b)x2 - 13x + 140 = 0
- c)x2 - 13x - 140 = 0
- d)x2 + 13x + 140 = 0
- e)None of these
Correct answer is option 'C'. Can you explain this answer?
If the roots of a quadratic equation are 20 and -7, then find the equation?
a)
x2 + 13x - 140 = 0
b)
x2 - 13x + 140 = 0
c)
x2 - 13x - 140 = 0
d)
x2 + 13x + 140 = 0
e)
None of these
|
|
Deepika Banerjee answered |
Explanation:
Any quadratic equation is of the form
x2 - (sum of the roots)x + (product of the roots) = 0 ---- (1)
where x is a real variable. As sum of the roots is 13 and product of the roots is -140, the quadratic equation with roots as 20 and -7 is: x2 - 13x - 140 = 0.
x2 - (sum of the roots)x + (product of the roots) = 0 ---- (1)
where x is a real variable. As sum of the roots is 13 and product of the roots is -140, the quadratic equation with roots as 20 and -7 is: x2 - 13x - 140 = 0.
The two numbers whose sum is 27 and their product is 182 are- a)12 and 13
- b)12 and 15
- c)14 and 15
- d)13 and 14
Correct answer is option 'D'. Can you explain this answer?
The two numbers whose sum is 27 and their product is 182 are
a)
12 and 13
b)
12 and 15
c)
14 and 15
d)
13 and 14
|
|
Prateek Gupta answered |
Explanation:Let the one number be xx .As the sum of numbers is 27 , then the other number will be (27−x)(27−x) According to question
If the roots of the equation 2x2 - 5x + b = 0 are in the ratio of 2:3, then find the value of b?- a)3
- b)4
- c)5
- d)6
- e)None of these
Correct answer is option 'A'. Can you explain this answer?
If the roots of the equation 2x2 - 5x + b = 0 are in the ratio of 2:3, then find the value of b?
a)
3
b)
4
c)
5
d)
6
e)
None of these
|
Sounak Malik answered |
Let the roots of the equation 2a and 3a respectively.
2a + 3a = 5a = -(- 5/2) = 5/2 => a = 1/2
Product of the roots: 6a2 = b/2 => b = 12a2
a = 1/2, b = 3.
The common root of 2x2+x−6 = 0 and x2−3x−10 = 0 is
- a)2
- b)5
- c)-2
- d)3/2
Correct answer is option 'C'. Can you explain this answer?
The common root of 2x2+x−6 = 0 and x2−3x−10 = 0 is
a)
2
b)
5
c)
-2
d)
3/2
|
|
Chirag Sen answered |
The common root of 2x^2 and x is x.
For all x, x2 + 2ax + (10 − 3a) > 0, then the interval in which a lies, is?
- a)a < -5
- b)a > 5
- c)-5 < a < 2
- d)2 < a < 5
- e)a < -2
Correct answer is option 'C'. Can you explain this answer?
For all x, x2 + 2ax + (10 − 3a) > 0, then the interval in which a lies, is?
a)
a < -5
b)
a > 5
c)
-5 < a < 2
d)
2 < a < 5
e)
a < -2
|
Rahul Mehta answered |
In f(x) = ax2 + bx + c
When a > 0 and D < 0
Then f(x) is always positive.
x2 + 2ax + 10 − 3a > 0, ∀x ∈ R
⇒ D < 0
⇒ 4a2 − 4(10 − 3a) < 0
⇒ a2 + 3a − 10 < 0
⇒ (a+5)(a−2) < 0
⇒ a ∈ (−5,2)
When a > 0 and D < 0
Then f(x) is always positive.
x2 + 2ax + 10 − 3a > 0, ∀x ∈ R
⇒ D < 0
⇒ 4a2 − 4(10 − 3a) < 0
⇒ a2 + 3a − 10 < 0
⇒ (a+5)(a−2) < 0
⇒ a ∈ (−5,2)
If the roots of the equation (a2 + b2)x2 − 2b(a + c)x + (b2+c2) = 0 are equal then
- a)2b = ac
- b)b2 = ac
- c)b = 2ac/(a + c)
- d)b = ac
- e)b = 2ac
Correct answer is option 'B'. Can you explain this answer?
If the roots of the equation (a2 + b2)x2 − 2b(a + c)x + (b2+c2) = 0 are equal then
a)
2b = ac
b)
b2 = ac
c)
b = 2ac/(a + c)
d)
b = ac
e)
b = 2ac
|
|
Ritika Choudhury answered |
(a2 + b2)x2 − 2b(a + c)x + (b2+c2) = 0
Roots are real and equal ∴ D = 0
D = b2 − 4ac = 0
⇒ [−2b(a+c)]2 − 4(a2 + b2)(b2 + c2) = 0
⇒ b2(a2 + c2 + 2ac) −(a2b2 + a2c2 + b4 + c2c2) = 0
⇒ b2a2 + b2c2 + 2acb2 − a2b2 − a2c2 − b4 − b2c2 = 0
⇒ 2acb2 − a2c2 − 2acb2 = 0
⇒ (b2 − ac)2 = 0
⇒ b2 = ac
Roots are real and equal ∴ D = 0
D = b2 − 4ac = 0
⇒ [−2b(a+c)]2 − 4(a2 + b2)(b2 + c2) = 0
⇒ b2(a2 + c2 + 2ac) −(a2b2 + a2c2 + b4 + c2c2) = 0
⇒ b2a2 + b2c2 + 2acb2 − a2b2 − a2c2 − b4 − b2c2 = 0
⇒ 2acb2 − a2c2 − 2acb2 = 0
⇒ (b2 − ac)2 = 0
⇒ b2 = ac
The product of two successive integral multiples of 5 is 1050. Then the numbers are- a)35 and 40
- b)25 and 30
- c)25 and 42
- d)30 and 35
Correct answer is option 'D'. Can you explain this answer?
The product of two successive integral multiples of 5 is 1050. Then the numbers are
a)
35 and 40
b)
25 and 30
c)
25 and 42
d)
30 and 35
|
|
Tanishq Yadav answered |
The problem:
The product of two successive integral multiples of 5 is 1050. We need to find these two numbers.
Approach:
Let's assume the two numbers as (5x) and (5x + 5), where x is an integer. We can form an equation based on the given information and solve for x.
Solution:
Let's form the equation based on the given information:
(5x) * (5x + 5) = 1050
Expanding the equation:
25x^2 + 25x = 1050
Simplifying the equation:
25x^2 + 25x - 1050 = 0
Factoring the equation:
25(x^2 + x - 42) = 0
Further simplification:
(x^2 + x - 42) = 0
Factoring the quadratic equation:
(x + 7)(x - 6) = 0
Solving for x:
x + 7 = 0 or x - 6 = 0
If x + 7 = 0, then x = -7
If x - 6 = 0, then x = 6
Since we are looking for positive integers, we can discard the negative value of x.
Calculating the numbers:
Using the value of x, we can find the two numbers:
First number = 5x = 5 * 6 = 30
Second number = 5x + 5 = 5 * 6 + 5 = 35
Thus, the two successive integral multiples of 5 that have a product of 1050 are 30 and 35.
Final Answer:
The correct answer is option D, which states that the numbers are 30 and 35.
The product of two successive integral multiples of 5 is 1050. We need to find these two numbers.
Approach:
Let's assume the two numbers as (5x) and (5x + 5), where x is an integer. We can form an equation based on the given information and solve for x.
Solution:
Let's form the equation based on the given information:
(5x) * (5x + 5) = 1050
Expanding the equation:
25x^2 + 25x = 1050
Simplifying the equation:
25x^2 + 25x - 1050 = 0
Factoring the equation:
25(x^2 + x - 42) = 0
Further simplification:
(x^2 + x - 42) = 0
Factoring the quadratic equation:
(x + 7)(x - 6) = 0
Solving for x:
x + 7 = 0 or x - 6 = 0
If x + 7 = 0, then x = -7
If x - 6 = 0, then x = 6
Since we are looking for positive integers, we can discard the negative value of x.
Calculating the numbers:
Using the value of x, we can find the two numbers:
First number = 5x = 5 * 6 = 30
Second number = 5x + 5 = 5 * 6 + 5 = 35
Thus, the two successive integral multiples of 5 that have a product of 1050 are 30 and 35.
Final Answer:
The correct answer is option D, which states that the numbers are 30 and 35.
by the method of completing the square is
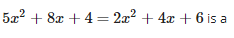
- a)quadratic equation
- b)constant
- c)cubic equation
- d)linear equation
Correct answer is option 'A'. Can you explain this answer?
a)
quadratic equation
b)
constant
c)
cubic equation
d)
linear equation
|
|
Prateek Gupta answered |
Here, the degree is 2, therefore it is a quadratic equation.
Chapter doubts & questions for Quadratic Equations - CSAT Preparation 2025 is part of UPSC CSE exam preparation. The chapters have been prepared according to the UPSC CSE exam syllabus. The Chapter doubts & questions, notes, tests & MCQs are made for UPSC CSE 2025 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests here.
Chapter doubts & questions of Quadratic Equations - CSAT Preparation in English & Hindi are available as part of UPSC CSE exam.
Download more important topics, notes, lectures and mock test series for UPSC CSE Exam by signing up for free.
CSAT Preparation
207 videos|249 docs|138 tests
|
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup