GATE Exam > GATE Questions > Consider the propagation of EM waves dilute i...
Start Learning for Free
Consider the propagation of EM waves dilute ionised gases such as the ionosphere. If the electron density is
1022/m3. The frequency of the wave that can passing through the gases is ____x 1010 Hz (your answer
should be an integer)
1022/m3. The frequency of the wave that can passing through the gases is ____x 1010 Hz (your answer
should be an integer)
Correct answer is '90'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
Consider the propagation of EM waves dilute ionised gases such as the ...
We know that the ciitical frequency.
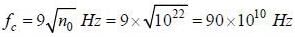
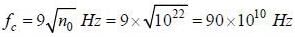
Most Upvoted Answer
Consider the propagation of EM waves dilute ionised gases such as the ...
Propagation of EM Waves in Dilute Ionised Gases
In the ionosphere, which is a layer of the Earth's atmosphere, the presence of ionized gases affects the propagation of electromagnetic (EM) waves. The electron density in the ionosphere plays a crucial role in determining the frequency of waves that can pass through the gases.
Frequency Calculation
To calculate the frequency of the wave that can pass through the dilute ionized gases, we can use the plasma frequency formula:
fp = √(ne * e^2 / (ε0 * me))
where:
fp is the plasma frequency,
ne is the electron density,
e is the elementary charge,
ε0 is the permittivity of free space,
me is the mass of an electron.
Given that the electron density is 1022/m^3, we can substitute this value into the formula:
fp = √(1022 * (1.6 x 10^-19)^2 / (8.85 x 10^-12 * 9.11 x 10^-31))
Simplifying the expression:
fp ≈ √(1022 * 2.56 x 10^-38 / (8.85 x 10^-12 * 9.11 x 10^-31))
fp ≈ √(2.9312 x 10^-16 / 8.08535 x 10^-43)
fp ≈ √(3.6235 x 10^27)
fp ≈ 6.02 x 10^13 Hz
Therefore, the calculated plasma frequency is approximately 6.02 x 10^13 Hz.
Limiting Frequency
The limiting frequency is the maximum frequency that can pass through the ionized gases without being completely absorbed. It is given by:
fL = fp / (2π)
Substituting the calculated plasma frequency:
fL ≈ (6.02 x 10^13) / (2π)
fL ≈ 9.58 x 10^12 Hz
Therefore, the limiting frequency is approximately 9.58 x 10^12 Hz.
Conclusion
The frequency of the wave that can pass through the dilute ionized gases, such as the ionosphere, is determined by the plasma frequency, which depends on the electron density. In this case, with an electron density of 1022/m^3, the frequency is approximately 6.02 x 10^13 Hz. However, the limiting frequency, which is the maximum frequency that can pass through without being absorbed, is approximately 9.58 x 10^12 Hz. Therefore, the correct answer is '90' (given in scientific notation as 9 x 10^10 Hz).
In the ionosphere, which is a layer of the Earth's atmosphere, the presence of ionized gases affects the propagation of electromagnetic (EM) waves. The electron density in the ionosphere plays a crucial role in determining the frequency of waves that can pass through the gases.
Frequency Calculation
To calculate the frequency of the wave that can pass through the dilute ionized gases, we can use the plasma frequency formula:
fp = √(ne * e^2 / (ε0 * me))
where:
fp is the plasma frequency,
ne is the electron density,
e is the elementary charge,
ε0 is the permittivity of free space,
me is the mass of an electron.
Given that the electron density is 1022/m^3, we can substitute this value into the formula:
fp = √(1022 * (1.6 x 10^-19)^2 / (8.85 x 10^-12 * 9.11 x 10^-31))
Simplifying the expression:
fp ≈ √(1022 * 2.56 x 10^-38 / (8.85 x 10^-12 * 9.11 x 10^-31))
fp ≈ √(2.9312 x 10^-16 / 8.08535 x 10^-43)
fp ≈ √(3.6235 x 10^27)
fp ≈ 6.02 x 10^13 Hz
Therefore, the calculated plasma frequency is approximately 6.02 x 10^13 Hz.
Limiting Frequency
The limiting frequency is the maximum frequency that can pass through the ionized gases without being completely absorbed. It is given by:
fL = fp / (2π)
Substituting the calculated plasma frequency:
fL ≈ (6.02 x 10^13) / (2π)
fL ≈ 9.58 x 10^12 Hz
Therefore, the limiting frequency is approximately 9.58 x 10^12 Hz.
Conclusion
The frequency of the wave that can pass through the dilute ionized gases, such as the ionosphere, is determined by the plasma frequency, which depends on the electron density. In this case, with an electron density of 1022/m^3, the frequency is approximately 6.02 x 10^13 Hz. However, the limiting frequency, which is the maximum frequency that can pass through without being absorbed, is approximately 9.58 x 10^12 Hz. Therefore, the correct answer is '90' (given in scientific notation as 9 x 10^10 Hz).

|
Explore Courses for GATE exam
|

|
Consider the propagation of EM waves dilute ionised gases such as the ionosphere. If the electron density is1022/m3. The frequency of the wave that can passing through the gases is ____x 1010 Hz (your answershould be an integer)Correct answer is '90'. Can you explain this answer?
Question Description
Consider the propagation of EM waves dilute ionised gases such as the ionosphere. If the electron density is1022/m3. The frequency of the wave that can passing through the gases is ____x 1010 Hz (your answershould be an integer)Correct answer is '90'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about Consider the propagation of EM waves dilute ionised gases such as the ionosphere. If the electron density is1022/m3. The frequency of the wave that can passing through the gases is ____x 1010 Hz (your answershould be an integer)Correct answer is '90'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Consider the propagation of EM waves dilute ionised gases such as the ionosphere. If the electron density is1022/m3. The frequency of the wave that can passing through the gases is ____x 1010 Hz (your answershould be an integer)Correct answer is '90'. Can you explain this answer?.
Consider the propagation of EM waves dilute ionised gases such as the ionosphere. If the electron density is1022/m3. The frequency of the wave that can passing through the gases is ____x 1010 Hz (your answershould be an integer)Correct answer is '90'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about Consider the propagation of EM waves dilute ionised gases such as the ionosphere. If the electron density is1022/m3. The frequency of the wave that can passing through the gases is ____x 1010 Hz (your answershould be an integer)Correct answer is '90'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Consider the propagation of EM waves dilute ionised gases such as the ionosphere. If the electron density is1022/m3. The frequency of the wave that can passing through the gases is ____x 1010 Hz (your answershould be an integer)Correct answer is '90'. Can you explain this answer?.
Solutions for Consider the propagation of EM waves dilute ionised gases such as the ionosphere. If the electron density is1022/m3. The frequency of the wave that can passing through the gases is ____x 1010 Hz (your answershould be an integer)Correct answer is '90'. Can you explain this answer? in English & in Hindi are available as part of our courses for GATE.
Download more important topics, notes, lectures and mock test series for GATE Exam by signing up for free.
Here you can find the meaning of Consider the propagation of EM waves dilute ionised gases such as the ionosphere. If the electron density is1022/m3. The frequency of the wave that can passing through the gases is ____x 1010 Hz (your answershould be an integer)Correct answer is '90'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Consider the propagation of EM waves dilute ionised gases such as the ionosphere. If the electron density is1022/m3. The frequency of the wave that can passing through the gases is ____x 1010 Hz (your answershould be an integer)Correct answer is '90'. Can you explain this answer?, a detailed solution for Consider the propagation of EM waves dilute ionised gases such as the ionosphere. If the electron density is1022/m3. The frequency of the wave that can passing through the gases is ____x 1010 Hz (your answershould be an integer)Correct answer is '90'. Can you explain this answer? has been provided alongside types of Consider the propagation of EM waves dilute ionised gases such as the ionosphere. If the electron density is1022/m3. The frequency of the wave that can passing through the gases is ____x 1010 Hz (your answershould be an integer)Correct answer is '90'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Consider the propagation of EM waves dilute ionised gases such as the ionosphere. If the electron density is1022/m3. The frequency of the wave that can passing through the gases is ____x 1010 Hz (your answershould be an integer)Correct answer is '90'. Can you explain this answer? tests, examples and also practice GATE tests.

|
Explore Courses for GATE exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
















