GATE Exam > GATE Questions > For high principal quantum number (n) for hyd...
Start Learning for Free
For high principal quantum number (n) for hydrogen atom the spacing between the neighbouring energy level is proportional to
- a)1/n3
- b)1/n2
- c)n2
- d)n3
Correct answer is option 'A'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
For high principal quantum number (n) for hydrogen atom the spacing be...
The difference of energy between energy levels having principal quantum number n and (n + 1) is given by
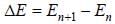
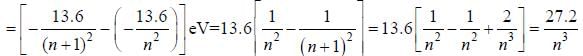
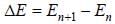
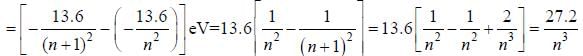
Most Upvoted Answer
For high principal quantum number (n) for hydrogen atom the spacing be...
The spacing between neighboring energy levels in a hydrogen atom is determined by the principal quantum number (n) of the energy levels. The correct answer is option A, which states that the spacing is proportional to 1/n^3.
Explanation:
1. Energy levels in a hydrogen atom:
- In a hydrogen atom, the electron is bound to the nucleus by the electrostatic attraction between the negatively charged electron and the positively charged proton.
- The electron can occupy different energy levels, which are characterized by the principal quantum number (n).
- The energy of these levels increases as the value of n increases.
2. Energy formula for hydrogen atom:
- The energy of an electron in a hydrogen atom can be calculated using the formula:
E = -13.6 eV / n^2
where E is the energy, -13.6 eV is a constant, and n is the principal quantum number.
3. Spacing between neighboring energy levels:
- The spacing between neighboring energy levels can be calculated by taking the difference in energy between two consecutive levels.
- Let's consider two energy levels with principal quantum numbers n and (n+1).
- The energy difference between these levels can be calculated by subtracting the energy of the lower level from the energy of the higher level:
ΔE = E(n+1) - E(n)
= [-13.6 eV / (n+1)^2] - [-13.6 eV / n^2]
= -13.6 eV * [1 / (n+1)^2 - 1 / n^2]
4. Simplifying the energy difference formula:
- To simplify the energy difference formula, we can use the concept of fractional differences.
- We can rewrite the energy difference formula as:
ΔE = -13.6 eV * [1 / (n+1)^2 - 1 / n^2]
= -13.6 eV * [n^2 - (n+1)^2] / [n^2 * (n+1)^2]
= -13.6 eV * [-2n - 1] / [n^2 * (n+1)^2]
5. Proportional relationship with n:
- From the simplified energy difference formula, we can observe that the spacing ΔE is proportional to (-2n - 1) / (n^2 * (n+1)^2).
- As n increases, the term (-2n - 1) becomes insignificant compared to the term (n^2 * (n+1)^2).
- Therefore, we can approximate the spacing as proportional to 1 / (n^2 * (n+1)^2).
6. Final answer:
- Since the spacing is proportional to 1 / (n^2 * (n+1)^2), the correct answer is option A: 1/n^3.
In summary, the spacing between neighboring energy levels in a hydrogen atom is proportional to 1/n^3, as the value of the principal quantum number (n) increases.
Explanation:
1. Energy levels in a hydrogen atom:
- In a hydrogen atom, the electron is bound to the nucleus by the electrostatic attraction between the negatively charged electron and the positively charged proton.
- The electron can occupy different energy levels, which are characterized by the principal quantum number (n).
- The energy of these levels increases as the value of n increases.
2. Energy formula for hydrogen atom:
- The energy of an electron in a hydrogen atom can be calculated using the formula:
E = -13.6 eV / n^2
where E is the energy, -13.6 eV is a constant, and n is the principal quantum number.
3. Spacing between neighboring energy levels:
- The spacing between neighboring energy levels can be calculated by taking the difference in energy between two consecutive levels.
- Let's consider two energy levels with principal quantum numbers n and (n+1).
- The energy difference between these levels can be calculated by subtracting the energy of the lower level from the energy of the higher level:
ΔE = E(n+1) - E(n)
= [-13.6 eV / (n+1)^2] - [-13.6 eV / n^2]
= -13.6 eV * [1 / (n+1)^2 - 1 / n^2]
4. Simplifying the energy difference formula:
- To simplify the energy difference formula, we can use the concept of fractional differences.
- We can rewrite the energy difference formula as:
ΔE = -13.6 eV * [1 / (n+1)^2 - 1 / n^2]
= -13.6 eV * [n^2 - (n+1)^2] / [n^2 * (n+1)^2]
= -13.6 eV * [-2n - 1] / [n^2 * (n+1)^2]
5. Proportional relationship with n:
- From the simplified energy difference formula, we can observe that the spacing ΔE is proportional to (-2n - 1) / (n^2 * (n+1)^2).
- As n increases, the term (-2n - 1) becomes insignificant compared to the term (n^2 * (n+1)^2).
- Therefore, we can approximate the spacing as proportional to 1 / (n^2 * (n+1)^2).
6. Final answer:
- Since the spacing is proportional to 1 / (n^2 * (n+1)^2), the correct answer is option A: 1/n^3.
In summary, the spacing between neighboring energy levels in a hydrogen atom is proportional to 1/n^3, as the value of the principal quantum number (n) increases.

|
Explore Courses for GATE exam
|

|
Similar GATE Doubts
For high principal quantum number (n) for hydrogen atom the spacing between the neighbouring energy level is proportional toa)1/n3b)1/n2c)n2d)n3Correct answer is option 'A'. Can you explain this answer?
Question Description
For high principal quantum number (n) for hydrogen atom the spacing between the neighbouring energy level is proportional toa)1/n3b)1/n2c)n2d)n3Correct answer is option 'A'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about For high principal quantum number (n) for hydrogen atom the spacing between the neighbouring energy level is proportional toa)1/n3b)1/n2c)n2d)n3Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for For high principal quantum number (n) for hydrogen atom the spacing between the neighbouring energy level is proportional toa)1/n3b)1/n2c)n2d)n3Correct answer is option 'A'. Can you explain this answer?.
For high principal quantum number (n) for hydrogen atom the spacing between the neighbouring energy level is proportional toa)1/n3b)1/n2c)n2d)n3Correct answer is option 'A'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about For high principal quantum number (n) for hydrogen atom the spacing between the neighbouring energy level is proportional toa)1/n3b)1/n2c)n2d)n3Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for For high principal quantum number (n) for hydrogen atom the spacing between the neighbouring energy level is proportional toa)1/n3b)1/n2c)n2d)n3Correct answer is option 'A'. Can you explain this answer?.
Solutions for For high principal quantum number (n) for hydrogen atom the spacing between the neighbouring energy level is proportional toa)1/n3b)1/n2c)n2d)n3Correct answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for GATE.
Download more important topics, notes, lectures and mock test series for GATE Exam by signing up for free.
Here you can find the meaning of For high principal quantum number (n) for hydrogen atom the spacing between the neighbouring energy level is proportional toa)1/n3b)1/n2c)n2d)n3Correct answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
For high principal quantum number (n) for hydrogen atom the spacing between the neighbouring energy level is proportional toa)1/n3b)1/n2c)n2d)n3Correct answer is option 'A'. Can you explain this answer?, a detailed solution for For high principal quantum number (n) for hydrogen atom the spacing between the neighbouring energy level is proportional toa)1/n3b)1/n2c)n2d)n3Correct answer is option 'A'. Can you explain this answer? has been provided alongside types of For high principal quantum number (n) for hydrogen atom the spacing between the neighbouring energy level is proportional toa)1/n3b)1/n2c)n2d)n3Correct answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice For high principal quantum number (n) for hydrogen atom the spacing between the neighbouring energy level is proportional toa)1/n3b)1/n2c)n2d)n3Correct answer is option 'A'. Can you explain this answer? tests, examples and also practice GATE tests.

|
Explore Courses for GATE exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















