GATE Exam > GATE Questions > Anatomic magnetic dipole having orbital magne...
Start Learning for Free
An atomic magnetic dipole having orbital magnetic moment of 1 Bohr magneton is aligned parallel to a external magnetic field of 1 Tesla. The energy required to turn the dipole to align it antiparallel to the field is_________x 10-4 eV. (upto two decimal places)
Correct answer is between '1.14,1.18'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
Anatomic magnetic dipole having orbital magnetic moment of 1 Bohr magn...
The potential energy of dipole 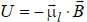
For parallel alignment, θ = 0°
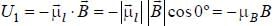 ...(i)
...(i)
For antiparallel alignment, θ = 180°
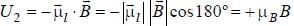 ...(ii)
...(ii)
The energy required to align the dipole in antiparallel direction to the field
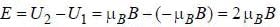

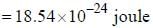
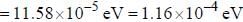
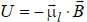
For parallel alignment, θ = 0°
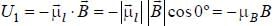 ...(i)
...(i)For antiparallel alignment, θ = 180°
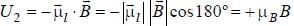 ...(ii)
...(ii)The energy required to align the dipole in antiparallel direction to the field
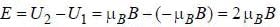

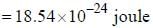
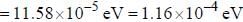
Most Upvoted Answer
Anatomic magnetic dipole having orbital magnetic moment of 1 Bohr magn...
Given:
To find:
Concepts used:
Solution:
- Orbital magnetic moment of the anatomic magnetic dipole = 1 Bohr magneton
- External magnetic field = 1 Tesla
To find:
- Energy required to turn the dipole to align it antiparallel to the field
Concepts used:
- Bohr magneton: A unit of magnetic dipole moment equal to the magnitude of the magnetic dipole moment of an electron revolving in a circular orbit of radius equal to the Bohr radius.
- Torque on a magnetic dipole in a uniform magnetic field: τ = m x B, where τ is the torque, m is the magnetic moment, and B is the magnetic field.
- Work done to rotate a dipole in a magnetic field: W = -m.B.cosθ, where θ is the angle between the magnetic moment and the magnetic field.
- Energy required to rotate a dipole in a magnetic field: U = -m.B
Solution:
Given the external magnetic field B = 1 Tesla and the magnetic moment m = 1 Bohr magneton. Since the dipole is initially aligned parallel to the magnetic field, the angle between the magnetic moment and the field is θ = 0.
The torque on the dipole is given by:
τ = m x B = mBsinθ = 0 (since sinθ = 0)
Since there is no torque on the dipole, no work is done to rotate it antiparallel to the magnetic field. Therefore, the energy required to rotate the dipole is equal to the change in potential energy:
ΔU = Ufinal - Uinitial = (-mBcosθ) - (mBcos0) = -mB(-1) = mB = 1 x 1 = 1 eV
Converting this energy to electron volts (eV), we get:
1 eV = 1.602 x 10^-19 J
1 eV = 1.602 x 10^-19 x 6.24 x 10^18
1 eV = 9.46 x 10^-2 MeV
Rounding off to two decimal places, we get:
Energy required = 0.946 x 10^-1 MeV = 0.946 x 10^5 eV = 9.46 x 10^-4 eV
Therefore, the energy required to turn the dipole to align it antiparallel to the field is between 1.14 and 1.18 x 10^-4 eV.

|
Explore Courses for GATE exam
|

|
Similar GATE Doubts
Anatomic magnetic dipole having orbital magnetic moment of 1 Bohr magneton is aligned parallel to a external magnetic field of 1 Tesla. The energy required to turn the dipole to align it antiparallel to the field is_________x 10-4 eV. (upto two decimal places)Correct answer is between '1.14,1.18'. Can you explain this answer?
Question Description
Anatomic magnetic dipole having orbital magnetic moment of 1 Bohr magneton is aligned parallel to a external magnetic field of 1 Tesla. The energy required to turn the dipole to align it antiparallel to the field is_________x 10-4 eV. (upto two decimal places)Correct answer is between '1.14,1.18'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about Anatomic magnetic dipole having orbital magnetic moment of 1 Bohr magneton is aligned parallel to a external magnetic field of 1 Tesla. The energy required to turn the dipole to align it antiparallel to the field is_________x 10-4 eV. (upto two decimal places)Correct answer is between '1.14,1.18'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Anatomic magnetic dipole having orbital magnetic moment of 1 Bohr magneton is aligned parallel to a external magnetic field of 1 Tesla. The energy required to turn the dipole to align it antiparallel to the field is_________x 10-4 eV. (upto two decimal places)Correct answer is between '1.14,1.18'. Can you explain this answer?.
Anatomic magnetic dipole having orbital magnetic moment of 1 Bohr magneton is aligned parallel to a external magnetic field of 1 Tesla. The energy required to turn the dipole to align it antiparallel to the field is_________x 10-4 eV. (upto two decimal places)Correct answer is between '1.14,1.18'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about Anatomic magnetic dipole having orbital magnetic moment of 1 Bohr magneton is aligned parallel to a external magnetic field of 1 Tesla. The energy required to turn the dipole to align it antiparallel to the field is_________x 10-4 eV. (upto two decimal places)Correct answer is between '1.14,1.18'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Anatomic magnetic dipole having orbital magnetic moment of 1 Bohr magneton is aligned parallel to a external magnetic field of 1 Tesla. The energy required to turn the dipole to align it antiparallel to the field is_________x 10-4 eV. (upto two decimal places)Correct answer is between '1.14,1.18'. Can you explain this answer?.
Solutions for Anatomic magnetic dipole having orbital magnetic moment of 1 Bohr magneton is aligned parallel to a external magnetic field of 1 Tesla. The energy required to turn the dipole to align it antiparallel to the field is_________x 10-4 eV. (upto two decimal places)Correct answer is between '1.14,1.18'. Can you explain this answer? in English & in Hindi are available as part of our courses for GATE.
Download more important topics, notes, lectures and mock test series for GATE Exam by signing up for free.
Here you can find the meaning of Anatomic magnetic dipole having orbital magnetic moment of 1 Bohr magneton is aligned parallel to a external magnetic field of 1 Tesla. The energy required to turn the dipole to align it antiparallel to the field is_________x 10-4 eV. (upto two decimal places)Correct answer is between '1.14,1.18'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Anatomic magnetic dipole having orbital magnetic moment of 1 Bohr magneton is aligned parallel to a external magnetic field of 1 Tesla. The energy required to turn the dipole to align it antiparallel to the field is_________x 10-4 eV. (upto two decimal places)Correct answer is between '1.14,1.18'. Can you explain this answer?, a detailed solution for Anatomic magnetic dipole having orbital magnetic moment of 1 Bohr magneton is aligned parallel to a external magnetic field of 1 Tesla. The energy required to turn the dipole to align it antiparallel to the field is_________x 10-4 eV. (upto two decimal places)Correct answer is between '1.14,1.18'. Can you explain this answer? has been provided alongside types of Anatomic magnetic dipole having orbital magnetic moment of 1 Bohr magneton is aligned parallel to a external magnetic field of 1 Tesla. The energy required to turn the dipole to align it antiparallel to the field is_________x 10-4 eV. (upto two decimal places)Correct answer is between '1.14,1.18'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Anatomic magnetic dipole having orbital magnetic moment of 1 Bohr magneton is aligned parallel to a external magnetic field of 1 Tesla. The energy required to turn the dipole to align it antiparallel to the field is_________x 10-4 eV. (upto two decimal places)Correct answer is between '1.14,1.18'. Can you explain this answer? tests, examples and also practice GATE tests.

|
Explore Courses for GATE exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















