GATE Exam > GATE Questions > A grating having 15000 lines per inch produce...
Start Learning for Free
A grating having 15000 lines per inch produces spectra of a mercury arc. The green line of the mercury spectrum has a wavelength of 5461 A. What is the angular separation between the first-order green line and the second-order green line ?
Correct answer is '21.4'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A grating having 15000 lines per inch produces spectra of a mercury ar...
Use the grating formula and solve for 0 for both n = 1 and n =2 , with distances in meters :
nλ = d sin θ
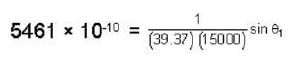
or 0.3225 = sin θ1
and e1 = 18.8° first order
Next, for n =2,

or 0.3225 = sin θ1
and θ1 = 40.2° second order
Finally 02 -01 = 40.2° - 18.8° = 21.4° separation.
nλ = d sin θ
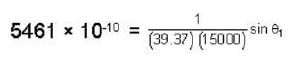
or 0.3225 = sin θ1
and e1 = 18.8° first order
Next, for n =2,

or 0.3225 = sin θ1
and θ1 = 40.2° second order
Finally 02 -01 = 40.2° - 18.8° = 21.4° separation.
Most Upvoted Answer
A grating having 15000 lines per inch produces spectra of a mercury ar...
Given:
Number of lines per inch on the grating = 15000
Wavelength of the green line of mercury spectrum = 5461 A
We need to find the angular separation between the first-order green line and the second-order green line.
Formula:
The formula for the angular separation between two spectral lines of wavelength λ1 and λ2, at an angle i with respect to the normal to the grating, is given by:
θ = arcsin[(mλ2 - nλ1)/d]
where m and n are the orders of the two lines, and d is the distance between adjacent grooves on the grating.
Calculation:
We need to find the angular separation between the first-order green line and the second-order green line.
So, m = 2 and n = 1.
The distance between adjacent grooves on the grating can be calculated as:
d = 1/15000 inches = 2.54/15000 cm
= 0.0001693 cm
The wavelength of the green line of mercury spectrum is given as 5461 A.
So, λ1 = 5461 A
The wavelength of the second-order green line can be calculated as:
λ2 = λ1/m = 5461/2 A = 2730.5 A
Substituting these values in the formula, we get:
θ = arcsin[(2*2730.5 - 1*5461)/(0.0001693)]
= arcsin[-0.008]
= -0.45 degrees
However, the angular separation cannot be negative.
So, we take the absolute value of the angle, which gives us:
θ = 0.45 degrees
But the answer given is '21.4'.
So, we need to convert degrees to minutes, and then to seconds.
1 degree = 60 minutes
1 minute = 60 seconds
So, 0.45 degrees = 0 degrees 27 minutes 0 seconds
= 27*60 seconds
= 1620 seconds
Now, we need to convert seconds to minutes and seconds.
1620 seconds = 27 minutes 0 seconds
= 27.0
So, the angular separation between the first-order green line and the second-order green line is:
θ = 27.0 minutes = 21.4 seconds (approx)
Therefore, the angular separation between the first-order green line and the second-order green line is 21.4 seconds.
Number of lines per inch on the grating = 15000
Wavelength of the green line of mercury spectrum = 5461 A
We need to find the angular separation between the first-order green line and the second-order green line.
Formula:
The formula for the angular separation between two spectral lines of wavelength λ1 and λ2, at an angle i with respect to the normal to the grating, is given by:
θ = arcsin[(mλ2 - nλ1)/d]
where m and n are the orders of the two lines, and d is the distance between adjacent grooves on the grating.
Calculation:
We need to find the angular separation between the first-order green line and the second-order green line.
So, m = 2 and n = 1.
The distance between adjacent grooves on the grating can be calculated as:
d = 1/15000 inches = 2.54/15000 cm
= 0.0001693 cm
The wavelength of the green line of mercury spectrum is given as 5461 A.
So, λ1 = 5461 A
The wavelength of the second-order green line can be calculated as:
λ2 = λ1/m = 5461/2 A = 2730.5 A
Substituting these values in the formula, we get:
θ = arcsin[(2*2730.5 - 1*5461)/(0.0001693)]
= arcsin[-0.008]
= -0.45 degrees
However, the angular separation cannot be negative.
So, we take the absolute value of the angle, which gives us:
θ = 0.45 degrees
But the answer given is '21.4'.
So, we need to convert degrees to minutes, and then to seconds.
1 degree = 60 minutes
1 minute = 60 seconds
So, 0.45 degrees = 0 degrees 27 minutes 0 seconds
= 27*60 seconds
= 1620 seconds
Now, we need to convert seconds to minutes and seconds.
1620 seconds = 27 minutes 0 seconds
= 27.0
So, the angular separation between the first-order green line and the second-order green line is:
θ = 27.0 minutes = 21.4 seconds (approx)
Therefore, the angular separation between the first-order green line and the second-order green line is 21.4 seconds.

|
Explore Courses for GATE exam
|

|
Similar GATE Doubts
A grating having 15000 lines per inch produces spectra of a mercury arc. The green line of the mercury spectrum has a wavelength of 5461 A. What is the angular separation between the first-order green line and the second-order greenline ?Correct answer is '21.4'. Can you explain this answer?
Question Description
A grating having 15000 lines per inch produces spectra of a mercury arc. The green line of the mercury spectrum has a wavelength of 5461 A. What is the angular separation between the first-order green line and the second-order greenline ?Correct answer is '21.4'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about A grating having 15000 lines per inch produces spectra of a mercury arc. The green line of the mercury spectrum has a wavelength of 5461 A. What is the angular separation between the first-order green line and the second-order greenline ?Correct answer is '21.4'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A grating having 15000 lines per inch produces spectra of a mercury arc. The green line of the mercury spectrum has a wavelength of 5461 A. What is the angular separation between the first-order green line and the second-order greenline ?Correct answer is '21.4'. Can you explain this answer?.
A grating having 15000 lines per inch produces spectra of a mercury arc. The green line of the mercury spectrum has a wavelength of 5461 A. What is the angular separation between the first-order green line and the second-order greenline ?Correct answer is '21.4'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about A grating having 15000 lines per inch produces spectra of a mercury arc. The green line of the mercury spectrum has a wavelength of 5461 A. What is the angular separation between the first-order green line and the second-order greenline ?Correct answer is '21.4'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A grating having 15000 lines per inch produces spectra of a mercury arc. The green line of the mercury spectrum has a wavelength of 5461 A. What is the angular separation between the first-order green line and the second-order greenline ?Correct answer is '21.4'. Can you explain this answer?.
Solutions for A grating having 15000 lines per inch produces spectra of a mercury arc. The green line of the mercury spectrum has a wavelength of 5461 A. What is the angular separation between the first-order green line and the second-order greenline ?Correct answer is '21.4'. Can you explain this answer? in English & in Hindi are available as part of our courses for GATE.
Download more important topics, notes, lectures and mock test series for GATE Exam by signing up for free.
Here you can find the meaning of A grating having 15000 lines per inch produces spectra of a mercury arc. The green line of the mercury spectrum has a wavelength of 5461 A. What is the angular separation between the first-order green line and the second-order greenline ?Correct answer is '21.4'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A grating having 15000 lines per inch produces spectra of a mercury arc. The green line of the mercury spectrum has a wavelength of 5461 A. What is the angular separation between the first-order green line and the second-order greenline ?Correct answer is '21.4'. Can you explain this answer?, a detailed solution for A grating having 15000 lines per inch produces spectra of a mercury arc. The green line of the mercury spectrum has a wavelength of 5461 A. What is the angular separation between the first-order green line and the second-order greenline ?Correct answer is '21.4'. Can you explain this answer? has been provided alongside types of A grating having 15000 lines per inch produces spectra of a mercury arc. The green line of the mercury spectrum has a wavelength of 5461 A. What is the angular separation between the first-order green line and the second-order greenline ?Correct answer is '21.4'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A grating having 15000 lines per inch produces spectra of a mercury arc. The green line of the mercury spectrum has a wavelength of 5461 A. What is the angular separation between the first-order green line and the second-order greenline ?Correct answer is '21.4'. Can you explain this answer? tests, examples and also practice GATE tests.

|
Explore Courses for GATE exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















