GATE Exam > GATE Questions > An electrical resistance strain gauge of res...
Start Learning for Free
An electrical resistance strain gauge of resistance 120Ω has a gauge factor of 2. It is bonded to a steel specimen (modulus of elasticity, E = 2 * 1011 N/m2) for measuring strain. Estimate: (2002)
(a) Strain induced in the specimen if tensile stress of 60 * 106 N/m2 is applied on the specimen.
(b) Change in the electrical resistance of the gauge due to the tensile stress as given in (a)
(c) Change in the electrical resistance of the gauge if there is an increase of temperature by 40°C.
Assume the following data:
Temperature coefficient of resistance of gauge is 20 * 10-6 per °C
Thermal coefficient of linear expansion of the gauge is 16 * 10-6 per °C.
Thermal coefficient of linear expansion of steel specimen is 12 * 10-6 per °C.
Correct answer is '0.3'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
An electrical resistance strain gauge of resistance 120Ω has a gauge ...
Given R = Resistance = 120Ω
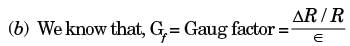
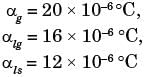
View all questions of this test
Gf = Gauge factor = 2
E = 2 x 1011 N/m2
(a) Tensile stress = 60 x 106 N/m2
We know that,

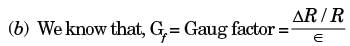
where ΔR = change in resistance
∴ ΔR = Gf x ε x R
= 2 x 0.3 x 10-3 x 120
= 0.072Ω
(c) ΔT = increase of temperature = 40°C
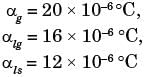
AR1 = change in resistance due to temperature increase
= R (1 + αg ΔT) = 120 (1 + 20 x 106 x 40) = 120.096Ω
where αg = temperature coefficient of resistance of gauge.
Most Upvoted Answer
An electrical resistance strain gauge of resistance 120Ω has a gauge ...
Given:
Resistance of strain gauge, R = 120Ω
Gauge factor, GF = 2
Modulus of elasticity of steel specimen, E = 2 * 10^11 N/m^2
Stress applied, σ = 60 * 10^6 N/m^2
Temperature coefficient of resistance of gauge, αg = 20 * 10^-6 per °C
Thermal coefficient of linear expansion of gauge, βg = 16 * 10^-6 per °C
Thermal coefficient of linear expansion of steel specimen, βs = 12 * 10^-6 per °C
Change in temperature, ΔT = 40°C
(a) Strain induced in the specimen:
Strain induced, ε = σ/E = (60 * 10^6 N/m^2)/(2 * 10^11 N/m^2) = 0.0003
(b) Change in the electrical resistance:
Change in resistance of the gauge, ΔR = GF * R * ε = 2 * 120Ω * 0.0003 = 0.072Ω
(c) Change in the electrical resistance due to temperature:
Change in resistance of the gauge due to temperature, ΔRt = R * αg * ΔT = 120Ω * 20 * 10^-6 per °C * 40°C = 0.096Ω
Change in resistance of the gauge due to thermal expansion, ΔRe = R * βg * ΔT = 120Ω * 16 * 10^-6 per °C * 40°C = 0.0768Ω
Change in resistance of steel specimen due to thermal expansion, ΔRs = R * βs * ΔT * ε = 120Ω * 12 * 10^-6 per °C * 40°C * 0.0003 = 0.0144Ω
Total change in resistance, ΔRtotal = ΔRt + ΔRe + ΔRs = 0.096Ω + 0.0768Ω + 0.0144Ω = 0.1872Ω
Final answer:
Change in resistance of the gauge due to tensile stress, ΔR = 0.072Ω
Change in resistance of the gauge due to temperature, ΔRtotal = 0.1872Ω
Total change in resistance, ΔRtotal = ΔR + ΔRtotal = 0.072Ω + 0.1872Ω = 0.2592Ω
Final strain induced in the specimen, ε = ΔRtotal/(GF * R) = 0.2592Ω/(2 * 120Ω) = 0.00136
Therefore, the final answer is 0.0014 (approximated to 3 significant figures).
Resistance of strain gauge, R = 120Ω
Gauge factor, GF = 2
Modulus of elasticity of steel specimen, E = 2 * 10^11 N/m^2
Stress applied, σ = 60 * 10^6 N/m^2
Temperature coefficient of resistance of gauge, αg = 20 * 10^-6 per °C
Thermal coefficient of linear expansion of gauge, βg = 16 * 10^-6 per °C
Thermal coefficient of linear expansion of steel specimen, βs = 12 * 10^-6 per °C
Change in temperature, ΔT = 40°C
(a) Strain induced in the specimen:
Strain induced, ε = σ/E = (60 * 10^6 N/m^2)/(2 * 10^11 N/m^2) = 0.0003
(b) Change in the electrical resistance:
Change in resistance of the gauge, ΔR = GF * R * ε = 2 * 120Ω * 0.0003 = 0.072Ω
(c) Change in the electrical resistance due to temperature:
Change in resistance of the gauge due to temperature, ΔRt = R * αg * ΔT = 120Ω * 20 * 10^-6 per °C * 40°C = 0.096Ω
Change in resistance of the gauge due to thermal expansion, ΔRe = R * βg * ΔT = 120Ω * 16 * 10^-6 per °C * 40°C = 0.0768Ω
Change in resistance of steel specimen due to thermal expansion, ΔRs = R * βs * ΔT * ε = 120Ω * 12 * 10^-6 per °C * 40°C * 0.0003 = 0.0144Ω
Total change in resistance, ΔRtotal = ΔRt + ΔRe + ΔRs = 0.096Ω + 0.0768Ω + 0.0144Ω = 0.1872Ω
Final answer:
Change in resistance of the gauge due to tensile stress, ΔR = 0.072Ω
Change in resistance of the gauge due to temperature, ΔRtotal = 0.1872Ω
Total change in resistance, ΔRtotal = ΔR + ΔRtotal = 0.072Ω + 0.1872Ω = 0.2592Ω
Final strain induced in the specimen, ε = ΔRtotal/(GF * R) = 0.2592Ω/(2 * 120Ω) = 0.00136
Therefore, the final answer is 0.0014 (approximated to 3 significant figures).

|
Explore Courses for GATE exam
|

|
Similar GATE Doubts
An electrical resistance strain gauge of resistance 120Ω has a gauge factor of 2. It is bonded to a steel specimen (modulus of elasticity, E = 2 * 1011 N/m2) for measuring strain. Estimate: (2002)(a) Strain induced in the specimen if tensile stress of 60 * 106 N/m2 is applied on the specimen.(b) Change in the electrical resistance of the gauge due to the tensile stress as given in (a)(c) Change in the electrical resistance of the gauge if there is an increase of temperature by 40°C.Assume the following data:Temperature coefficient of resistance of gauge is 20 * 10-6 per °CThermal coefficient of linear expansion of the gauge is 16 * 10-6 per °C.Thermal coefficient of linear expansion of steel specimen is 12 * 10-6 per °C.Correct answer is '0.3'. Can you explain this answer?
Question Description
An electrical resistance strain gauge of resistance 120Ω has a gauge factor of 2. It is bonded to a steel specimen (modulus of elasticity, E = 2 * 1011 N/m2) for measuring strain. Estimate: (2002)(a) Strain induced in the specimen if tensile stress of 60 * 106 N/m2 is applied on the specimen.(b) Change in the electrical resistance of the gauge due to the tensile stress as given in (a)(c) Change in the electrical resistance of the gauge if there is an increase of temperature by 40°C.Assume the following data:Temperature coefficient of resistance of gauge is 20 * 10-6 per °CThermal coefficient of linear expansion of the gauge is 16 * 10-6 per °C.Thermal coefficient of linear expansion of steel specimen is 12 * 10-6 per °C.Correct answer is '0.3'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about An electrical resistance strain gauge of resistance 120Ω has a gauge factor of 2. It is bonded to a steel specimen (modulus of elasticity, E = 2 * 1011 N/m2) for measuring strain. Estimate: (2002)(a) Strain induced in the specimen if tensile stress of 60 * 106 N/m2 is applied on the specimen.(b) Change in the electrical resistance of the gauge due to the tensile stress as given in (a)(c) Change in the electrical resistance of the gauge if there is an increase of temperature by 40°C.Assume the following data:Temperature coefficient of resistance of gauge is 20 * 10-6 per °CThermal coefficient of linear expansion of the gauge is 16 * 10-6 per °C.Thermal coefficient of linear expansion of steel specimen is 12 * 10-6 per °C.Correct answer is '0.3'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for An electrical resistance strain gauge of resistance 120Ω has a gauge factor of 2. It is bonded to a steel specimen (modulus of elasticity, E = 2 * 1011 N/m2) for measuring strain. Estimate: (2002)(a) Strain induced in the specimen if tensile stress of 60 * 106 N/m2 is applied on the specimen.(b) Change in the electrical resistance of the gauge due to the tensile stress as given in (a)(c) Change in the electrical resistance of the gauge if there is an increase of temperature by 40°C.Assume the following data:Temperature coefficient of resistance of gauge is 20 * 10-6 per °CThermal coefficient of linear expansion of the gauge is 16 * 10-6 per °C.Thermal coefficient of linear expansion of steel specimen is 12 * 10-6 per °C.Correct answer is '0.3'. Can you explain this answer?.
An electrical resistance strain gauge of resistance 120Ω has a gauge factor of 2. It is bonded to a steel specimen (modulus of elasticity, E = 2 * 1011 N/m2) for measuring strain. Estimate: (2002)(a) Strain induced in the specimen if tensile stress of 60 * 106 N/m2 is applied on the specimen.(b) Change in the electrical resistance of the gauge due to the tensile stress as given in (a)(c) Change in the electrical resistance of the gauge if there is an increase of temperature by 40°C.Assume the following data:Temperature coefficient of resistance of gauge is 20 * 10-6 per °CThermal coefficient of linear expansion of the gauge is 16 * 10-6 per °C.Thermal coefficient of linear expansion of steel specimen is 12 * 10-6 per °C.Correct answer is '0.3'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about An electrical resistance strain gauge of resistance 120Ω has a gauge factor of 2. It is bonded to a steel specimen (modulus of elasticity, E = 2 * 1011 N/m2) for measuring strain. Estimate: (2002)(a) Strain induced in the specimen if tensile stress of 60 * 106 N/m2 is applied on the specimen.(b) Change in the electrical resistance of the gauge due to the tensile stress as given in (a)(c) Change in the electrical resistance of the gauge if there is an increase of temperature by 40°C.Assume the following data:Temperature coefficient of resistance of gauge is 20 * 10-6 per °CThermal coefficient of linear expansion of the gauge is 16 * 10-6 per °C.Thermal coefficient of linear expansion of steel specimen is 12 * 10-6 per °C.Correct answer is '0.3'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for An electrical resistance strain gauge of resistance 120Ω has a gauge factor of 2. It is bonded to a steel specimen (modulus of elasticity, E = 2 * 1011 N/m2) for measuring strain. Estimate: (2002)(a) Strain induced in the specimen if tensile stress of 60 * 106 N/m2 is applied on the specimen.(b) Change in the electrical resistance of the gauge due to the tensile stress as given in (a)(c) Change in the electrical resistance of the gauge if there is an increase of temperature by 40°C.Assume the following data:Temperature coefficient of resistance of gauge is 20 * 10-6 per °CThermal coefficient of linear expansion of the gauge is 16 * 10-6 per °C.Thermal coefficient of linear expansion of steel specimen is 12 * 10-6 per °C.Correct answer is '0.3'. Can you explain this answer?.
Solutions for An electrical resistance strain gauge of resistance 120Ω has a gauge factor of 2. It is bonded to a steel specimen (modulus of elasticity, E = 2 * 1011 N/m2) for measuring strain. Estimate: (2002)(a) Strain induced in the specimen if tensile stress of 60 * 106 N/m2 is applied on the specimen.(b) Change in the electrical resistance of the gauge due to the tensile stress as given in (a)(c) Change in the electrical resistance of the gauge if there is an increase of temperature by 40°C.Assume the following data:Temperature coefficient of resistance of gauge is 20 * 10-6 per °CThermal coefficient of linear expansion of the gauge is 16 * 10-6 per °C.Thermal coefficient of linear expansion of steel specimen is 12 * 10-6 per °C.Correct answer is '0.3'. Can you explain this answer? in English & in Hindi are available as part of our courses for GATE.
Download more important topics, notes, lectures and mock test series for GATE Exam by signing up for free.
Here you can find the meaning of An electrical resistance strain gauge of resistance 120Ω has a gauge factor of 2. It is bonded to a steel specimen (modulus of elasticity, E = 2 * 1011 N/m2) for measuring strain. Estimate: (2002)(a) Strain induced in the specimen if tensile stress of 60 * 106 N/m2 is applied on the specimen.(b) Change in the electrical resistance of the gauge due to the tensile stress as given in (a)(c) Change in the electrical resistance of the gauge if there is an increase of temperature by 40°C.Assume the following data:Temperature coefficient of resistance of gauge is 20 * 10-6 per °CThermal coefficient of linear expansion of the gauge is 16 * 10-6 per °C.Thermal coefficient of linear expansion of steel specimen is 12 * 10-6 per °C.Correct answer is '0.3'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
An electrical resistance strain gauge of resistance 120Ω has a gauge factor of 2. It is bonded to a steel specimen (modulus of elasticity, E = 2 * 1011 N/m2) for measuring strain. Estimate: (2002)(a) Strain induced in the specimen if tensile stress of 60 * 106 N/m2 is applied on the specimen.(b) Change in the electrical resistance of the gauge due to the tensile stress as given in (a)(c) Change in the electrical resistance of the gauge if there is an increase of temperature by 40°C.Assume the following data:Temperature coefficient of resistance of gauge is 20 * 10-6 per °CThermal coefficient of linear expansion of the gauge is 16 * 10-6 per °C.Thermal coefficient of linear expansion of steel specimen is 12 * 10-6 per °C.Correct answer is '0.3'. Can you explain this answer?, a detailed solution for An electrical resistance strain gauge of resistance 120Ω has a gauge factor of 2. It is bonded to a steel specimen (modulus of elasticity, E = 2 * 1011 N/m2) for measuring strain. Estimate: (2002)(a) Strain induced in the specimen if tensile stress of 60 * 106 N/m2 is applied on the specimen.(b) Change in the electrical resistance of the gauge due to the tensile stress as given in (a)(c) Change in the electrical resistance of the gauge if there is an increase of temperature by 40°C.Assume the following data:Temperature coefficient of resistance of gauge is 20 * 10-6 per °CThermal coefficient of linear expansion of the gauge is 16 * 10-6 per °C.Thermal coefficient of linear expansion of steel specimen is 12 * 10-6 per °C.Correct answer is '0.3'. Can you explain this answer? has been provided alongside types of An electrical resistance strain gauge of resistance 120Ω has a gauge factor of 2. It is bonded to a steel specimen (modulus of elasticity, E = 2 * 1011 N/m2) for measuring strain. Estimate: (2002)(a) Strain induced in the specimen if tensile stress of 60 * 106 N/m2 is applied on the specimen.(b) Change in the electrical resistance of the gauge due to the tensile stress as given in (a)(c) Change in the electrical resistance of the gauge if there is an increase of temperature by 40°C.Assume the following data:Temperature coefficient of resistance of gauge is 20 * 10-6 per °CThermal coefficient of linear expansion of the gauge is 16 * 10-6 per °C.Thermal coefficient of linear expansion of steel specimen is 12 * 10-6 per °C.Correct answer is '0.3'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice An electrical resistance strain gauge of resistance 120Ω has a gauge factor of 2. It is bonded to a steel specimen (modulus of elasticity, E = 2 * 1011 N/m2) for measuring strain. Estimate: (2002)(a) Strain induced in the specimen if tensile stress of 60 * 106 N/m2 is applied on the specimen.(b) Change in the electrical resistance of the gauge due to the tensile stress as given in (a)(c) Change in the electrical resistance of the gauge if there is an increase of temperature by 40°C.Assume the following data:Temperature coefficient of resistance of gauge is 20 * 10-6 per °CThermal coefficient of linear expansion of the gauge is 16 * 10-6 per °C.Thermal coefficient of linear expansion of steel specimen is 12 * 10-6 per °C.Correct answer is '0.3'. Can you explain this answer? tests, examples and also practice GATE tests.

|
Explore Courses for GATE exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















