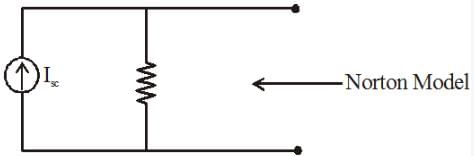
Norton's theorem is a useful technique in circuit analysis that allows us to simplify complex circuits by replacing them with an equivalent circuit consisting of a current source and an impedance in parallel. This theorem is particularly helpful when we want to find the current flowing through a specific branch of a circuit.

Norton's theorem states that any linear bilateral network can be replaced by an equivalent circuit consisting of a current source and an impedance in parallel. The current source is called the Norton current, denoted as IN, and the impedance is called the Norton impedance, denoted as ZN.

Norton's theorem can be applied to a circuit by following these steps:

1. Identify the load resistor or branch for which we want to find the current.

2. Disconnect the load resistor or branch from the circuit.

3. Calculate the open-circuit voltage across the load terminals.

4. Calculate the short-circuit current through the load terminals.

5. The Norton current (IN) is equal to the short-circuit current, and the Norton impedance (ZN) is equal to the open-circuit voltage divided by the short-circuit current.

The equivalent circuit obtained using Norton's theorem consists of a current source with a value equal to the Norton current (IN) in parallel with an impedance equal to the Norton impedance (ZN). This equivalent circuit accurately represents the behavior of the original circuit with respect to the specific load resistor or branch.

By using Norton's theorem, we can simplify complex circuits and analyze the behavior of the circuit with respect to a specific load. This enables us to calculate the current flowing through the load resistor or branch without having to analyze the entire circuit. It also allows us to easily determine the effect of changes in the load resistor or branch on the circuit's behavior.

In summary, Norton's theorem is a powerful tool in circuit analysis that allows us to simplify complex circuits by replacing them with an equivalent circuit consisting of a current source and an impedance in parallel. This simplification helps in analyzing and calculating the behavior of circuits with respect to specific loads.