SSC Exam > SSC Questions > Bending moment M and torque T are applied on...
Start Learning for Free
Bending moment M and torque T are applied on a solid circular shaft. If the maximum bending stress equals maximum shear stress developed, then M is equal to
- a)T/2
- b)T
- c)2T
- d)4T
Correct answer is option 'A'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
Bending moment M and torque T are applied on a solid circular shaft. ...
Bending stress due to bending moment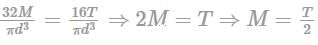
View all questions of this test
σ = 32M/πd3
Shear stress due to twisting moment/ torque:
τ = 16T/πd3
If the maximum bending stress equals maximum shear stress developed:
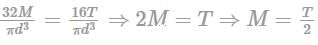
Most Upvoted Answer
Bending moment M and torque T are applied on a solid circular shaft. ...
Explanation:
The maximum bending stress and maximum shear stress occur at different points in a circular shaft.
When a bending moment is applied to a circular shaft, it causes the shaft to bend. This bending creates tensile and compressive stresses on the cross-section of the shaft. The maximum bending stress occurs at the outermost fibers of the shaft, farthest from the neutral axis.
When a torque is applied to a circular shaft, it causes the shaft to twist. This twisting creates shear stresses on the cross-section of the shaft. The maximum shear stress occurs at the outermost fibers of the shaft, perpendicular to the axis of the shaft.
Bending Moment and Shear Stress:
- Bending moment (M) is the product of the applied force and the distance from the axis of rotation.
- Torque (T) is the product of the applied force and the radius of the shaft.
In a circular shaft, the maximum bending stress (σ_b) is given by the formula:
σ_b = (M * c) / I
Where:
- M is the bending moment applied to the shaft
- c is the distance from the neutral axis to the outermost fiber of the shaft
- I is the moment of inertia of the shaft cross-section
The maximum shear stress (τ) is given by the formula:
τ = (T * r) / J
Where:
- T is the torque applied to the shaft
- r is the radius of the shaft
- J is the polar moment of inertia of the shaft cross-section
Equalizing the Maximum Bending Stress and Maximum Shear Stress:
To determine when the maximum bending stress equals the maximum shear stress, we can equate the formulas for bending stress and shear stress.
σ_b = τ
Substituting the formulas for bending stress and shear stress, we get:
(M * c) / I = (T * r) / J
Simplifying the equation, we find:
(M * c * J) = (T * r * I)
Since c, J, r, and I are constants for a given shaft, we can further simplify the equation:
M = T * (r * I) / (c * J)
From the equation, we can see that the bending moment (M) is proportional to the torque (T). Therefore, if the maximum bending stress equals the maximum shear stress, then M is equal to T divided by a constant factor.
Conclusion:
In this case, the correct answer is option 'A', M is equal to T/2.
The maximum bending stress and maximum shear stress occur at different points in a circular shaft.
When a bending moment is applied to a circular shaft, it causes the shaft to bend. This bending creates tensile and compressive stresses on the cross-section of the shaft. The maximum bending stress occurs at the outermost fibers of the shaft, farthest from the neutral axis.
When a torque is applied to a circular shaft, it causes the shaft to twist. This twisting creates shear stresses on the cross-section of the shaft. The maximum shear stress occurs at the outermost fibers of the shaft, perpendicular to the axis of the shaft.
Bending Moment and Shear Stress:
- Bending moment (M) is the product of the applied force and the distance from the axis of rotation.
- Torque (T) is the product of the applied force and the radius of the shaft.
In a circular shaft, the maximum bending stress (σ_b) is given by the formula:
σ_b = (M * c) / I
Where:
- M is the bending moment applied to the shaft
- c is the distance from the neutral axis to the outermost fiber of the shaft
- I is the moment of inertia of the shaft cross-section
The maximum shear stress (τ) is given by the formula:
τ = (T * r) / J
Where:
- T is the torque applied to the shaft
- r is the radius of the shaft
- J is the polar moment of inertia of the shaft cross-section
Equalizing the Maximum Bending Stress and Maximum Shear Stress:
To determine when the maximum bending stress equals the maximum shear stress, we can equate the formulas for bending stress and shear stress.
σ_b = τ
Substituting the formulas for bending stress and shear stress, we get:
(M * c) / I = (T * r) / J
Simplifying the equation, we find:
(M * c * J) = (T * r * I)
Since c, J, r, and I are constants for a given shaft, we can further simplify the equation:
M = T * (r * I) / (c * J)
From the equation, we can see that the bending moment (M) is proportional to the torque (T). Therefore, if the maximum bending stress equals the maximum shear stress, then M is equal to T divided by a constant factor.
Conclusion:
In this case, the correct answer is option 'A', M is equal to T/2.

|
Explore Courses for SSC exam
|

|
Similar SSC Doubts
Bending moment M and torque T are applied on a solid circular shaft. If the maximum bending stress equals maximum shear stress developed, then M is equal toa)T/2b)Tc)2Td)4TCorrect answer is option 'A'. Can you explain this answer?
Question Description
Bending moment M and torque T are applied on a solid circular shaft. If the maximum bending stress equals maximum shear stress developed, then M is equal toa)T/2b)Tc)2Td)4TCorrect answer is option 'A'. Can you explain this answer? for SSC 2024 is part of SSC preparation. The Question and answers have been prepared according to the SSC exam syllabus. Information about Bending moment M and torque T are applied on a solid circular shaft. If the maximum bending stress equals maximum shear stress developed, then M is equal toa)T/2b)Tc)2Td)4TCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for SSC 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Bending moment M and torque T are applied on a solid circular shaft. If the maximum bending stress equals maximum shear stress developed, then M is equal toa)T/2b)Tc)2Td)4TCorrect answer is option 'A'. Can you explain this answer?.
Bending moment M and torque T are applied on a solid circular shaft. If the maximum bending stress equals maximum shear stress developed, then M is equal toa)T/2b)Tc)2Td)4TCorrect answer is option 'A'. Can you explain this answer? for SSC 2024 is part of SSC preparation. The Question and answers have been prepared according to the SSC exam syllabus. Information about Bending moment M and torque T are applied on a solid circular shaft. If the maximum bending stress equals maximum shear stress developed, then M is equal toa)T/2b)Tc)2Td)4TCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for SSC 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Bending moment M and torque T are applied on a solid circular shaft. If the maximum bending stress equals maximum shear stress developed, then M is equal toa)T/2b)Tc)2Td)4TCorrect answer is option 'A'. Can you explain this answer?.
Solutions for Bending moment M and torque T are applied on a solid circular shaft. If the maximum bending stress equals maximum shear stress developed, then M is equal toa)T/2b)Tc)2Td)4TCorrect answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for SSC.
Download more important topics, notes, lectures and mock test series for SSC Exam by signing up for free.
Here you can find the meaning of Bending moment M and torque T are applied on a solid circular shaft. If the maximum bending stress equals maximum shear stress developed, then M is equal toa)T/2b)Tc)2Td)4TCorrect answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Bending moment M and torque T are applied on a solid circular shaft. If the maximum bending stress equals maximum shear stress developed, then M is equal toa)T/2b)Tc)2Td)4TCorrect answer is option 'A'. Can you explain this answer?, a detailed solution for Bending moment M and torque T are applied on a solid circular shaft. If the maximum bending stress equals maximum shear stress developed, then M is equal toa)T/2b)Tc)2Td)4TCorrect answer is option 'A'. Can you explain this answer? has been provided alongside types of Bending moment M and torque T are applied on a solid circular shaft. If the maximum bending stress equals maximum shear stress developed, then M is equal toa)T/2b)Tc)2Td)4TCorrect answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Bending moment M and torque T are applied on a solid circular shaft. If the maximum bending stress equals maximum shear stress developed, then M is equal toa)T/2b)Tc)2Td)4TCorrect answer is option 'A'. Can you explain this answer? tests, examples and also practice SSC tests.

|
Explore Courses for SSC exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















