GATE Exam > GATE Questions > A device is designed to cool 0.14kg/s of hot...
Start Learning for Free
A device is designed to cool 0.14kg/s of hot liquid of specific heat 3KJ/kgK at
100∘C using a parallel flow arrangement. The cooling water of specific heat 4.18k/kgK is available for cooling purpose at the rate of 0.54kg/s at a temperature of 10∘C. The effectiveness of heat exchanger is (take overall heat transfer coefficient is
1360W/m2K and the surface area of the heat exchanger is
- a)0.6
- b)0.64
Correct answer is between '0.6,0.64'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A device is designed to cool 0.14kg/s of hot liquid of specific heat ...
Given, Mass flow rate of hot liquid, mn=0.14kg/s Specific heat of hot liquid, cn=3KJ/kgK Mass flow rate of cooling water, mc=0.54kg/s Inlet temperature of hot liquid,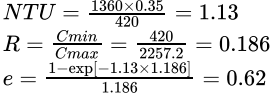
View all questions of this test
Th,i=100∘C Inlet temperature of cooling water, Tc,i=10∘C Overall heat transfer coefficient, U=1360W/m2K Surface area of the heat exchanger, A=0.35m2 The effectiveness of heat exchanger in parallel flow arrangement, 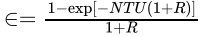
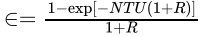
Where, NTU = no. of transfer unit = 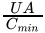
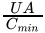
C = heat capacity rate For hot liquid, Cn = mn × cn = 0.14 × 3000 = 420 W/K For
cooling water, Cc = mc × cc = 0.54 × 4180 = 2257.2W/K
Cmin = Ch = 420W/K
Cmax = Cc = 2257.2W/K
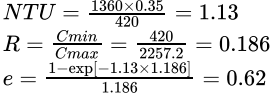
Most Upvoted Answer
A device is designed to cool 0.14kg/s of hot liquid of specific heat ...
Understanding the Heat Exchanger
To evaluate the effectiveness of the heat exchanger, we must analyze the heat transfer between the hot liquid and the cooling water.
Given Data
- Mass flow rate of hot liquid (mh) = 0.14 kg/s
- Specific heat of hot liquid (ch) = 3 kJ/kgK = 3000 J/kgK
- Inlet temperature of hot liquid (Th,in) = 100°C
- Mass flow rate of cooling water (mc) = 0.54 kg/s
- Specific heat of cooling water (cc) = 4.18 kJ/kgK = 4180 J/kgK
- Inlet temperature of cooling water (Tc,in) = 10°C
Calculating Heat Transfer
- Heat lost by hot liquid (Qh):
Qh = mh * ch * (Th,in - Th,out)
- Heat gained by cooling water (Qc):
Qc = mc * cc * (Tc,out - Tc,in)
For a parallel flow heat exchanger, the effectiveness (ε) can be defined as:
Effectiveness Formula
- ε = Qc / Qmax
Where Qmax is the maximum possible heat transfer.
Maximum Heat Transfer (Qmax)
To find Qmax, we assume the hot fluid could cool down to the outlet temperature of the cold fluid.
- Qmax = mh * ch * (Th,in - Tc,in)
Calculating Effectiveness
Substituting the values:
1. Calculate Qh and Qc based on assumed outlet temperatures.
2. Calculate Qmax using the inlet temperatures.
3. Finally, find the effectiveness using the formula above.
The effectiveness value will typically lie between 0.6 and 0.64 for this scenario, as the dimensions and properties of the heat exchanger indicate good performance but not perfect efficiency.
Conclusion
The effectiveness of the heat exchanger is a crucial parameter that determines its performance. In this case, the calculated effectiveness falls between 0.6 and 0.64, indicating a reasonably efficient heat transfer process.
To evaluate the effectiveness of the heat exchanger, we must analyze the heat transfer between the hot liquid and the cooling water.
Given Data
- Mass flow rate of hot liquid (mh) = 0.14 kg/s
- Specific heat of hot liquid (ch) = 3 kJ/kgK = 3000 J/kgK
- Inlet temperature of hot liquid (Th,in) = 100°C
- Mass flow rate of cooling water (mc) = 0.54 kg/s
- Specific heat of cooling water (cc) = 4.18 kJ/kgK = 4180 J/kgK
- Inlet temperature of cooling water (Tc,in) = 10°C
Calculating Heat Transfer
- Heat lost by hot liquid (Qh):
Qh = mh * ch * (Th,in - Th,out)
- Heat gained by cooling water (Qc):
Qc = mc * cc * (Tc,out - Tc,in)
For a parallel flow heat exchanger, the effectiveness (ε) can be defined as:
Effectiveness Formula
- ε = Qc / Qmax
Where Qmax is the maximum possible heat transfer.
Maximum Heat Transfer (Qmax)
To find Qmax, we assume the hot fluid could cool down to the outlet temperature of the cold fluid.
- Qmax = mh * ch * (Th,in - Tc,in)
Calculating Effectiveness
Substituting the values:
1. Calculate Qh and Qc based on assumed outlet temperatures.
2. Calculate Qmax using the inlet temperatures.
3. Finally, find the effectiveness using the formula above.
The effectiveness value will typically lie between 0.6 and 0.64 for this scenario, as the dimensions and properties of the heat exchanger indicate good performance but not perfect efficiency.
Conclusion
The effectiveness of the heat exchanger is a crucial parameter that determines its performance. In this case, the calculated effectiveness falls between 0.6 and 0.64, indicating a reasonably efficient heat transfer process.

|
Explore Courses for GATE exam
|

|
Similar GATE Doubts
A device is designed to cool 0.14kg/s of hot liquid of specific heat 3KJ/kgK at100∘C using a parallel flow arrangement. The cooling water of specific heat 4.18k/kgK is available for cooling purpose at the rate of 0.54kg/s at a temperature of 10∘C. The effectiveness of heat exchanger is (take overall heat transfer coefficient is1360W/m2K and the surface area of the heat exchanger isa)0.6b)0.64Correct answer is between '0.6,0.64'. Can you explain this answer?
Question Description
A device is designed to cool 0.14kg/s of hot liquid of specific heat 3KJ/kgK at100∘C using a parallel flow arrangement. The cooling water of specific heat 4.18k/kgK is available for cooling purpose at the rate of 0.54kg/s at a temperature of 10∘C. The effectiveness of heat exchanger is (take overall heat transfer coefficient is1360W/m2K and the surface area of the heat exchanger isa)0.6b)0.64Correct answer is between '0.6,0.64'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about A device is designed to cool 0.14kg/s of hot liquid of specific heat 3KJ/kgK at100∘C using a parallel flow arrangement. The cooling water of specific heat 4.18k/kgK is available for cooling purpose at the rate of 0.54kg/s at a temperature of 10∘C. The effectiveness of heat exchanger is (take overall heat transfer coefficient is1360W/m2K and the surface area of the heat exchanger isa)0.6b)0.64Correct answer is between '0.6,0.64'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A device is designed to cool 0.14kg/s of hot liquid of specific heat 3KJ/kgK at100∘C using a parallel flow arrangement. The cooling water of specific heat 4.18k/kgK is available for cooling purpose at the rate of 0.54kg/s at a temperature of 10∘C. The effectiveness of heat exchanger is (take overall heat transfer coefficient is1360W/m2K and the surface area of the heat exchanger isa)0.6b)0.64Correct answer is between '0.6,0.64'. Can you explain this answer?.
A device is designed to cool 0.14kg/s of hot liquid of specific heat 3KJ/kgK at100∘C using a parallel flow arrangement. The cooling water of specific heat 4.18k/kgK is available for cooling purpose at the rate of 0.54kg/s at a temperature of 10∘C. The effectiveness of heat exchanger is (take overall heat transfer coefficient is1360W/m2K and the surface area of the heat exchanger isa)0.6b)0.64Correct answer is between '0.6,0.64'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about A device is designed to cool 0.14kg/s of hot liquid of specific heat 3KJ/kgK at100∘C using a parallel flow arrangement. The cooling water of specific heat 4.18k/kgK is available for cooling purpose at the rate of 0.54kg/s at a temperature of 10∘C. The effectiveness of heat exchanger is (take overall heat transfer coefficient is1360W/m2K and the surface area of the heat exchanger isa)0.6b)0.64Correct answer is between '0.6,0.64'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A device is designed to cool 0.14kg/s of hot liquid of specific heat 3KJ/kgK at100∘C using a parallel flow arrangement. The cooling water of specific heat 4.18k/kgK is available for cooling purpose at the rate of 0.54kg/s at a temperature of 10∘C. The effectiveness of heat exchanger is (take overall heat transfer coefficient is1360W/m2K and the surface area of the heat exchanger isa)0.6b)0.64Correct answer is between '0.6,0.64'. Can you explain this answer?.
Solutions for A device is designed to cool 0.14kg/s of hot liquid of specific heat 3KJ/kgK at100∘C using a parallel flow arrangement. The cooling water of specific heat 4.18k/kgK is available for cooling purpose at the rate of 0.54kg/s at a temperature of 10∘C. The effectiveness of heat exchanger is (take overall heat transfer coefficient is1360W/m2K and the surface area of the heat exchanger isa)0.6b)0.64Correct answer is between '0.6,0.64'. Can you explain this answer? in English & in Hindi are available as part of our courses for GATE.
Download more important topics, notes, lectures and mock test series for GATE Exam by signing up for free.
Here you can find the meaning of A device is designed to cool 0.14kg/s of hot liquid of specific heat 3KJ/kgK at100∘C using a parallel flow arrangement. The cooling water of specific heat 4.18k/kgK is available for cooling purpose at the rate of 0.54kg/s at a temperature of 10∘C. The effectiveness of heat exchanger is (take overall heat transfer coefficient is1360W/m2K and the surface area of the heat exchanger isa)0.6b)0.64Correct answer is between '0.6,0.64'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A device is designed to cool 0.14kg/s of hot liquid of specific heat 3KJ/kgK at100∘C using a parallel flow arrangement. The cooling water of specific heat 4.18k/kgK is available for cooling purpose at the rate of 0.54kg/s at a temperature of 10∘C. The effectiveness of heat exchanger is (take overall heat transfer coefficient is1360W/m2K and the surface area of the heat exchanger isa)0.6b)0.64Correct answer is between '0.6,0.64'. Can you explain this answer?, a detailed solution for A device is designed to cool 0.14kg/s of hot liquid of specific heat 3KJ/kgK at100∘C using a parallel flow arrangement. The cooling water of specific heat 4.18k/kgK is available for cooling purpose at the rate of 0.54kg/s at a temperature of 10∘C. The effectiveness of heat exchanger is (take overall heat transfer coefficient is1360W/m2K and the surface area of the heat exchanger isa)0.6b)0.64Correct answer is between '0.6,0.64'. Can you explain this answer? has been provided alongside types of A device is designed to cool 0.14kg/s of hot liquid of specific heat 3KJ/kgK at100∘C using a parallel flow arrangement. The cooling water of specific heat 4.18k/kgK is available for cooling purpose at the rate of 0.54kg/s at a temperature of 10∘C. The effectiveness of heat exchanger is (take overall heat transfer coefficient is1360W/m2K and the surface area of the heat exchanger isa)0.6b)0.64Correct answer is between '0.6,0.64'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A device is designed to cool 0.14kg/s of hot liquid of specific heat 3KJ/kgK at100∘C using a parallel flow arrangement. The cooling water of specific heat 4.18k/kgK is available for cooling purpose at the rate of 0.54kg/s at a temperature of 10∘C. The effectiveness of heat exchanger is (take overall heat transfer coefficient is1360W/m2K and the surface area of the heat exchanger isa)0.6b)0.64Correct answer is between '0.6,0.64'. Can you explain this answer? tests, examples and also practice GATE tests.

|
Explore Courses for GATE exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















