NEET Exam > NEET Questions > A particle originally at rest at the highest ...
Start Learning for Free
A particle originally at rest at the highest point of a smooth vertical circle is slightly displaced . It will leave the circle at a vertical distance h below the highest point such that (a)h=R (b) h=R/3 ( c ) h= R/2 .if any body have universal self scorer so plz seen question no.14 in motion in 2 dimensions .?
Verified Answer
A particle originally at rest at the highest point of a smooth vertica...
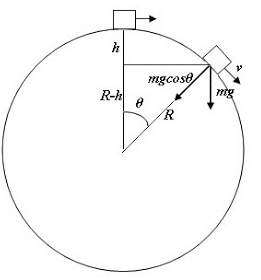
Suppose the particle leaves the circle at distance h below the highest point.
The point where it leaves the circle reaction force(N)=0
therefore mgcosθ = mv2/R
or cosθ =v2/Rg
Now applying conservation of energy
mgh = (1/2)mv2
or v2 = 2gh
therefore cosθ = 2h/R
by geometry
cosθ = R-h/R,
therefore R-h/R = 2h/R or h = R/3
 This question is part of UPSC exam. View all NEET courses
This question is part of UPSC exam. View all NEET courses
Most Upvoted Answer
A particle originally at rest at the highest point of a smooth vertica...
The problem asks us to determine the vertical distance h below the highest point at which a particle will leave a smooth vertical circle, given that it is slightly displaced from rest. We are given three options for h: (a) h = R, (b) h = R/3, and (c) h = R/2.
To solve this problem, we need to consider the forces acting on the particle at different points of the circle and analyze the energy changes.
1. Forces Acting on the Particle:
- At the highest point: The only force acting on the particle is the weight, which is directed downwards.
- At any other point: In addition to the weight, there is also a normal force acting on the particle, which is directed towards the center of the circle. This force provides the necessary centripetal force for circular motion.
2. Energy Analysis:
- At the highest point: The particle has maximum potential energy and zero kinetic energy.
- At any other point: The particle has both potential energy and kinetic energy. The total mechanical energy (E) of the particle is given by the sum of its potential energy (PE) and kinetic energy (KE): E = PE + KE.
3. Displacement from Rest:
- When the particle is slightly displaced from rest, it will have a non-zero initial velocity. This means that it will have kinetic energy at the highest point.
4. Conservation of Energy:
- According to the principle of conservation of energy, the total mechanical energy of the particle remains constant throughout its motion.
- Therefore, the sum of the particle's potential energy and kinetic energy at any point should be equal to the sum of its potential energy and kinetic energy at the highest point.
5. Analyzing the Options:
- (a) h = R: If the particle leaves the circle at a vertical distance equal to the radius (R), it means that it has lost all its potential energy and gained maximum kinetic energy. This would require a large initial displacement from rest, which is not mentioned in the problem.
- (b) h = R/3: This option suggests that the particle loses a significant amount of potential energy and gains some kinetic energy. This seems plausible as a slight displacement from rest would result in a smaller loss of potential energy.
- (c) h = R/2: This option suggests that the particle loses half of its potential energy and gains half of its kinetic energy. This seems plausible as a slight displacement from rest would result in a moderate loss of potential energy.
Based on the analysis, option (b) h = R/3 and option (c) h = R/2 seem more reasonable compared to option (a) h = R. However, without additional information about the initial displacement, it is difficult to determine the exact value of h.
To solve this problem, we need to consider the forces acting on the particle at different points of the circle and analyze the energy changes.
1. Forces Acting on the Particle:
- At the highest point: The only force acting on the particle is the weight, which is directed downwards.
- At any other point: In addition to the weight, there is also a normal force acting on the particle, which is directed towards the center of the circle. This force provides the necessary centripetal force for circular motion.
2. Energy Analysis:
- At the highest point: The particle has maximum potential energy and zero kinetic energy.
- At any other point: The particle has both potential energy and kinetic energy. The total mechanical energy (E) of the particle is given by the sum of its potential energy (PE) and kinetic energy (KE): E = PE + KE.
3. Displacement from Rest:
- When the particle is slightly displaced from rest, it will have a non-zero initial velocity. This means that it will have kinetic energy at the highest point.
4. Conservation of Energy:
- According to the principle of conservation of energy, the total mechanical energy of the particle remains constant throughout its motion.
- Therefore, the sum of the particle's potential energy and kinetic energy at any point should be equal to the sum of its potential energy and kinetic energy at the highest point.
5. Analyzing the Options:
- (a) h = R: If the particle leaves the circle at a vertical distance equal to the radius (R), it means that it has lost all its potential energy and gained maximum kinetic energy. This would require a large initial displacement from rest, which is not mentioned in the problem.
- (b) h = R/3: This option suggests that the particle loses a significant amount of potential energy and gains some kinetic energy. This seems plausible as a slight displacement from rest would result in a smaller loss of potential energy.
- (c) h = R/2: This option suggests that the particle loses half of its potential energy and gains half of its kinetic energy. This seems plausible as a slight displacement from rest would result in a moderate loss of potential energy.
Based on the analysis, option (b) h = R/3 and option (c) h = R/2 seem more reasonable compared to option (a) h = R. However, without additional information about the initial displacement, it is difficult to determine the exact value of h.
Attention NEET Students!
To make sure you are not studying endlessly, EduRev has designed NEET study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in NEET.

|
Explore Courses for NEET exam
|

|
Similar NEET Doubts
A particle originally at rest at the highest point of a smooth vertical circle is slightly displaced . It will leave the circle at a vertical distance h below the highest point such that (a)h=R (b) h=R/3 ( c ) h= R/2 .if any body have universal self scorer so plz seen question no.14 in motion in 2 dimensions .?
Question Description
A particle originally at rest at the highest point of a smooth vertical circle is slightly displaced . It will leave the circle at a vertical distance h below the highest point such that (a)h=R (b) h=R/3 ( c ) h= R/2 .if any body have universal self scorer so plz seen question no.14 in motion in 2 dimensions .? for NEET 2024 is part of NEET preparation. The Question and answers have been prepared according to the NEET exam syllabus. Information about A particle originally at rest at the highest point of a smooth vertical circle is slightly displaced . It will leave the circle at a vertical distance h below the highest point such that (a)h=R (b) h=R/3 ( c ) h= R/2 .if any body have universal self scorer so plz seen question no.14 in motion in 2 dimensions .? covers all topics & solutions for NEET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A particle originally at rest at the highest point of a smooth vertical circle is slightly displaced . It will leave the circle at a vertical distance h below the highest point such that (a)h=R (b) h=R/3 ( c ) h= R/2 .if any body have universal self scorer so plz seen question no.14 in motion in 2 dimensions .?.
A particle originally at rest at the highest point of a smooth vertical circle is slightly displaced . It will leave the circle at a vertical distance h below the highest point such that (a)h=R (b) h=R/3 ( c ) h= R/2 .if any body have universal self scorer so plz seen question no.14 in motion in 2 dimensions .? for NEET 2024 is part of NEET preparation. The Question and answers have been prepared according to the NEET exam syllabus. Information about A particle originally at rest at the highest point of a smooth vertical circle is slightly displaced . It will leave the circle at a vertical distance h below the highest point such that (a)h=R (b) h=R/3 ( c ) h= R/2 .if any body have universal self scorer so plz seen question no.14 in motion in 2 dimensions .? covers all topics & solutions for NEET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A particle originally at rest at the highest point of a smooth vertical circle is slightly displaced . It will leave the circle at a vertical distance h below the highest point such that (a)h=R (b) h=R/3 ( c ) h= R/2 .if any body have universal self scorer so plz seen question no.14 in motion in 2 dimensions .?.
Solutions for A particle originally at rest at the highest point of a smooth vertical circle is slightly displaced . It will leave the circle at a vertical distance h below the highest point such that (a)h=R (b) h=R/3 ( c ) h= R/2 .if any body have universal self scorer so plz seen question no.14 in motion in 2 dimensions .? in English & in Hindi are available as part of our courses for NEET.
Download more important topics, notes, lectures and mock test series for NEET Exam by signing up for free.
Here you can find the meaning of A particle originally at rest at the highest point of a smooth vertical circle is slightly displaced . It will leave the circle at a vertical distance h below the highest point such that (a)h=R (b) h=R/3 ( c ) h= R/2 .if any body have universal self scorer so plz seen question no.14 in motion in 2 dimensions .? defined & explained in the simplest way possible. Besides giving the explanation of
A particle originally at rest at the highest point of a smooth vertical circle is slightly displaced . It will leave the circle at a vertical distance h below the highest point such that (a)h=R (b) h=R/3 ( c ) h= R/2 .if any body have universal self scorer so plz seen question no.14 in motion in 2 dimensions .?, a detailed solution for A particle originally at rest at the highest point of a smooth vertical circle is slightly displaced . It will leave the circle at a vertical distance h below the highest point such that (a)h=R (b) h=R/3 ( c ) h= R/2 .if any body have universal self scorer so plz seen question no.14 in motion in 2 dimensions .? has been provided alongside types of A particle originally at rest at the highest point of a smooth vertical circle is slightly displaced . It will leave the circle at a vertical distance h below the highest point such that (a)h=R (b) h=R/3 ( c ) h= R/2 .if any body have universal self scorer so plz seen question no.14 in motion in 2 dimensions .? theory, EduRev gives you an
ample number of questions to practice A particle originally at rest at the highest point of a smooth vertical circle is slightly displaced . It will leave the circle at a vertical distance h below the highest point such that (a)h=R (b) h=R/3 ( c ) h= R/2 .if any body have universal self scorer so plz seen question no.14 in motion in 2 dimensions .? tests, examples and also practice NEET tests.

|
Explore Courses for NEET exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.

























