NEET Exam > NEET Questions > A vibration magnetometer placed in a magnetic...
Start Learning for Free
A vibration magnetometer placed in a magnetic meridian has a small bar magnet. the magnet execute oscillation with the time period of 2 second in Earth's horizontal magnetic field of 24 microtesla. when a horizontal field of 18 microtesla is produced opposite to the earth's field by placing a current carrying wire , the new time period of the magnetic field will be?
Verified Answer
A vibration magnetometer placed in a magnetic meridian has a small bar...
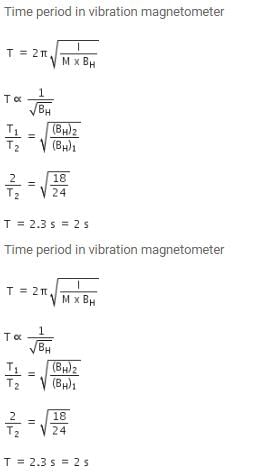
 This question is part of UPSC exam. View all NEET courses
This question is part of UPSC exam. View all NEET courses
Most Upvoted Answer
A vibration magnetometer placed in a magnetic meridian has a small bar...
Introduction:
A vibration magnetometer is a device used to measure the strength and direction of a magnetic field. It consists of a small bar magnet suspended by a thin fiber or spring, allowing it to oscillate freely. The time period of these oscillations can be used to determine the strength of the magnetic field.
Given Information:
- Time period of the magnet's oscillations in Earth's magnetic field: 2 seconds
- Strength of Earth's horizontal magnetic field: 24 microtesla
- Strength of the horizontal magnetic field produced by a current carrying wire in the opposite direction: 18 microtesla
Analysis:
When the magnet is placed in Earth's magnetic field, it aligns itself with the field lines and executes oscillations. The time period of these oscillations is directly proportional to the strength of the magnetic field.
When a current carrying wire is placed near the magnetometer, it produces a magnetic field opposite to the Earth's field. This new magnetic field affects the magnet's oscillations and changes its time period.
Solution:
To determine the new time period of the magnet's oscillations, we can use the concept of magnetic forces and the equation of motion.
1. Find the magnetic force:
The magnetic force on the magnet due to the Earth's field is given by the equation:
F1 = B1 * I * l * sinθ
where B1 is the strength of the Earth's magnetic field, I is the current in the wire, l is the length of the wire, and θ is the angle between the wire and the magnet.
2. Find the new magnetic force:
When the wire produces a magnetic field opposite to the Earth's field, the total magnetic force on the magnet becomes:
F_total = F1 - F2
where F2 is the magnetic force due to the new field produced by the wire. Since the magnetometer is in equilibrium during its oscillations, F_total is equal to zero.
3. Calculate the new time period:
Using the equation of motion for simple harmonic motion, we have:
F_total = -mω^2A
where m is the mass of the magnet, ω is the angular frequency, and A is the amplitude of the oscillations.
Since F_total is equal to zero, we can solve for the new angular frequency:
ω_new = sqrt((F1-F2)/(mA))
Finally, we can calculate the new time period using the equation:
T_new = 2π/ω_new
Conclusion:
By considering the magnetic forces and the equation of motion, we can determine the new time period of the magnet's oscillations when a horizontal magnetic field opposite to the Earth's field is produced by a current carrying wire.
A vibration magnetometer is a device used to measure the strength and direction of a magnetic field. It consists of a small bar magnet suspended by a thin fiber or spring, allowing it to oscillate freely. The time period of these oscillations can be used to determine the strength of the magnetic field.
Given Information:
- Time period of the magnet's oscillations in Earth's magnetic field: 2 seconds
- Strength of Earth's horizontal magnetic field: 24 microtesla
- Strength of the horizontal magnetic field produced by a current carrying wire in the opposite direction: 18 microtesla
Analysis:
When the magnet is placed in Earth's magnetic field, it aligns itself with the field lines and executes oscillations. The time period of these oscillations is directly proportional to the strength of the magnetic field.
When a current carrying wire is placed near the magnetometer, it produces a magnetic field opposite to the Earth's field. This new magnetic field affects the magnet's oscillations and changes its time period.
Solution:
To determine the new time period of the magnet's oscillations, we can use the concept of magnetic forces and the equation of motion.
1. Find the magnetic force:
The magnetic force on the magnet due to the Earth's field is given by the equation:
F1 = B1 * I * l * sinθ
where B1 is the strength of the Earth's magnetic field, I is the current in the wire, l is the length of the wire, and θ is the angle between the wire and the magnet.
2. Find the new magnetic force:
When the wire produces a magnetic field opposite to the Earth's field, the total magnetic force on the magnet becomes:
F_total = F1 - F2
where F2 is the magnetic force due to the new field produced by the wire. Since the magnetometer is in equilibrium during its oscillations, F_total is equal to zero.
3. Calculate the new time period:
Using the equation of motion for simple harmonic motion, we have:
F_total = -mω^2A
where m is the mass of the magnet, ω is the angular frequency, and A is the amplitude of the oscillations.
Since F_total is equal to zero, we can solve for the new angular frequency:
ω_new = sqrt((F1-F2)/(mA))
Finally, we can calculate the new time period using the equation:
T_new = 2π/ω_new
Conclusion:
By considering the magnetic forces and the equation of motion, we can determine the new time period of the magnet's oscillations when a horizontal magnetic field opposite to the Earth's field is produced by a current carrying wire.
Attention NEET Students!
To make sure you are not studying endlessly, EduRev has designed NEET study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in NEET.

|
Explore Courses for NEET exam
|

|
Similar NEET Doubts
A vibration magnetometer placed in a magnetic meridian has a small bar magnet. the magnet execute oscillation with the time period of 2 second in Earth's horizontal magnetic field of 24 microtesla. when a horizontal field of 18 microtesla is produced opposite to the earth's field by placing a current carrying wire , the new time period of the magnetic field will be?
Question Description
A vibration magnetometer placed in a magnetic meridian has a small bar magnet. the magnet execute oscillation with the time period of 2 second in Earth's horizontal magnetic field of 24 microtesla. when a horizontal field of 18 microtesla is produced opposite to the earth's field by placing a current carrying wire , the new time period of the magnetic field will be? for NEET 2024 is part of NEET preparation. The Question and answers have been prepared according to the NEET exam syllabus. Information about A vibration magnetometer placed in a magnetic meridian has a small bar magnet. the magnet execute oscillation with the time period of 2 second in Earth's horizontal magnetic field of 24 microtesla. when a horizontal field of 18 microtesla is produced opposite to the earth's field by placing a current carrying wire , the new time period of the magnetic field will be? covers all topics & solutions for NEET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A vibration magnetometer placed in a magnetic meridian has a small bar magnet. the magnet execute oscillation with the time period of 2 second in Earth's horizontal magnetic field of 24 microtesla. when a horizontal field of 18 microtesla is produced opposite to the earth's field by placing a current carrying wire , the new time period of the magnetic field will be?.
A vibration magnetometer placed in a magnetic meridian has a small bar magnet. the magnet execute oscillation with the time period of 2 second in Earth's horizontal magnetic field of 24 microtesla. when a horizontal field of 18 microtesla is produced opposite to the earth's field by placing a current carrying wire , the new time period of the magnetic field will be? for NEET 2024 is part of NEET preparation. The Question and answers have been prepared according to the NEET exam syllabus. Information about A vibration magnetometer placed in a magnetic meridian has a small bar magnet. the magnet execute oscillation with the time period of 2 second in Earth's horizontal magnetic field of 24 microtesla. when a horizontal field of 18 microtesla is produced opposite to the earth's field by placing a current carrying wire , the new time period of the magnetic field will be? covers all topics & solutions for NEET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A vibration magnetometer placed in a magnetic meridian has a small bar magnet. the magnet execute oscillation with the time period of 2 second in Earth's horizontal magnetic field of 24 microtesla. when a horizontal field of 18 microtesla is produced opposite to the earth's field by placing a current carrying wire , the new time period of the magnetic field will be?.
Solutions for A vibration magnetometer placed in a magnetic meridian has a small bar magnet. the magnet execute oscillation with the time period of 2 second in Earth's horizontal magnetic field of 24 microtesla. when a horizontal field of 18 microtesla is produced opposite to the earth's field by placing a current carrying wire , the new time period of the magnetic field will be? in English & in Hindi are available as part of our courses for NEET.
Download more important topics, notes, lectures and mock test series for NEET Exam by signing up for free.
Here you can find the meaning of A vibration magnetometer placed in a magnetic meridian has a small bar magnet. the magnet execute oscillation with the time period of 2 second in Earth's horizontal magnetic field of 24 microtesla. when a horizontal field of 18 microtesla is produced opposite to the earth's field by placing a current carrying wire , the new time period of the magnetic field will be? defined & explained in the simplest way possible. Besides giving the explanation of
A vibration magnetometer placed in a magnetic meridian has a small bar magnet. the magnet execute oscillation with the time period of 2 second in Earth's horizontal magnetic field of 24 microtesla. when a horizontal field of 18 microtesla is produced opposite to the earth's field by placing a current carrying wire , the new time period of the magnetic field will be?, a detailed solution for A vibration magnetometer placed in a magnetic meridian has a small bar magnet. the magnet execute oscillation with the time period of 2 second in Earth's horizontal magnetic field of 24 microtesla. when a horizontal field of 18 microtesla is produced opposite to the earth's field by placing a current carrying wire , the new time period of the magnetic field will be? has been provided alongside types of A vibration magnetometer placed in a magnetic meridian has a small bar magnet. the magnet execute oscillation with the time period of 2 second in Earth's horizontal magnetic field of 24 microtesla. when a horizontal field of 18 microtesla is produced opposite to the earth's field by placing a current carrying wire , the new time period of the magnetic field will be? theory, EduRev gives you an
ample number of questions to practice A vibration magnetometer placed in a magnetic meridian has a small bar magnet. the magnet execute oscillation with the time period of 2 second in Earth's horizontal magnetic field of 24 microtesla. when a horizontal field of 18 microtesla is produced opposite to the earth's field by placing a current carrying wire , the new time period of the magnetic field will be? tests, examples and also practice NEET tests.

|
Explore Courses for NEET exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.

























