NEET Exam > NEET Questions > A long straight wire carries a electric curre...
Start Learning for Free
A long straight wire carries a electric current of 2 ampere. the magnetic induction at the perpendicular distance of 5m is from the wire is?
Verified Answer
A long straight wire carries a electric current of 2 ampere. the magne...
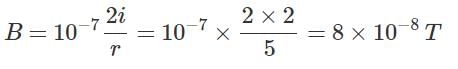
 This question is part of UPSC exam. View all NEET courses
This question is part of UPSC exam. View all NEET courses
Most Upvoted Answer
A long straight wire carries a electric current of 2 ampere. the magne...
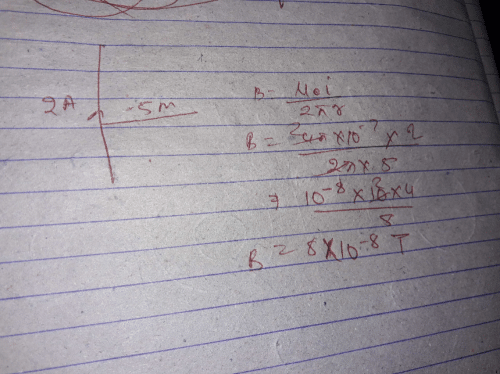
Community Answer
A long straight wire carries a electric current of 2 ampere. the magne...
Magnetic Induction due to a Current-carrying Wire
To determine the magnetic induction or magnetic field strength at a perpendicular distance from a long straight wire carrying an electric current, we can use the formula given by Ampere's law. Ampere's law states that the magnetic field strength around a closed loop is directly proportional to the current passing through the loop.
The Formula
The formula to calculate the magnetic field strength (B) at a perpendicular distance (r) from a long straight wire carrying a current (I) is given by:
B = μ₀ * (I / 2πr)
Where:
B = magnetic field strength (in Tesla)
μ₀ = permeability of free space (4π × 10⁻⁷ T·m/A)
I = electric current (in Amperes)
r = perpendicular distance from the wire (in meters)
π = mathematical constant pi (approximately 3.14159)
Given Values
In this case, the electric current passing through the wire is 2 Amperes, and the perpendicular distance from the wire is 5 meters. We can substitute these values into the formula to calculate the magnetic induction.
Calculating the Magnetic Induction
Using the formula mentioned earlier:
B = (4π × 10⁻⁷ T·m/A) * (2 A / 2π * 5 m)
B = (4π × 10⁻⁷ T·m/A) * (1 A / 5 m)
B = 8π × 10⁻⁸ T/m
The Result
Therefore, the magnetic induction or magnetic field strength at a perpendicular distance of 5 meters from the wire carrying a current of 2 Amperes is approximately 8π × 10⁻⁸ Tesla per meter.
Explanation
- The formula used to calculate the magnetic induction is derived from Ampere's law, which relates the magnetic field strength to the current passing through a closed loop.
- The magnetic field strength decreases as the distance from the wire increases. This inverse relationship is due to the spreading out of the magnetic field lines as they move away from the wire.
- The value of μ₀ represents the permeability of free space, which is a constant value in physics.
- The formula also includes a factor of 2πr in the denominator to account for the circular path around the wire where the magnetic field is being measured.
To determine the magnetic induction or magnetic field strength at a perpendicular distance from a long straight wire carrying an electric current, we can use the formula given by Ampere's law. Ampere's law states that the magnetic field strength around a closed loop is directly proportional to the current passing through the loop.
The Formula
The formula to calculate the magnetic field strength (B) at a perpendicular distance (r) from a long straight wire carrying a current (I) is given by:
B = μ₀ * (I / 2πr)
Where:
B = magnetic field strength (in Tesla)
μ₀ = permeability of free space (4π × 10⁻⁷ T·m/A)
I = electric current (in Amperes)
r = perpendicular distance from the wire (in meters)
π = mathematical constant pi (approximately 3.14159)
Given Values
In this case, the electric current passing through the wire is 2 Amperes, and the perpendicular distance from the wire is 5 meters. We can substitute these values into the formula to calculate the magnetic induction.
Calculating the Magnetic Induction
Using the formula mentioned earlier:
B = (4π × 10⁻⁷ T·m/A) * (2 A / 2π * 5 m)
B = (4π × 10⁻⁷ T·m/A) * (1 A / 5 m)
B = 8π × 10⁻⁸ T/m
The Result
Therefore, the magnetic induction or magnetic field strength at a perpendicular distance of 5 meters from the wire carrying a current of 2 Amperes is approximately 8π × 10⁻⁸ Tesla per meter.
Explanation
- The formula used to calculate the magnetic induction is derived from Ampere's law, which relates the magnetic field strength to the current passing through a closed loop.
- The magnetic field strength decreases as the distance from the wire increases. This inverse relationship is due to the spreading out of the magnetic field lines as they move away from the wire.
- The value of μ₀ represents the permeability of free space, which is a constant value in physics.
- The formula also includes a factor of 2πr in the denominator to account for the circular path around the wire where the magnetic field is being measured.
Attention NEET Students!
To make sure you are not studying endlessly, EduRev has designed NEET study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in NEET.

|
Explore Courses for NEET exam
|

|
Similar NEET Doubts
A long straight wire carries a electric current of 2 ampere. the magnetic induction at the perpendicular distance of 5m is from the wire is?
Question Description
A long straight wire carries a electric current of 2 ampere. the magnetic induction at the perpendicular distance of 5m is from the wire is? for NEET 2024 is part of NEET preparation. The Question and answers have been prepared according to the NEET exam syllabus. Information about A long straight wire carries a electric current of 2 ampere. the magnetic induction at the perpendicular distance of 5m is from the wire is? covers all topics & solutions for NEET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A long straight wire carries a electric current of 2 ampere. the magnetic induction at the perpendicular distance of 5m is from the wire is?.
A long straight wire carries a electric current of 2 ampere. the magnetic induction at the perpendicular distance of 5m is from the wire is? for NEET 2024 is part of NEET preparation. The Question and answers have been prepared according to the NEET exam syllabus. Information about A long straight wire carries a electric current of 2 ampere. the magnetic induction at the perpendicular distance of 5m is from the wire is? covers all topics & solutions for NEET 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A long straight wire carries a electric current of 2 ampere. the magnetic induction at the perpendicular distance of 5m is from the wire is?.
Solutions for A long straight wire carries a electric current of 2 ampere. the magnetic induction at the perpendicular distance of 5m is from the wire is? in English & in Hindi are available as part of our courses for NEET.
Download more important topics, notes, lectures and mock test series for NEET Exam by signing up for free.
Here you can find the meaning of A long straight wire carries a electric current of 2 ampere. the magnetic induction at the perpendicular distance of 5m is from the wire is? defined & explained in the simplest way possible. Besides giving the explanation of
A long straight wire carries a electric current of 2 ampere. the magnetic induction at the perpendicular distance of 5m is from the wire is?, a detailed solution for A long straight wire carries a electric current of 2 ampere. the magnetic induction at the perpendicular distance of 5m is from the wire is? has been provided alongside types of A long straight wire carries a electric current of 2 ampere. the magnetic induction at the perpendicular distance of 5m is from the wire is? theory, EduRev gives you an
ample number of questions to practice A long straight wire carries a electric current of 2 ampere. the magnetic induction at the perpendicular distance of 5m is from the wire is? tests, examples and also practice NEET tests.

|
Explore Courses for NEET exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.




















