GATE Exam > GATE Questions > The ground state magnetic moment of 33S16is__...
Start Learning for Free
The ground state magnetic moment of 33S16 is_____________μN.(Correct upto two decimal place).
Correct answer is '1.15'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
The ground state magnetic moment of 33S16is_____________μN.(Correct...
The nucleus 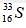 has 16 protons and 17 neutrons.
has 16 protons and 17 neutrons.
The shell structure of neutrons:
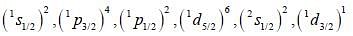
Since, last neutron lies in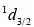 shell.
shell.
Therefore, the nuclear spin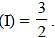
The magnetic moment of last neutron in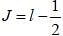 state is given by
state is given by
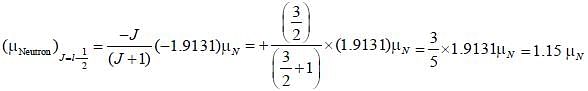
 has 16 protons and 17 neutrons.
has 16 protons and 17 neutrons.The shell structure of neutrons:
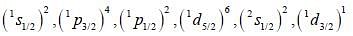
Since, last neutron lies in
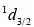 shell.
shell.Therefore, the nuclear spin
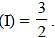
The magnetic moment of last neutron in
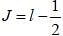 state is given by
state is given by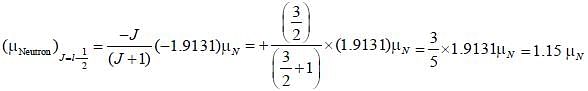
Most Upvoted Answer
The ground state magnetic moment of 33S16is_____________μN.(Correct...
The ground state of sulfur-33 (33S16) has an atomic number of 16, which means it has 16 protons. The ground state electron configuration of sulfur is 1s22s22p63s23p4.
To determine the ground state magnetic moment, we need to consider the spin and orbital angular momentum of the electrons.
In the ground state electron configuration, the 1s and 2s orbitals are fully filled with two electrons each, and the 2p orbital is half-filled with only one electron. Therefore, the total spin angular momentum is given by the spin of the 2p electron:
s = 1/2
The total orbital angular momentum is given by the sum of the individual orbital angular momenta of the electrons in the 3s and 3p orbitals:
l = 0 (for the 3s orbital) + 1 (for each of the three 3p orbitals) = 3
The total angular momentum (J) is given by the vector sum of the spin and orbital angular momenta:
J = |l - s| to |l + s|
J = |3 - 1/2| to |3 + 1/2|
J = 5/2 to 7/2
The ground state magnetic moment (μ) is given by the formula:
μ = gJμB
where g is the Landé g-factor, J is the total angular momentum, and μB is the Bohr magneton.
The Landé g-factor for sulfur is approximately 2.
Using the minimum value of J (5/2), we can calculate the minimum ground state magnetic moment:
μmin = (2)(5/2)(μB)
μmin ≈ 5(μB)
Therefore, the ground state magnetic moment of 33S16 is approximately 5 Bohr magnetons (μB).
To determine the ground state magnetic moment, we need to consider the spin and orbital angular momentum of the electrons.
In the ground state electron configuration, the 1s and 2s orbitals are fully filled with two electrons each, and the 2p orbital is half-filled with only one electron. Therefore, the total spin angular momentum is given by the spin of the 2p electron:
s = 1/2
The total orbital angular momentum is given by the sum of the individual orbital angular momenta of the electrons in the 3s and 3p orbitals:
l = 0 (for the 3s orbital) + 1 (for each of the three 3p orbitals) = 3
The total angular momentum (J) is given by the vector sum of the spin and orbital angular momenta:
J = |l - s| to |l + s|
J = |3 - 1/2| to |3 + 1/2|
J = 5/2 to 7/2
The ground state magnetic moment (μ) is given by the formula:
μ = gJμB
where g is the Landé g-factor, J is the total angular momentum, and μB is the Bohr magneton.
The Landé g-factor for sulfur is approximately 2.
Using the minimum value of J (5/2), we can calculate the minimum ground state magnetic moment:
μmin = (2)(5/2)(μB)
μmin ≈ 5(μB)
Therefore, the ground state magnetic moment of 33S16 is approximately 5 Bohr magnetons (μB).

|
Explore Courses for GATE exam
|

|
Similar GATE Doubts
The ground state magnetic moment of 33S16is_____________μN.(Correct upto two decimal place).Correct answer is '1.15'. Can you explain this answer?
Question Description
The ground state magnetic moment of 33S16is_____________μN.(Correct upto two decimal place).Correct answer is '1.15'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about The ground state magnetic moment of 33S16is_____________μN.(Correct upto two decimal place).Correct answer is '1.15'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The ground state magnetic moment of 33S16is_____________μN.(Correct upto two decimal place).Correct answer is '1.15'. Can you explain this answer?.
The ground state magnetic moment of 33S16is_____________μN.(Correct upto two decimal place).Correct answer is '1.15'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about The ground state magnetic moment of 33S16is_____________μN.(Correct upto two decimal place).Correct answer is '1.15'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The ground state magnetic moment of 33S16is_____________μN.(Correct upto two decimal place).Correct answer is '1.15'. Can you explain this answer?.
Solutions for The ground state magnetic moment of 33S16is_____________μN.(Correct upto two decimal place).Correct answer is '1.15'. Can you explain this answer? in English & in Hindi are available as part of our courses for GATE.
Download more important topics, notes, lectures and mock test series for GATE Exam by signing up for free.
Here you can find the meaning of The ground state magnetic moment of 33S16is_____________μN.(Correct upto two decimal place).Correct answer is '1.15'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The ground state magnetic moment of 33S16is_____________μN.(Correct upto two decimal place).Correct answer is '1.15'. Can you explain this answer?, a detailed solution for The ground state magnetic moment of 33S16is_____________μN.(Correct upto two decimal place).Correct answer is '1.15'. Can you explain this answer? has been provided alongside types of The ground state magnetic moment of 33S16is_____________μN.(Correct upto two decimal place).Correct answer is '1.15'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The ground state magnetic moment of 33S16is_____________μN.(Correct upto two decimal place).Correct answer is '1.15'. Can you explain this answer? tests, examples and also practice GATE tests.

|
Explore Courses for GATE exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















