GATE Exam > GATE Questions > A car is moving with constant linear accelera...
Start Learning for Free
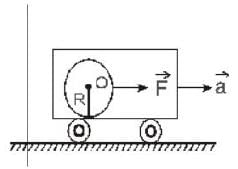
A car is moving with constant linear acceleration a along horizontal x-axis. A solid sphere of mass M and radius R is found rolling without slipping on the horizontal floor of the car in the same direction as seen from an inertial frame outside the car. The acceleration of the sphere in the inertial frame is ________ .
Correct answer is '0.71'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A car is moving with constant linear acceleration a along horizontal x...
The torque about the point O is,
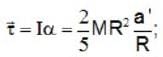where a’ is the acceleration of solid sphere during its motion,
we know that,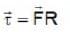
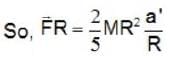
The net force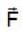
in the inertial frame is,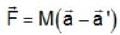 
Put the value of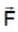
in equation (1), we get
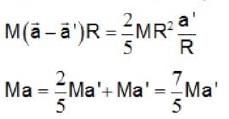
The acceleration of the sphere in the inertial frame is,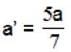
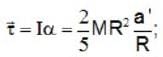where a’ is the acceleration of solid sphere during its motion, we know that,
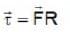
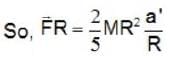
The net force
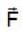in the inertial frame is,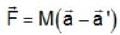 Put the value of
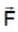in equation (1), we get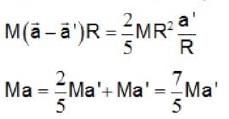The acceleration of the sphere in the inertial frame is,
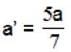
Most Upvoted Answer
A car is moving with constant linear acceleration a along horizontal x...
Acceleration of the Sphere in the Inertial Frame:
In order to find the acceleration of the sphere in the inertial frame, we need to consider the forces acting on the sphere and apply Newton's laws of motion.
1. Forces Acting on the Sphere:
a) Gravity:
The force of gravity acts vertically downward on the sphere with a magnitude of mg, where m is the mass of the sphere.
b) Normal Force:
The normal force acts perpendicular to the surface of the floor and prevents the sphere from sinking into the floor. It has an equal magnitude and opposite direction to the gravitational force, i.e., mg.
c) Friction:
Since the sphere is rolling without slipping, the static friction force acts between the sphere and the floor. It provides the necessary torque to cause the sphere to roll. The maximum static friction force can be calculated using the equation f_friction = μ_s * N, where μ_s is the coefficient of static friction and N is the normal force.
2. Applying Newton's Laws of Motion:
a) In the x-direction:
The net force acting on the sphere in the x-direction is the horizontal component of the static friction force. This force causes the sphere to accelerate in the x-direction.
b) In the y-direction:
The net force acting on the sphere in the y-direction is the difference between the gravitational force and the vertical component of the static friction force. Since the sphere is not accelerating vertically, this net force is zero.
3. Equations of Motion:
a) In the x-direction:
Using Newton's second law, we can write the equation as:
ma = f_friction
ma = μ_s * N
b) In the y-direction:
Since the net force in the y-direction is zero, we have:
mg - f_friction = 0
mg = μ_s * N
4. Solving for Acceleration:
Dividing the equation in the x-direction by the equation in the y-direction, we get:
a/g = μ_s * N / mg
a/g = μ_s
Given that the coefficient of static friction, μ_s, is 0.71, we can substitute it into the equation:
a/g = 0.71
Therefore, the acceleration of the sphere in the inertial frame is 0.71 times the acceleration due to gravity (g).
In order to find the acceleration of the sphere in the inertial frame, we need to consider the forces acting on the sphere and apply Newton's laws of motion.
1. Forces Acting on the Sphere:
a) Gravity:
The force of gravity acts vertically downward on the sphere with a magnitude of mg, where m is the mass of the sphere.
b) Normal Force:
The normal force acts perpendicular to the surface of the floor and prevents the sphere from sinking into the floor. It has an equal magnitude and opposite direction to the gravitational force, i.e., mg.
c) Friction:
Since the sphere is rolling without slipping, the static friction force acts between the sphere and the floor. It provides the necessary torque to cause the sphere to roll. The maximum static friction force can be calculated using the equation f_friction = μ_s * N, where μ_s is the coefficient of static friction and N is the normal force.
2. Applying Newton's Laws of Motion:
a) In the x-direction:
The net force acting on the sphere in the x-direction is the horizontal component of the static friction force. This force causes the sphere to accelerate in the x-direction.
b) In the y-direction:
The net force acting on the sphere in the y-direction is the difference between the gravitational force and the vertical component of the static friction force. Since the sphere is not accelerating vertically, this net force is zero.
3. Equations of Motion:
a) In the x-direction:
Using Newton's second law, we can write the equation as:
ma = f_friction
ma = μ_s * N
b) In the y-direction:
Since the net force in the y-direction is zero, we have:
mg - f_friction = 0
mg = μ_s * N
4. Solving for Acceleration:
Dividing the equation in the x-direction by the equation in the y-direction, we get:
a/g = μ_s * N / mg
a/g = μ_s
Given that the coefficient of static friction, μ_s, is 0.71, we can substitute it into the equation:
a/g = 0.71
Therefore, the acceleration of the sphere in the inertial frame is 0.71 times the acceleration due to gravity (g).
|
Explore Courses for GATE exam
|
|
Similar GATE Doubts
A car is moving with constant linear acceleration a along horizontal x-axis. A solid sphere of mass M and radius R is found rolling without slipping on the horizontal floor of the car in the same direction as seen from an inertial frame outside the car. The acceleration of the sphere in the inertial frame is ________ .Correct answer is '0.71'. Can you explain this answer?
Question Description
A car is moving with constant linear acceleration a along horizontal x-axis. A solid sphere of mass M and radius R is found rolling without slipping on the horizontal floor of the car in the same direction as seen from an inertial frame outside the car. The acceleration of the sphere in the inertial frame is ________ .Correct answer is '0.71'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about A car is moving with constant linear acceleration a along horizontal x-axis. A solid sphere of mass M and radius R is found rolling without slipping on the horizontal floor of the car in the same direction as seen from an inertial frame outside the car. The acceleration of the sphere in the inertial frame is ________ .Correct answer is '0.71'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A car is moving with constant linear acceleration a along horizontal x-axis. A solid sphere of mass M and radius R is found rolling without slipping on the horizontal floor of the car in the same direction as seen from an inertial frame outside the car. The acceleration of the sphere in the inertial frame is ________ .Correct answer is '0.71'. Can you explain this answer?.
A car is moving with constant linear acceleration a along horizontal x-axis. A solid sphere of mass M and radius R is found rolling without slipping on the horizontal floor of the car in the same direction as seen from an inertial frame outside the car. The acceleration of the sphere in the inertial frame is ________ .Correct answer is '0.71'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about A car is moving with constant linear acceleration a along horizontal x-axis. A solid sphere of mass M and radius R is found rolling without slipping on the horizontal floor of the car in the same direction as seen from an inertial frame outside the car. The acceleration of the sphere in the inertial frame is ________ .Correct answer is '0.71'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A car is moving with constant linear acceleration a along horizontal x-axis. A solid sphere of mass M and radius R is found rolling without slipping on the horizontal floor of the car in the same direction as seen from an inertial frame outside the car. The acceleration of the sphere in the inertial frame is ________ .Correct answer is '0.71'. Can you explain this answer?.
Solutions for A car is moving with constant linear acceleration a along horizontal x-axis. A solid sphere of mass M and radius R is found rolling without slipping on the horizontal floor of the car in the same direction as seen from an inertial frame outside the car. The acceleration of the sphere in the inertial frame is ________ .Correct answer is '0.71'. Can you explain this answer? in English & in Hindi are available as part of our courses for GATE.
Download more important topics, notes, lectures and mock test series for GATE Exam by signing up for free.
Here you can find the meaning of A car is moving with constant linear acceleration a along horizontal x-axis. A solid sphere of mass M and radius R is found rolling without slipping on the horizontal floor of the car in the same direction as seen from an inertial frame outside the car. The acceleration of the sphere in the inertial frame is ________ .Correct answer is '0.71'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A car is moving with constant linear acceleration a along horizontal x-axis. A solid sphere of mass M and radius R is found rolling without slipping on the horizontal floor of the car in the same direction as seen from an inertial frame outside the car. The acceleration of the sphere in the inertial frame is ________ .Correct answer is '0.71'. Can you explain this answer?, a detailed solution for A car is moving with constant linear acceleration a along horizontal x-axis. A solid sphere of mass M and radius R is found rolling without slipping on the horizontal floor of the car in the same direction as seen from an inertial frame outside the car. The acceleration of the sphere in the inertial frame is ________ .Correct answer is '0.71'. Can you explain this answer? has been provided alongside types of A car is moving with constant linear acceleration a along horizontal x-axis. A solid sphere of mass M and radius R is found rolling without slipping on the horizontal floor of the car in the same direction as seen from an inertial frame outside the car. The acceleration of the sphere in the inertial frame is ________ .Correct answer is '0.71'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A car is moving with constant linear acceleration a along horizontal x-axis. A solid sphere of mass M and radius R is found rolling without slipping on the horizontal floor of the car in the same direction as seen from an inertial frame outside the car. The acceleration of the sphere in the inertial frame is ________ .Correct answer is '0.71'. Can you explain this answer? tests, examples and also practice GATE tests.
|
|
Explore Courses for GATE exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup