GATE Exam > GATE Questions > A semiconductor sample at room temperature h...
Start Learning for Free
A semiconductor sample at room temperature has intrinsic concentration of 2.5 X 1017 /m3. After doping what will be the minority carrier concentration if the majority carrier concentration is given as 5.5 X 1021 /m3.
- a)1.136 X 1013 /m3
- b)2.2 X 104 /m3
- c)3.33 X 1010 /m3
- d)4.54 X 10-5 /m3
Correct answer is option 'A'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A semiconductor sample at room temperature has intrinsic concentratio...
In a pure Semiconductor (Intrinsic Semiconductor), the electron and hole concentrations are n1p1 respectively. By doping impurity atoms the SC becomes extrinsic then the electrons and hole concentrations n2p2 respectively, then the following equations are acceptable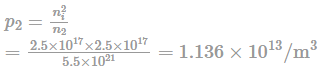
View all questions of this test
n1p1 = n2p2 = ni2For Intrinsic Semiconductor, n = p = ni2 and as per questions before doping n1p1 = ni2
Therefore,
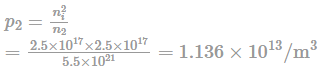
Most Upvoted Answer
A semiconductor sample at room temperature has intrinsic concentratio...
To determine the minority carrier concentration after doping a semiconductor sample, we need to understand the concept of doping and its impact on the carrier concentrations.
Doping is the process of intentionally adding impurities to a semiconductor material to alter its electrical properties. The impurities used for doping are typically atoms of different elements, such as Boron (B) or Phosphorous (P), which have either fewer or more valence electrons than the semiconductor material.
Intrinsic concentration (ni) is the carrier concentration in an undoped semiconductor material at a given temperature. It represents the equilibrium concentration of both the majority (holes in p-type material or electrons in n-type material) and minority carriers (electrons in p-type material or holes in n-type material).
Given data:
Intrinsic concentration (ni) = 2.5 x 10^17 /m^3 (equilibrium concentration of both majority and minority carriers)
Majority carrier concentration = 5.5 x 10^21 /m^3 (after doping)
To find the minority carrier concentration, we can use the equation:
np = ni^2 / n
Where np is the minority carrier concentration, ni is the intrinsic concentration, and n is the majority carrier concentration.
Calculation:
Substituting the given values into the equation:
np = (2.5 x 10^17 /m^3)^2 / (5.5 x 10^21 /m^3)
np = 6.25 x 10^34 /m^6 / 5.5 x 10^21 /m^3
np = 1.136 x 10^13 /m^3
Therefore, the minority carrier concentration after doping is 1.136 x 10^13 /m^3.
Hence, the correct answer is option 'A': 1.136 x 10^13 /m^3.
Doping is the process of intentionally adding impurities to a semiconductor material to alter its electrical properties. The impurities used for doping are typically atoms of different elements, such as Boron (B) or Phosphorous (P), which have either fewer or more valence electrons than the semiconductor material.
Intrinsic concentration (ni) is the carrier concentration in an undoped semiconductor material at a given temperature. It represents the equilibrium concentration of both the majority (holes in p-type material or electrons in n-type material) and minority carriers (electrons in p-type material or holes in n-type material).
Given data:
Intrinsic concentration (ni) = 2.5 x 10^17 /m^3 (equilibrium concentration of both majority and minority carriers)
Majority carrier concentration = 5.5 x 10^21 /m^3 (after doping)
To find the minority carrier concentration, we can use the equation:
np = ni^2 / n
Where np is the minority carrier concentration, ni is the intrinsic concentration, and n is the majority carrier concentration.
Calculation:
Substituting the given values into the equation:
np = (2.5 x 10^17 /m^3)^2 / (5.5 x 10^21 /m^3)
np = 6.25 x 10^34 /m^6 / 5.5 x 10^21 /m^3
np = 1.136 x 10^13 /m^3
Therefore, the minority carrier concentration after doping is 1.136 x 10^13 /m^3.
Hence, the correct answer is option 'A': 1.136 x 10^13 /m^3.

|
Explore Courses for GATE exam
|

|
Similar GATE Doubts
A semiconductor sample at room temperature has intrinsic concentration of 2.5 X 1017 /m3. After doping what will be the minority carrier concentration if the majority carrier concentration is given as 5.5 X 1021 /m3.a)1.136 X 1013 /m3b)2.2 X 104 /m3c)3.33 X 1010 /m3d)4.54 X 10-5 /m3Correct answer is option 'A'. Can you explain this answer?
Question Description
A semiconductor sample at room temperature has intrinsic concentration of 2.5 X 1017 /m3. After doping what will be the minority carrier concentration if the majority carrier concentration is given as 5.5 X 1021 /m3.a)1.136 X 1013 /m3b)2.2 X 104 /m3c)3.33 X 1010 /m3d)4.54 X 10-5 /m3Correct answer is option 'A'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about A semiconductor sample at room temperature has intrinsic concentration of 2.5 X 1017 /m3. After doping what will be the minority carrier concentration if the majority carrier concentration is given as 5.5 X 1021 /m3.a)1.136 X 1013 /m3b)2.2 X 104 /m3c)3.33 X 1010 /m3d)4.54 X 10-5 /m3Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A semiconductor sample at room temperature has intrinsic concentration of 2.5 X 1017 /m3. After doping what will be the minority carrier concentration if the majority carrier concentration is given as 5.5 X 1021 /m3.a)1.136 X 1013 /m3b)2.2 X 104 /m3c)3.33 X 1010 /m3d)4.54 X 10-5 /m3Correct answer is option 'A'. Can you explain this answer?.
A semiconductor sample at room temperature has intrinsic concentration of 2.5 X 1017 /m3. After doping what will be the minority carrier concentration if the majority carrier concentration is given as 5.5 X 1021 /m3.a)1.136 X 1013 /m3b)2.2 X 104 /m3c)3.33 X 1010 /m3d)4.54 X 10-5 /m3Correct answer is option 'A'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about A semiconductor sample at room temperature has intrinsic concentration of 2.5 X 1017 /m3. After doping what will be the minority carrier concentration if the majority carrier concentration is given as 5.5 X 1021 /m3.a)1.136 X 1013 /m3b)2.2 X 104 /m3c)3.33 X 1010 /m3d)4.54 X 10-5 /m3Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A semiconductor sample at room temperature has intrinsic concentration of 2.5 X 1017 /m3. After doping what will be the minority carrier concentration if the majority carrier concentration is given as 5.5 X 1021 /m3.a)1.136 X 1013 /m3b)2.2 X 104 /m3c)3.33 X 1010 /m3d)4.54 X 10-5 /m3Correct answer is option 'A'. Can you explain this answer?.
Solutions for A semiconductor sample at room temperature has intrinsic concentration of 2.5 X 1017 /m3. After doping what will be the minority carrier concentration if the majority carrier concentration is given as 5.5 X 1021 /m3.a)1.136 X 1013 /m3b)2.2 X 104 /m3c)3.33 X 1010 /m3d)4.54 X 10-5 /m3Correct answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for GATE.
Download more important topics, notes, lectures and mock test series for GATE Exam by signing up for free.
Here you can find the meaning of A semiconductor sample at room temperature has intrinsic concentration of 2.5 X 1017 /m3. After doping what will be the minority carrier concentration if the majority carrier concentration is given as 5.5 X 1021 /m3.a)1.136 X 1013 /m3b)2.2 X 104 /m3c)3.33 X 1010 /m3d)4.54 X 10-5 /m3Correct answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A semiconductor sample at room temperature has intrinsic concentration of 2.5 X 1017 /m3. After doping what will be the minority carrier concentration if the majority carrier concentration is given as 5.5 X 1021 /m3.a)1.136 X 1013 /m3b)2.2 X 104 /m3c)3.33 X 1010 /m3d)4.54 X 10-5 /m3Correct answer is option 'A'. Can you explain this answer?, a detailed solution for A semiconductor sample at room temperature has intrinsic concentration of 2.5 X 1017 /m3. After doping what will be the minority carrier concentration if the majority carrier concentration is given as 5.5 X 1021 /m3.a)1.136 X 1013 /m3b)2.2 X 104 /m3c)3.33 X 1010 /m3d)4.54 X 10-5 /m3Correct answer is option 'A'. Can you explain this answer? has been provided alongside types of A semiconductor sample at room temperature has intrinsic concentration of 2.5 X 1017 /m3. After doping what will be the minority carrier concentration if the majority carrier concentration is given as 5.5 X 1021 /m3.a)1.136 X 1013 /m3b)2.2 X 104 /m3c)3.33 X 1010 /m3d)4.54 X 10-5 /m3Correct answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A semiconductor sample at room temperature has intrinsic concentration of 2.5 X 1017 /m3. After doping what will be the minority carrier concentration if the majority carrier concentration is given as 5.5 X 1021 /m3.a)1.136 X 1013 /m3b)2.2 X 104 /m3c)3.33 X 1010 /m3d)4.54 X 10-5 /m3Correct answer is option 'A'. Can you explain this answer? tests, examples and also practice GATE tests.

|
Explore Courses for GATE exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















