GATE Exam > GATE Questions > A p - n junction is formed from germanium of ...
Start Learning for Free
A p - n junction is formed from germanium of conductivity 0.8 ohm-1 cm-1on p- side & 1.6 ohm-1 cm-1 on n-side. Calculate potential barrier at 300K (in V). (ni = 2.1 x 1013 cm-1) (Given μp = 2000 cm2/volt-sec and μn = 4000 cm2/volt-sec) (K = 1.38 x 10-16 erg per °K)
Correct answer is '0.2467'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A p - n junction is formed from germanium of conductivity 0.8 ohm-1 cm...
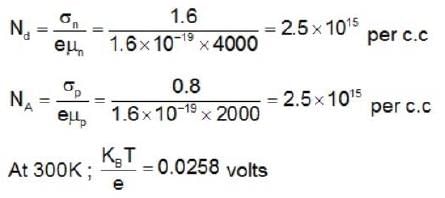
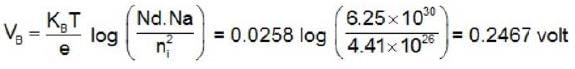
Most Upvoted Answer
A p - n junction is formed from germanium of conductivity 0.8 ohm-1 cm...
And 1.2 ohm-1 cm-1 on n-side. The junction has an area of 0.5 cm2 and the potential barrier is 0.3 V. Determine the current flowing through the junction when a forward bias of 0.4 V is applied.
To determine the current flowing through the junction, we can use the diode equation:
I = Is * (e^(Vd/Vt) - 1)
Where:
I is the current flowing through the junction
Is is the reverse saturation current
Vd is the voltage across the junction
Vt is the thermal voltage, which is approximately 0.026 V at room temperature
First, we need to calculate the reverse saturation current (Is) using the area and conductivity of the junction.
Is = (qnDpAp + qnDnAn) / 2
Where:
q is the charge of an electron (1.6 * 10^-19 C)
Dp and Dn are the diffusion coefficients of holes and electrons, respectively
Ap and An are the cross-sectional areas of the p-side and n-side, respectively
Assuming the diffusion coefficients are equal, and using the given values:
Dp = Dn = 1 cm2/s
Ap = An = 0.5 cm2
Is = (1.6 * 10^-19 C * 1 cm2/s * 0.8 ohm-1 cm-1 * 0.5 cm2 + 1.6 * 10^-19 C * 1 cm2/s * 1.2 ohm-1 cm-1 * 0.5 cm2) / 2
= (0.4 * 10^-19 C + 0.6 * 10^-19 C) / 2
= 0.5 * 10^-19 C
Next, we can calculate the current (I) using the forward bias voltage (Vd = 0.4 V) and the thermal voltage (Vt = 0.026 V).
I = (0.5 * 10^-19 C) * (e^(0.4 V/0.026 V) - 1)
≈ (0.5 * 10^-19 C) * (e^15.385 - 1)
≈ (0.5 * 10^-19 C) * (4.8 * 10^6 - 1)
≈ (0.5 * 10^-19 C) * (4.8 * 10^6)
≈ 2.4 * 10^-13 A
Therefore, the current flowing through the junction when a forward bias of 0.4 V is applied is approximately 2.4 * 10^-13 A.
To determine the current flowing through the junction, we can use the diode equation:
I = Is * (e^(Vd/Vt) - 1)
Where:
I is the current flowing through the junction
Is is the reverse saturation current
Vd is the voltage across the junction
Vt is the thermal voltage, which is approximately 0.026 V at room temperature
First, we need to calculate the reverse saturation current (Is) using the area and conductivity of the junction.
Is = (qnDpAp + qnDnAn) / 2
Where:
q is the charge of an electron (1.6 * 10^-19 C)
Dp and Dn are the diffusion coefficients of holes and electrons, respectively
Ap and An are the cross-sectional areas of the p-side and n-side, respectively
Assuming the diffusion coefficients are equal, and using the given values:
Dp = Dn = 1 cm2/s
Ap = An = 0.5 cm2
Is = (1.6 * 10^-19 C * 1 cm2/s * 0.8 ohm-1 cm-1 * 0.5 cm2 + 1.6 * 10^-19 C * 1 cm2/s * 1.2 ohm-1 cm-1 * 0.5 cm2) / 2
= (0.4 * 10^-19 C + 0.6 * 10^-19 C) / 2
= 0.5 * 10^-19 C
Next, we can calculate the current (I) using the forward bias voltage (Vd = 0.4 V) and the thermal voltage (Vt = 0.026 V).
I = (0.5 * 10^-19 C) * (e^(0.4 V/0.026 V) - 1)
≈ (0.5 * 10^-19 C) * (e^15.385 - 1)
≈ (0.5 * 10^-19 C) * (4.8 * 10^6 - 1)
≈ (0.5 * 10^-19 C) * (4.8 * 10^6)
≈ 2.4 * 10^-13 A
Therefore, the current flowing through the junction when a forward bias of 0.4 V is applied is approximately 2.4 * 10^-13 A.

|
Explore Courses for GATE exam
|

|
Similar GATE Doubts
A p - n junction is formed from germanium of conductivity 0.8 ohm-1 cm-1on p- side & 1.6 ohm-1 cm-1on n-side. Calculate potential barrier at 300K (in V). (ni= 2.1 x 1013 cm-1) (Given μp = 2000 cm2/volt-sec and μn = 4000 cm2/volt-sec) (K = 1.38 x 10-16 erg per °K)Correct answer is '0.2467'. Can you explain this answer?
Question Description
A p - n junction is formed from germanium of conductivity 0.8 ohm-1 cm-1on p- side & 1.6 ohm-1 cm-1on n-side. Calculate potential barrier at 300K (in V). (ni= 2.1 x 1013 cm-1) (Given μp = 2000 cm2/volt-sec and μn = 4000 cm2/volt-sec) (K = 1.38 x 10-16 erg per °K)Correct answer is '0.2467'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about A p - n junction is formed from germanium of conductivity 0.8 ohm-1 cm-1on p- side & 1.6 ohm-1 cm-1on n-side. Calculate potential barrier at 300K (in V). (ni= 2.1 x 1013 cm-1) (Given μp = 2000 cm2/volt-sec and μn = 4000 cm2/volt-sec) (K = 1.38 x 10-16 erg per °K)Correct answer is '0.2467'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A p - n junction is formed from germanium of conductivity 0.8 ohm-1 cm-1on p- side & 1.6 ohm-1 cm-1on n-side. Calculate potential barrier at 300K (in V). (ni= 2.1 x 1013 cm-1) (Given μp = 2000 cm2/volt-sec and μn = 4000 cm2/volt-sec) (K = 1.38 x 10-16 erg per °K)Correct answer is '0.2467'. Can you explain this answer?.
A p - n junction is formed from germanium of conductivity 0.8 ohm-1 cm-1on p- side & 1.6 ohm-1 cm-1on n-side. Calculate potential barrier at 300K (in V). (ni= 2.1 x 1013 cm-1) (Given μp = 2000 cm2/volt-sec and μn = 4000 cm2/volt-sec) (K = 1.38 x 10-16 erg per °K)Correct answer is '0.2467'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about A p - n junction is formed from germanium of conductivity 0.8 ohm-1 cm-1on p- side & 1.6 ohm-1 cm-1on n-side. Calculate potential barrier at 300K (in V). (ni= 2.1 x 1013 cm-1) (Given μp = 2000 cm2/volt-sec and μn = 4000 cm2/volt-sec) (K = 1.38 x 10-16 erg per °K)Correct answer is '0.2467'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A p - n junction is formed from germanium of conductivity 0.8 ohm-1 cm-1on p- side & 1.6 ohm-1 cm-1on n-side. Calculate potential barrier at 300K (in V). (ni= 2.1 x 1013 cm-1) (Given μp = 2000 cm2/volt-sec and μn = 4000 cm2/volt-sec) (K = 1.38 x 10-16 erg per °K)Correct answer is '0.2467'. Can you explain this answer?.
Solutions for A p - n junction is formed from germanium of conductivity 0.8 ohm-1 cm-1on p- side & 1.6 ohm-1 cm-1on n-side. Calculate potential barrier at 300K (in V). (ni= 2.1 x 1013 cm-1) (Given μp = 2000 cm2/volt-sec and μn = 4000 cm2/volt-sec) (K = 1.38 x 10-16 erg per °K)Correct answer is '0.2467'. Can you explain this answer? in English & in Hindi are available as part of our courses for GATE.
Download more important topics, notes, lectures and mock test series for GATE Exam by signing up for free.
Here you can find the meaning of A p - n junction is formed from germanium of conductivity 0.8 ohm-1 cm-1on p- side & 1.6 ohm-1 cm-1on n-side. Calculate potential barrier at 300K (in V). (ni= 2.1 x 1013 cm-1) (Given μp = 2000 cm2/volt-sec and μn = 4000 cm2/volt-sec) (K = 1.38 x 10-16 erg per °K)Correct answer is '0.2467'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A p - n junction is formed from germanium of conductivity 0.8 ohm-1 cm-1on p- side & 1.6 ohm-1 cm-1on n-side. Calculate potential barrier at 300K (in V). (ni= 2.1 x 1013 cm-1) (Given μp = 2000 cm2/volt-sec and μn = 4000 cm2/volt-sec) (K = 1.38 x 10-16 erg per °K)Correct answer is '0.2467'. Can you explain this answer?, a detailed solution for A p - n junction is formed from germanium of conductivity 0.8 ohm-1 cm-1on p- side & 1.6 ohm-1 cm-1on n-side. Calculate potential barrier at 300K (in V). (ni= 2.1 x 1013 cm-1) (Given μp = 2000 cm2/volt-sec and μn = 4000 cm2/volt-sec) (K = 1.38 x 10-16 erg per °K)Correct answer is '0.2467'. Can you explain this answer? has been provided alongside types of A p - n junction is formed from germanium of conductivity 0.8 ohm-1 cm-1on p- side & 1.6 ohm-1 cm-1on n-side. Calculate potential barrier at 300K (in V). (ni= 2.1 x 1013 cm-1) (Given μp = 2000 cm2/volt-sec and μn = 4000 cm2/volt-sec) (K = 1.38 x 10-16 erg per °K)Correct answer is '0.2467'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A p - n junction is formed from germanium of conductivity 0.8 ohm-1 cm-1on p- side & 1.6 ohm-1 cm-1on n-side. Calculate potential barrier at 300K (in V). (ni= 2.1 x 1013 cm-1) (Given μp = 2000 cm2/volt-sec and μn = 4000 cm2/volt-sec) (K = 1.38 x 10-16 erg per °K)Correct answer is '0.2467'. Can you explain this answer? tests, examples and also practice GATE tests.

|
Explore Courses for GATE exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















